Skip to main content
SpringerLink
Log in

Weil–Petersson Teichmüller space III: dependence of Riemann mappings for Weil–Petersson curves

  • Published:
Mathematische Annalen Aims and scope Submit manuscript

Abstract

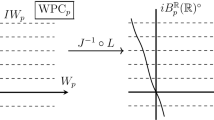
The classical Riemann mapping theorem implies that there exists a so-called Riemann mapping which takes the upper half plane onto the left domain bounded by a Jordan curve in the extended complex plane. The primary purpose of the paper is to study the basic problem: how does a Riemann mapping depend on the corresponding Jordan curve? We are mainly concerned with those Jordan curves in the Weil–Petersson class, namely, the corresponding Riemann mappings can be quasiconformally extended to the whole plane with Beltrami coefficients being square integrable under the Poincaré metric. After giving a geometric characterization of a Weil–Petersson curve, we endow the space of all normalized Weil–Petersson curves with a new real Hilbert manifold structure in a geometric manner and show that this new structure is topologically equivalent to the standard complex Hilbert manifold structure, which implies that an appropriately chosen Riemann mapping depends continuously on a Weil–Petersson curve (and vice versa). This can be considered as the first result about the continuous dependence of Riemann mappings on non-smooth Jordan curves.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

Notes

  1. We conjecture that in general neither \(\log f'\) nor \(\log (h^{-1})'\) depends continuously on \(\Gamma \) (or b).

  2. After an earlier version of this manuscript was posted on arXiv [46], we learned from Tim Mesikepp that Bishop [6] obtained various geometric characterizations of a bounded Weil-Petersson curve. The authors would like to thank Tim Mesikepp for calling this reference to their attention.

References

  1. Ahlfors, L.V.: Lectures on quasiconformal mapping. Van Nostrand, New York City (1966)

    MATH  Google Scholar 

  2. Astala, K., Gehring, F.W.: Injectivity, the BMO norm and the universal Teichmüller space. J. Anal. Math. 46, 16–57 (1986)

    MATH  Google Scholar 

  3. Astala, K., González, M.J.: Chord-arc curves and the Beurling transform. Invent. Math. 205, 57–81 (2016)

    MathSciNet  MATH  Google Scholar 

  4. Astala, K., Zinsmeister, M.: Teichmüller spaces and BMOA. Math. Ann. 289, 613–625 (1991)

    MathSciNet  MATH  Google Scholar 

  5. Beurling, A., Ahlfors, L.V.: The boundary correspondence under quasiconformal mappings. Acta Math. 96, 125–142 (1956)

    MathSciNet  MATH  Google Scholar 

  6. Bishop, Ch.: Curves of finite total curvature and the Weil–Petersson class. preprint (2019)

  7. Bowick, M.J., Rajeev, S.G.: String theory as the Kähler geometry of loop space. Phys. Rev. Lett. 58, 535–538 (1987)

    MathSciNet  Google Scholar 

  8. Bowick, M.J., Rajeev, S.G.: The holomorphic geometry of closed bosnic string theory and \(\text{ Diff }\, S^1/S^1\). Nuclear Phys. B 293, 348–384 (1987)

    MathSciNet  Google Scholar 

  9. Coifman, R.R., Fefferman, C.: Weighted norm inequalities for maximal functions and singular integrals. Studia Math. 51, 241–250 (1974)

    MathSciNet  MATH  Google Scholar 

  10. Coifman, R.R., Meyer, Y.: Lavrentiev’s curves and conformal mappings. Institute Mittag–Leffler, Report No.5 (1983)

  11. Cui, G.: Integrably asymptotic affine homeomorphisms of the circle and Teichmüller spaces. Sci. China Ser. A. 43, 267–279 (2000)

    MathSciNet  MATH  Google Scholar 

  12. David, G.: Thése de troisiéme cycle. Université de Paris XI, 91405 Orsay, France

  13. Fefferman, C., Stein, E.: \(H^p\) spaces of several variables. Acta Math. 129, 137–193 (1972)

    MathSciNet  MATH  Google Scholar 

  14. Feiszli, M., Narayan, A.: Numerical computation of Weil–Peterson geodesics in the universal Teichmüller space. SIAM J. Imaging Sci. 10, 1322–1345 (2017)

    MathSciNet  MATH  Google Scholar 

  15. Figalli, A.: On flows of \(H^{\frac{3}{2}}\)-vector fields on the circle. Math. Ann. 347, 43–57 (2010)

    MathSciNet  MATH  Google Scholar 

  16. Gallardo-Gutiérrez, E.A., González, M.J., Pérez-González, F., Pommerenke, Ch., Rättyä, J.: Locally univalent functions, VMOA and the Dirichlet space. Proc. Lond. Math. Soc. 106, 565–588 (2013)

    MathSciNet  MATH  Google Scholar 

  17. Gardiner, F.P., Lakic, N.: Quasiconformal Teichmüller Theory . Math. Surveys Monogr., 76, Am. Math. Soc., Providence, RI (2000)

  18. Garnett, J.B.: Bounded analytic functions. Academic Press, New York (1981)

    MATH  Google Scholar 

  19. Gay-Balmaz, F., Marsden, J.E., Ratiu, T.S.: The geometry of the universal Teichmüller space and the Euler-Weil-Petersson equation. Technical report, Ecole Normale Supérieure de Paris, Paris, France (2009)

  20. Gay-Balmaz, F., Ratiu, T.S.: The geometry of the universal Teichmüller space and the Euler–Weil–Petersson equation. Adv. Math. 279, 717–778 (2015)

    MathSciNet  MATH  Google Scholar 

  21. Hamilton, D.E.: BMO and Teichmüller spaces. Ann. Acad. Sci. Fenn. Math. 14, 213–224 (1989)

    MathSciNet  MATH  Google Scholar 

  22. He, Y., Wei, H., Shen, Y.: Some notes on quasisymmetric flows of Zygmund vector fields. J. Math. Anal. Appl. 455, 370–380 (2017)

    MathSciNet  MATH  Google Scholar 

  23. Katznelson, Y., Nag, S., Sullivan, D.: On conformal welding homeomorphisms associated to Jordan curves. Ann. Acad. Sci. Fenn. Ser. A I Math. 15, 293–306 (1990)

    MathSciNet  MATH  Google Scholar 

  24. Kirillov, A.A.: Kähler structure on the \(K\)-orbits of a group of diffeomorphisms of the circle. Funktsional. Anal. i Prilozhen. 21, 42–45 (1987)

    MathSciNet  Google Scholar 

  25. Kirillov, A.A., Yuriev, D.V.: Kähler geometry of the infinite-dimensional homogeneous space \(M=\text{ diff}_+(S^1)/\text{rot }(S^1)\). Funktsional. Anal. i Prilozhen. 21, 35–46 (1987)

    MathSciNet  Google Scholar 

  26. Kushnarev, S.: Teichons: Soliton-like geodesics on universal Teichmüller space. Experiment. Math. 18, 325–336 (2009)

    MathSciNet  MATH  Google Scholar 

  27. Lavrentiev, M.: Boundary problems in the theory of univalent functions. Mat. Sb. (N.S.) 1, 815–844 (1936)

    Google Scholar 

  28. Lavrentiev, M.: Boundary problems in the theory of univalent functions. Am. Math. Soc. Transl. Ser. 2 32, 1–35 (1963)

    MathSciNet  Google Scholar 

  29. Lehto, O.: Univalent Functions and Teichmüller Spaces. Springer, New York (1986)

    MATH  Google Scholar 

  30. Nag, S.: The complex analytic theory of teichmüller spaces. Wiley-Interscience, Hoboken (1988)

    MATH  Google Scholar 

  31. Nag, S., Sullivan, D.: Teichmüller theory and the universal period mapping via quantum calculus and the \(H^{{\frac{1}{2}}}\) space on the circle. Osaka J. Math. 32, 1–34 (1995)

    MathSciNet  MATH  Google Scholar 

  32. Pommerenke, Ch.: Schlichte Funktionen und BMOA. Comment. Math. Helv. 52, 591–602 (1977)

    MathSciNet  MATH  Google Scholar 

  33. Pommerenke, Ch.: Boundary behaviour of conformal maps. Springer, Berlin (1992)

    MATH  Google Scholar 

  34. Radnell, D., Schippers, E., Staubach, W.: A Hilbert manifold structure on the Weil-Petersson class Teichmüller space of bordered Riemann surfaces. Commun. Contemp. Math. 17(42), 1550016, 42 (2015)

  35. Radnell, D., Schippers, E., Staubach, W.: Convergence of the Weil-Petersson metric on the Teichmuller spaces of bordered Riemann surfaces. Commun. Contemp. Math. 19(1), 1650025, 39 (2017)

    MathSciNet  MATH  Google Scholar 

  36. Radnell, D., Schippers, E., Staubach, W.: Quasiconformal Teichmüller theory as an analytical foundation for two-dimensional conformal field theory, In Lie algebras, vertex operator algebras, and related topics. Contemp. Math. 695, 205–238 (2017)

    MATH  Google Scholar 

  37. Semmes, S.: The Cauchy integral, chord-arc curves, and quasiconformal mappings. Proc. Bieberbach Conf. (Purdue University, 1985) (A. Baernstein, P. Duren, A. Marden, and D. Drasin, eds.), Math. Surveys, no. 21, Am. Math. Soc., Providence, R. I., (1986)

  38. Semmes, S.: Estimates for \((\overline{\partial }-\mu \partial )^{-1}\) and Calderón’s theorem on the Cauchy integral. Tran. Am. Math. Soc. 306, 191–232 (1988)

    MATH  Google Scholar 

  39. Semmes, S.: Quasiconformal mappings and chord-arc curves. Tran. Am. Math. Soc. 306, 233–263 (1988)

    MathSciNet  MATH  Google Scholar 

  40. Semmes, S.: Nonlinear fourier analysis. Bull. Am. Math. Soc. 20, 1–18 (1989)

    MathSciNet  MATH  Google Scholar 

  41. Sharon, E., Mumford, D.: 2D-Shape analysis using conformal mapping. Int. J. Comput. Vis. 70, 55–75 (2006)

    Google Scholar 

  42. Shen, Y.: Weil–Petersson Teichmüller space. Am. J. Math. 140, 1041–1074 (2018)

    MATH  Google Scholar 

  43. Shen, Y., Tang, S.: Weil–Petersson Teichmüller space II: smoothness of flow curves of \(H^{\frac{3}{2}}\)-vector fields. Adv. Math. 359, 106891 (2020)

    MathSciNet  MATH  Google Scholar 

  44. Shen, Y., Tang, S., Wu, L.: Weil–Petersson and little Teichmüller spaces on the real line. Ann. Acad. Sci. Fenn. Math. 43, 935–943 (2018)

    MathSciNet  MATH  Google Scholar 

  45. Shen, Y., Wei, H.: Universal Teichmüller space and BMO. Adv. Math. 234, 129–148 (2013)

    MathSciNet  MATH  Google Scholar 

  46. Shen, Y., Wu, L.: Weil–Petersson Teichmüller space III: dependence of Riemann mappings for Weil–Petersson curves. arXiv: 1907.12262

  47. Takhtajan, L., Teo, Lee-Peng: Weil–Petersson metric on the universal Teichmüller space. Mem. Am. Math. Soc. 183(861), (2006)

  48. Viklund, F., Wang, Y.: Interplay between Loewner and Dirichlet energies via conformal welding and flow-lines. arXiv:1903.08525

  49. Wang, Y.: Equivalent descriptions of the Loewner energy. Invent. Math. 218, 573–621 (2019)

    MathSciNet  MATH  Google Scholar 

  50. Wang, Y.: A note on Loewner energy, conformal restriction and Werners measure on self-avoiding loops. arXiv:1810.04578

  51. Wu, L., Hu, Y., Shen, Y.: Weil–Petersson Teichmüller space revisited. J. Math. Anal. Appl. 491, 124304 (2020)

    MathSciNet  MATH  Google Scholar 

  52. Wu, S.: Analytic dependence of Riemann mappings for bounded domains and minimal surfaces. Comm. Pure Appl. Math. 46, 1303–1326 (1993)

    MathSciNet  MATH  Google Scholar 

  53. Zhu, K.: Operator Theory in Function Spaces, Second Edition, Mathematical Surveys and Monographs, vol. 138. American Mathematical Society, Providence, RI (2007)

  54. Zhuravlev, I.V.: A model of the universal Teichmüller space. Sibirsk. Mat. Zh. 27, 75–82 (1986)

    MathSciNet  Google Scholar 

  55. Zinsmeister, M.: Domaines réguliers du plan. Ann. Inst. Fourier (Grenoble) 35, 49–55 (1985)

    MathSciNet  MATH  Google Scholar 

Download references

Acknowledgements

The authors would like to thank the referee for a careful reading of the manuscript and for several corrections which improves the presentation of the paper.

Author information

Authors and Affiliations

  1. Department of Mathematics, Soochow University, Suzhou, 215006, People’s Republic of China

    Yuliang Shen & Li Wu

Authors
  1. Yuliang Shen
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Li Wu
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Yuliang Shen.

Additional information

Communicated by Ngaiming Mok.

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Shen, Y., Wu, L. Weil–Petersson Teichmüller space III: dependence of Riemann mappings for Weil–Petersson curves. Math. Ann. 381, 875–904 (2021). https://doi.org/10.1007/s00208-020-02067-5

Download citation

  • Received:

  • Revised:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00208-020-02067-5

Mathematics Subject Classification

Search

Navigation