Skip to main content

Advertisement

SpringerLink
Log in

Modified Unequal Mixture Scalar Vector Hulthén–Yukawa Potentials Model as a Quark–Antiquark Interaction and Neutral Atoms via Relativistic Treatment Using the Improved Approximation of the Centrifugal Term and Bopp’s Shift Method

  • Published:
Few-Body Systems Aims and scope Submit manuscript

Abstract

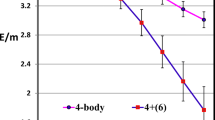
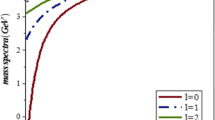
In this work, we present approximate solutions of the modified Klein–Gordon containing an interaction of the modified unequal mixture scalar vector Hulthén–Yukawa potentials model (MUMSVHYa-PM) using the procedure of improved approximation of the centrifugal term, Bopp’s shift method and perturbation theory. This study is realized in the relativistic noncommutative three-dimensional real-space (RNC: 3D-RS) symmetries. The unequal mixture of scalar and vector Hulthén–Yukawa potentials model (UMSVHYa-PM) is extended by including new radial terms to become MUMSVHYa-PM. We show that the new energy shift depends on the global parameters characterizing the noncommutativity space-space and the confining potential parameter (\(V_{0} ,S_{0} ,V_{_{0} }^{'} ,S_{_{0} }^{'} ,\delta )\) in addition to the Gamma function and the discreet atomic quantum numbers \(\left( {j,l,s,m} \right) \). Thus, the new energy eigenvalues appear as a function of the shift energy and the energy to the UMSVHYa-PM and ordinary energy spectrum corresponds to UMSVHYa-PM. The present results are applied in calculating both the energy spectrum and the new mass of mesons such as charmonium and bottomonium that have the quark and antiquark flavor. Furthermore, we obtained new energy eigenvalues of neutral atoms such as (\(^{{22}}\mathrm{Na},^{{12}}{\mathrm{C}}\) and \({}^{{158}}{\mathrm{Au}})\) atoms under MUMSVHYa-PM. We have also discussed some special cases of physical importance.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Similar content being viewed by others

References

  1. H. Hulthén, Uber die Eigenlösungen der Schrödinger chung des Deutrons. Ark. Mat. Astron. Fys. A 28, 5 (1942)

    MathSciNet  Google Scholar 

  2. M.C. Onyeaju, J.O.A. Idiodi, A.N. Ikot, M. Solaimani, H. Hassanabadi, Linear and nonlinear optical properties in spherical quantum dots: generalized Hulthén potential. Few-Body Syst. 59, 793–805 (2016). https://doi.org/10.1007/s00601-016-1110-4

    Article  ADS  Google Scholar 

  3. M. Hosseinpour, F.M. Andrade, E.O. Silva, H. Hassanabadi, Scattering and bound states for the Hulthén potential in a cosmic string background. Eur. Phys. J. C 77, 270 (2017). https://doi.org/10.1140/epjc/s10052-017-4834-5

    Article  ADS  Google Scholar 

  4. O. Bayrak, I. Boztosun, Bound state solutions of the Hulthén potential by using the asymptotic iteration method. Physica Scripta 76(1), 92–96 (2007). https://doi.org/10.1088/0031-8949/76/1/016

    Article  ADS  MathSciNet  MATH  Google Scholar 

  5. Y.P. Varshni, Physics eigenenergies and oscillator strengths for the Hnlthen potential. Phys. Rev. A 41, 4682 (1990). https://doi.org/10.1103/PhysRevA.41.4682

    Article  ADS  MathSciNet  Google Scholar 

  6. B. Durand, L. Durand, Duality for heavy-quark systems. Phys. Rev. D 23(5), 1092–1102 (1981). https://doi.org/10.1103/physrevd.23.1092

    Article  ADS  Google Scholar 

  7. J.A. Obu, P.O. Okoi, U.S. Okorie, Relativistic and nonrelativistic treatment of Hulthén–Kratzer potential model in D-dimensions Indian Journal of Physics. Indian J. Phys. (2019). https://doi.org/10.1007/s12648-019-01638-w

    Article  Google Scholar 

  8. S.M. Ikhdair, R. Sever, Approximate eigenvalue and eigenfunction solutions for the generalized Hulthén Potential with any Angular Momentum. J. Math. Chem 42(3), 461–471 (2006). https://doi.org/10.1007/s10910-006-9115-8

    Article  MATH  Google Scholar 

  9. A.D. Antia, A.N. Ikot, I.O. Akpan, O.A. Awoga, Approximate solutions of the Klein–Gordon equation with unequal scalar and vector modified Hylleraas potential. Indian J. Phys. 87(2), 155–160 (2012). https://doi.org/10.1007/s12648-012-0210-3

    Article  ADS  Google Scholar 

  10. A.N. Ikot, L.E. Akpabio, E.J. Uwah, Bound state solutions of the Klein Gordon equation with the Hulthén potential. Electron. J. Theor. Phys. 8, 225 (2011)

    Google Scholar 

  11. N. Saad, The Klein-Gordon equation with a generalized Hulthén potential in D-dimensions. Phys. Script 76(6), 623–627 (2007). https://doi.org/10.1088/0031-8949/76/6/005

    Article  ADS  MATH  Google Scholar 

  12. D. Agboola, Comment on The Klein–Gordon equation with a generalized Hulthén potential in D dimensions. Phys. Script 81(6), 067001 (2010). https://doi.org/10.1088/0031-8949/81/06/067001

    Article  ADS  MATH  Google Scholar 

  13. J. Stanek, Approximate analytical solutions for arbitrary l-state of the Hulthén potential with an improved approximation of the centrifugal term. Cent. Eur. J. Chem. 9, 737–742 (2011). https://doi.org/10.2478/s11532-011-0050-6

    Article  Google Scholar 

  14. H. Yukawa, On the interaction of elementary particles I. Proc. Phys. Math. Soc. Jpn. 17, 48 (1935). https://doi.org/10.11429/ppmsj1919.17.0_48

    Article  MATH  Google Scholar 

  15. A. A. Rajabi and M. Hamzavi, Approximate analytical solutions of the perturbed Yukawa Potential with Centrifugal Barrier. Zeitschrift Für Naturforschung A 68(6-7) (2013). https://doi.org/10.5560/zna.2013-0023

  16. M. Hamzavi, M. Movahedi, K.E. Thylwe, A.A. Rajabi, Approximate analytical solution of the Yukawa potential with arbitrary angular momenta. Chin. Phys. Lett. 29(8), 080302 (2012). https://doi.org/10.1088/0256-307x/29/8/080302

    Article  ADS  Google Scholar 

  17. E.R. Vrscay, Hydrogen atom with a Yukawa potential: perturbation theory and continued-fractions-Padé approximants at large order. Phys. Rev. A 33(2), 1433–1436 (1986). https://doi.org/10.1103/physreva.33.1433

    Article  ADS  MathSciNet  Google Scholar 

  18. J.M. Ugalde, C. Sarasola, X. Lopez, Atomic and molecular bound ground states of the Yukawa potential. Phys. Rev. A 56(2), 1642–1645 (1997). https://doi.org/10.1103/physreva.56.1642

    Article  ADS  Google Scholar 

  19. J.P. Gazeau, A. Maquet, Bound states in a Yukawa potential: a Sturmian group-theoretical approach. Phys. Rev. A (1979). https://doi.org/10.1103/PhysRevA.20.727

    Article  MathSciNet  Google Scholar 

  20. H. Totsuji, Theory of critical screening radius of energy levels of hydrogen-like atoms in plasmas. J. Phys.Soc. Jpn. 31(2), 584–590 (1971). https://doi.org/10.1143/jpsj.31.584

    Article  ADS  Google Scholar 

  21. C.B. Smith, Bound states in a Debye–Hückel potential. Phys. Rev. 134, A1235 (1964). https://doi.org/10.1103/PhysRev.134.A1235

    Article  ADS  Google Scholar 

  22. G.M. Harris, Attractive two-body interactions in partially ionized plasmas. Phys. Rev. 125, 1131 (1962). https://doi.org/10.1103/PhysRev.125.1131

    Article  ADS  Google Scholar 

  23. J. McEnnan, L. Kissel, R.H. Pratt, Analytic perturbation theory for screened Coulomb potentials: Nonrelativistic case. Phys. Rev. A. 13(2), 532–559 (1976). https://doi.org/10.1103/physreva.13.532

    Article  ADS  Google Scholar 

  24. C.H. Mehta, S.H. Patil, Nonperturbative approach to screened Coulomb potentials. Phys. Rev.A. 17(1), 34–42 (1978). https://doi.org/10.1103/physreva.17.34

    Article  ADS  Google Scholar 

  25. R. Dutt, Y.P. Varshni, An analytic approximation for the energy levels of neutral atoms. Z. Phys. Atoms Nucl. 313, 143–145 (1983). https://doi.org/10.1007/bf01417219

    Article  ADS  Google Scholar 

  26. S.M. Ikhdair, Approximate \(\kappa \)-state solutions to the Dirac–Yukawa problem based on the spin and pseudospin symmetry. Open Phys. (2012). https://doi.org/10.2478/s11534-011-0121-5

    Article  Google Scholar 

  27. A.N. Ikot, E. Maghsoodi, S. Zarrinkamar, N. Salehi, H. Hassanabadi, Psudospin and spin symmetry of generalized Yukawa problems with a Coulomb-like tensor interaction via susyqm. Int. J. Mod. Phys. E 22(07), 1350052 (2013). https://doi.org/10.1142/s0218301313500523

    Article  ADS  Google Scholar 

  28. O. Aydoğdu, R. Sever, The Dirac–Yukawa problem in view of pseudospin symmetry. Phys. Scripta 84(2), 025005 (2011). https://doi.org/10.1088/0031-8949/84/02/025005

    Article  ADS  MATH  Google Scholar 

  29. M. Hamzavi, S.M. Ikhdair, Approximate solution of the Duffin–Kemmer–Petiau equation for a vector Yukawa potential with arbitrary total angular momenta. Few-Body Syst. 54(11), 1753–1763 (2012). https://doi.org/10.1007/s00601-012-0487-y

    Article  ADS  Google Scholar 

  30. M. Hamzavi, S.M. Ikhdair, K.E. Thylwe, Spinless particles in the field of unequal scalar-vector Yukawa potentials. Chin. Phys. B 22(4), 040301 (2013). https://doi.org/10.1088/1674-1056/22/4/040301

    Article  ADS  Google Scholar 

  31. Abdelmadjid Maireche, Investigations on the relativistic interactions in one-electron atoms with modified Yukawa potential for spin 1/2 particles. Int. Front. Sci. Lett. 11, 29–44 (2017). https://doi.org/10.18052/www.scipress.com/IFSL.11.29

    Article  Google Scholar 

  32. A.I. Ahmadov, S.M. Aslanova, M.S. Orujova, S.V. Badalov, S.H. Dong, Approximate bound state solutions of the Klein–Gordon equation with the linear combination of Hulthén and Yukawa potentials. Phys. Lett. A 383(24), 3010–3017 (2019). https://doi.org/10.1016/j.physleta.2019.06.043

    Article  ADS  MathSciNet  Google Scholar 

  33. S. Capozziello, G. Lambiase, G. Scarpetta, Generalized uncertainty principle from quantum geometry. Int. J. Theor. Phys. 39, 15–22 (2000). https://doi.org/10.1023/A:1003634814685

    Article  MathSciNet  MATH  Google Scholar 

  34. S. Doplicher, K. Fredenhagen, J.E. Roberts, Spacetime quantization induced by classical gravity. Phys. Lett. B 331(1–2), 39–44 (1994). https://doi.org/10.1016/0370-2693(94)90940-7

    Article  ADS  MathSciNet  Google Scholar 

  35. E. Witten, Refection on the fate spacetime. Phys. Today 49(4), 24 (1996). https://doi.org/10.1063/1.881493

    Article  MathSciNet  Google Scholar 

  36. A. Kempf, G. Mangano, R.B. Mann, Hilbert space representation of the minimal length uncertainty relation. Phys. Rev. D 52(2), 1108–1118 (1995). https://doi.org/10.1103/physrevd.52.1108

    Article  ADS  MathSciNet  Google Scholar 

  37. F. Scardigli, Some heuristic semi-classical derivations of the Planck length, the Hawking effect and the Unruh effect. Il Nuovo Cimento B Ser. 11 110(9), 1029–1034 (1995). https://doi.org/10.1007/bf02726152

    Article  Google Scholar 

  38. R.J. Adler, D.I. Santigo, On gravity and the uncertainty principal. Mod. Phys. Lett. A 14(20), 1371–1381 (1999). https://doi.org/10.1142/s0217732399001462

    Article  ADS  Google Scholar 

  39. T. Kanazawa, G. Lambiase, G. Vilasi, A. Yoshioka, Noncommutative Schwarzschild geometry and generalized uncertainty principle. Eur. Phys. J. C (2019). https://doi.org/10.1140/epjc/s10052-019-6610-1

    Article  Google Scholar 

  40. F. Scardigli, Generalized uncertainty principle in quantum gravity from micro-black hole gedanken experiment. Phys.Lett. B 452(1–2), 39–44 (1999). https://doi.org/10.1016/s0370-2693(99)00167-7

    Article  ADS  Google Scholar 

  41. Abdelmadjid Maireche, Nonrelativistic treatment of Hydrogen-like and neutral atoms subjected to the generalized perturbed Yukawa potential with centrifugal barrier in the symmetries of noncommutative Quantum mechanics. Int. J. Geom. Meth. Mod. Phys. (2020). https://doi.org/10.1142/S021988782050067X

    Article  MathSciNet  Google Scholar 

  42. P.M. Ho, H.C. Kao, Noncommutative quantum mechanics from noncommutative quantum field theory. Phys. Rev. Lett. (2002). https://doi.org/10.1103/physrevlett.88.151602

    Article  MathSciNet  Google Scholar 

  43. P. Gnatenko, Parameters of noncommutativity in Lie-algebraic noncommutative space. Phys. Rev. D 99(2), 026009-1 (2019). https://doi.org/10.1103/physrevd.99.026009

    Article  ADS  MathSciNet  Google Scholar 

  44. Abdelmadjid Maireche, A new model for describing heavy-light mesons in the extended nonrelativistic quark model under a new modified potential containing Cornell. Gaussian And Inverse Square Terms in The Symmetries Of NCQM, To Physics Journal 3, 197–215 (2019). Retrieved fromhttps://purkh.com/index.php/tophy/article/view/500

  45. O. Bertolami, J.G. Rosa, C.M.L. De Aragao, P. Castorina, D. Zappala, Scaling of varialbles and the relation between noncommutative parameters in noncommutative quantum mechanics. Mod. Phys. Lett. A 21(10), 795–802 (2006). https://doi.org/10.1142/s0217732306019840

    Article  ADS  Google Scholar 

  46. Abdelmadjid Maireche, A recent study of excited energy levels of diatomics for modified more general exponential screened Coulomb potential: extended quantum mechanics. J. Nano- Electron. Phys. 9(3), 03021 (2017). https://doi.org/10.21272/jnep.9(3).03021

    Article  Google Scholar 

  47. E. F. Djemaï and H. Smail, On Quantum Mechanics on Noncommutative Quantum Phase Space, Commun. Theor. Phys. (Beijing, China) 41(6), 837-844 (2004). https://doi.org/10.1088/0253-6102/41/6/837

  48. Y. Yuan, K. Li, J.H. Wang, C.Y. Chen, Spin-1/2 relativistic particle in a magnetic field in NC phase space. Chin. Phys. C 34(5), 543–547 (2010). https://doi.org/10.1088/1674-1137/34/5/005

    Article  ADS  Google Scholar 

  49. O. Bertolami, P. Leal, Aspects of phase-space noncommutative quantum mechanics. Phys. Lett. B 750, 6–11 (2015). https://doi.org/10.1016/j.physletb.2015.08.024

    Article  ADS  MathSciNet  MATH  Google Scholar 

  50. C. Bastos, O. Bertolami, N.C. Dias, J.N. Prata, Weyl–Wigner formulation of noncommutative quantum mechanics. J. Math. Phys. 49(7), 072101 (2008). https://doi.org/10.1063/1.2944996

    Article  ADS  MathSciNet  MATH  Google Scholar 

  51. J. Zhang, Fractional angular momentum in non-commutative spaces. Phys. Lett. B 584(1–2), 204–209 (2004). https://doi.org/10.1016/j.physletb.2004.01.049

    Article  ADS  MathSciNet  MATH  Google Scholar 

  52. J. Gamboa, M. Loewe, J.C. Rojas, Noncommutative quantum mechanics. Phys. Rev. D 64, 067901 (2001). https://doi.org/10.1103/PhysRevD.64.067901

    Article  ADS  MathSciNet  Google Scholar 

  53. M. Chaichian, Sheikh-Jabbari, A. Tureanu, Hydrogen atom spectrum and the lamb shift in noncommutative QED. Phys. Rev. Lett. 86(13), 2716–2719 (2001). https://doi.org/10.1103/physrevlett.86.2716

    Article  ADS  Google Scholar 

  54. Abdelmadjid Maireche, Nonrelativistic Bound State Solutions of the Modified 2D-Killingbeck Potential Involving 2D-Killingbeck Potential and Some Central Terms for Hydrogenic Atoms and Quarkonium System, J. Nano- Electron. Phys. 11 No 4, 04024 (2019). https://doi.org/10.21272/jnep.11(4).04024

  55. Abdelmadjid Maireche, New bound state energies for spherical quantum dots in presence of a confining potential model at Nano and Plank’s Scales. Nano.World. J. 1(4), 122–129 (2016). https://doi.org/10.17756/nwj.2016-016

    Article  Google Scholar 

  56. M.A. De Andrade, C. Neves, Noncommutative mapping from the symplectic formalism. J. Math. Phys. 59(1), 012105 (2018). https://doi.org/10.1063/1.4986964

    Article  ADS  MathSciNet  MATH  Google Scholar 

  57. E.M.C. Abreu, C. Neves, W. Oliveira, Noncommutativity from the symplectic point of view. Int. J. Mod. Phys. A 21, 5359 (2006). https://doi.org/10.1142/s0217751x06034094

    Article  ADS  MathSciNet  MATH  Google Scholar 

  58. E.M.C. Abreu, J.A. Neto, A.C.R. Mendes, C. Neves, W. Oliveira, M.V. Marcial, Lagrangian formulation for noncommutative nonlinear systems. Int. J. Mod. Phys. A. 27, 1250053 (2012). https://doi.org/10.1142/s0217751x12500534

    Article  ADS  MathSciNet  MATH  Google Scholar 

  59. L. Mezincescu, "Star operation in quantum mechanics," e-print arXiv: hep-th/0007046v2

  60. J. Wang, K. Li, The HMW effect in noncommutative quantum mechanics. J.Phys.A Math.Theor. 40(9), 2197–2202 (2007). https://doi.org/10.1088/1751-8113/40/9/021

    Article  ADS  MathSciNet  MATH  Google Scholar 

  61. K. Li, J. Wang, The topological AC effect on non-commutative phase space. Eur. Phys. J. C 50(4), 1007–1011 (2007). https://doi.org/10.1140/epjc/s10052-007-0256-0

    Article  ADS  MATH  Google Scholar 

  62. Abdelmadjid Maireche, New nonrelativistic bound state solutions of the NGECSC potential involving GECSC potential and new central terms for hydrogen atom. Afr. Rev. Phys. 13(0023), 142–149 (2018)

    Google Scholar 

  63. Abdelmadjid Maireche, A New Nonrelativistic Investigation for the Lowest Excitations States ofInteractions in one-electron atoms, muonic, hadronic and rydberg atoms with modified inverse powerPotential. Int. Front. Sci. Lett. 9, 33–46 (2016). https://doi.org/10.18052/www.scipress.com/IFSL.9.33

    Article  Google Scholar 

  64. Abdelmadjid Maireche, New quantum atomic spectrum of Schrödinger equation with pseudo harmonic potential in both noncommutative three dimensional spaces and phases. Lat. Am. J. Phys. Educ. 9(1), 1301 (2015)

    Google Scholar 

  65. Abdelmadjid Maireche, A theoretical investigation of the Schrödinger equation for a modified two-dimensional purely sextic double-well potential. YJES 16, 41–54 (2018)

    Google Scholar 

  66. A. Maireche, Extended of the Schrödinger equation with new coulomb potentials plus linear and harmonic radial terms in the symmetries of noncommutative quantum mechanics. J. Nano- Electron. Phys. 10(6), 06015-1–06015-7 (2018). https://doi.org/10.21272/jnep.10(6).06015

  67. Abdelmadjid Maireche, The Klein–Gordon equation with modified Coulomb plus inverse-square potential in the noncommutative three-dimensional space. Mod. Phys. Lett. A 35(5), 052050015 (2020). https://doi.org/10.1142/s0217732320500157

    Article  ADS  MathSciNet  MATH  Google Scholar 

  68. S. Dong, S.H. Dong, H. Bahlouli, V.B. Bezerra, Algebraic approach to the Klein–Gordon equation with hyperbolic scare potential. Int. J. Mod. Phys. E 20(01), 55–61 (2011). https://doi.org/10.1142/s0218301311017326

    Article  ADS  Google Scholar 

  69. W.C. Qiang, S.H. Dong, Analytical approximations to the l-wave solutions of the Klein–Gordon equation for a second Pöschl-Teller like potential. Phys. Lett. A 372(27–28), 4789–4792 (2008). https://doi.org/10.1016/j.physleta.2008.05.020

    Article  ADS  MATH  Google Scholar 

  70. A. Maireche, The Klein–Gordon equation with modified coulomb potential plus inverse-square-root potential in three-dimensional noncommutative space. Phys. J. 3, 186–196 (2019)

    Google Scholar 

  71. H. Motavalli, A.R. Akbarieh, Mod. Phys. Lett. A 25(29), 2523–2528 (2010). https://doi.org/10.1142/s0217732310033529

    Article  ADS  Google Scholar 

  72. M. Darroodi, H. Mehraban, H. Hassanabadi, The Klein-Gordon equation with the Kratzer potential in the noncommutative space. Mod. Phys. Lett. A 33(35), 1850203 (2018). https://doi.org/10.1142/s0217732318502036

    Article  ADS  MathSciNet  MATH  Google Scholar 

  73. A. Saidi, M.B. Sedra, Spin-one (1 + 3)-dimensional DKP equation with modified Kratzer potential in the non-commutative space. Mod. Phys. Lett. A 35(5), 2050014 (2019). https://doi.org/10.1142/s0217732320500145

    Article  ADS  MathSciNet  MATH  Google Scholar 

  74. M. Badawi, N. Bessis, G. Bessis, On the introduction of the rotation-vibration coupling in diatomic molecules and the factorization method. J. Phys. B Am. Mol.Phys. 5(8), L157–L159 (1972). https://doi.org/10.1088/0022-3700/5/8/004

    Article  ADS  Google Scholar 

  75. R.L. Greene, C. Aldrich, Variational wave functions for a screened Coulomb potential. Phys. Rev. A 14(6), 2363–2366 (1976). https://doi.org/10.1103/physreva.14.2363

    Article  ADS  Google Scholar 

  76. S.H. Dong, W.C. Qiang, G.H. Sun, V.B. Bezerra, Analytical approximations to the l-wave solutions of the Schrödinger equation with the Eckart potential. J. Phys. A Math.Theor. 40(34), 10535–10540 (2007). https://doi.org/10.1088/1751-8113/40/34/010

    Article  ADS  MATH  Google Scholar 

  77. A.C. Onate, A.N. Ikot, M.C. Onyeaju, O. Ebomwonyi, Dirac Equation with Unequal Scalar and Vector Potentials under Spin and Pseudospin Symmetry. Sri Lankan J. Phys. 19(1), 17–36 (2018). https://doi.org/10.4038/sljp.v19i1.8043

    Article  ADS  Google Scholar 

  78. M. Hamzavi, K.E. Thylwe, A.A. Rajabi, Approximate bound states solution of the Hellmann potential. Commun. Theor. Phys. 60, 1–8 (2013). https://doi.org/10.1088/0253-6102/60/1/01.52

    Article  ADS  MATH  Google Scholar 

  79. O.J. Oluwadare, K.E. Thylwe, K.J. Oyewumi, Non-relativistic phase shifts for Scattering on generalized radial Yukawa potential. Commun. Theor. Phys. 65, 434–440 (2016). https://doi.org/10.1088/0253-6102/65/4/434.53

    Article  ADS  MathSciNet  MATH  Google Scholar 

  80. W.C. Qiang, S.H. Dong, Analytical approximations to the solutions of the Manning–Rosen potential with centrifugal term. Phys. Lett. A 368(1–2), 13–17 (2007). https://doi.org/10.1016/j.physleta.2007.03.057

    Article  ADS  MathSciNet  MATH  Google Scholar 

  81. W.C. Qiang, S.H. Dong, The Manning–Rosen potential studied by a new approximate scheme to the centrifugal term. Phys. Scripta 79(4), 045004 (2009). https://doi.org/10.1088/0031-8949/79/04/045004

    Article  ADS  MATH  Google Scholar 

  82. G. Wei, Z. Zhen, S. Dong, The relativistic bound and scattering states of the Manning–Rosen potential with an improved new approximate scheme to the centrifugal term. Cent. Eur. J. Phys. 7, 175–183 (2009). https://doi.org/10.2478/s11534-008-0143-9

    Article  Google Scholar 

  83. S. Gradshteyn and I. M. Ryzhik, Table of Integrals, Series and Products (7th. ed.; Elsevier, edited by Alan Jeffrey, University of Newcastle upon Tyne, England and Daniel Zwillinger (Rensselaer Polytechnic Institute USA), 2007)

  84. M. Abu-Shady, T.A. Abdel-Karim, S.Y. Ezz-Alarab, Masses and thermodynamic properties of heavy mesons in the non-relativistic quark model using the Nikiforov-Uvarov method. J. Egypt. Math.Soc 27, 14 (2019). https://doi.org/10.1186/s42787-019-0014-0

    Article  MathSciNet  MATH  Google Scholar 

  85. M. Abu-Shady, S.Y. Ezz-Alarab, Trigonometric Rosen–Morse potential as a quark–antiquark interaction potential for meson properties in the non-relativistic quark model using EAIM. Few-Body Syst. (2019). https://doi.org/10.1007/s00601-019-1531-y

    Article  Google Scholar 

  86. N.V. Maksimenko, S.M. Kuchin, Electric polarizability of pions in semirelativistic quark model. Phys. Particle Nucl. Lett. 9(2), 134–138 (2012). https://doi.org/10.1134/s1547477112020112and

    Article  ADS  Google Scholar 

  87. C.Y. Chen, D.S. Sun, F.L. Lu, Approximate analytical solutions of Klein–Gordon equation with Hulthén potentials for nonzero angular momentum. Phys. Lett. A 370(3–4), 219–221 (2007). https://doi.org/10.1016/j.physleta.2007.05.079

    Article  ADS  MathSciNet  MATH  Google Scholar 

Download references

Acknowledgements

We are grateful to the referee for giving such constructive comments, which considerably improved the quality of the paper. This work has been partly supported by the AMHESR and DGRST under project no. B00L02UN280120180001 and by the Laboratory of Physics and Material Chemistry, University of M’sila-ALGERIA.

Author information

Authors and Affiliations

  1. Laboratory of Physics and Material Chemistry, Physics department, Sciences Faculty, University of M’sila-Algeria, M’sila, Algeria

    Abdelmadjid Maireche

Authors
  1. Abdelmadjid Maireche
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Abdelmadjid Maireche.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Maireche, A. Modified Unequal Mixture Scalar Vector Hulthén–Yukawa Potentials Model as a Quark–Antiquark Interaction and Neutral Atoms via Relativistic Treatment Using the Improved Approximation of the Centrifugal Term and Bopp’s Shift Method. Few-Body Syst 61, 30 (2020). https://doi.org/10.1007/s00601-020-01559-z

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s00601-020-01559-z

Search

Navigation