Impact of Imperfect Channel State Information on the Physical Layer Security in D2D Wireless Networks Using Untrusted Relays

Abstract

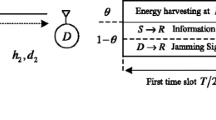
In this paper, we investigate the impact of channel estimation errors on the physical layer security of an overlaying device-to-device (D2D) wireless network with an amplify-and-forward untrusted relay. An untrusted relay assists D2D communication while may capture the confidential data. Under the practical assumption of imperfect channel state information (ICSI) for the relay-to-receiver D2D link, we take into account optimal power allocation (OPA) problem to maximize the achievable secrecy rate of two different scenarios which are without jamming and with friendly jamming. Based on these OPA solutions, we study the secrecy performance of the two scenarios by driving closed-form expressions for the ergodic secrecy rate (ESR) in Rayleigh fading channel. We also calculate the high signal-to-noise ratio (SNR) slope and high SNR power offset of the optimized scenarios by finding the asymptotic ESR. Numerical results confirm the accuracy of our proposed theoretical analysis. The results also demonstrate that our proposed OPAs enhance the ESR performance compared with other power allocation techniques. Moreover, they show the effect of ICSI on the ESR such that as channel estimation error grows, the ESR performance reduction is occurred.

Appendices

Appendix 1

Let define ${\upgamma }_{\text{rr}} \triangleq \frac{{{\upgamma }_{{{\text{r}}2}} }}{{{\upgamma }_{{1{\text{r}}}} }}$. Then the pdf of $\gamma_{rr}$ can be calculated as

$$\begin{aligned} f_{{{\upgamma }_{\text{rr}} }} \left( x \right) & = \frac{\partial }{\partial x}F_{{{\upgamma }_{\text{rr}} }} \left( x \right) \\ & = \frac{\partial }{\partial x}\left( {\Pr \left\{ {{\upgamma }_{\text{rr}} < x} \right\}} \right) \\ & = \overbrace {{\frac{\partial }{\partial x}\mathop \int \limits_{0}^{\infty } \mathop \int \limits_{0}^{\beta x} f_{{{\upgamma }_{{{\text{r}}2}} }} \left( \alpha \right)f_{{{\upgamma }_{{1{\text{r}}}} }} \left( \beta \right)d\alpha d\beta }}^{{A_{1} }} = \mathop \int \limits_{0}^{\infty } \left( {\frac{\partial }{\partial x}\mathop \int \limits_{0}^{\beta x} f_{{{\upgamma }_{{{\text{r}}2}} }} \left( \alpha \right)d\alpha } \right)f_{{{\upgamma }_{{1{\text{r}}}} }} \left( \beta \right)d\beta \\ & = \mathop \int \limits_{0}^{\infty } \left( {\overbrace {{\beta f_{{{\upgamma }_{{{\text{r}}2}} }} \left( {\beta x} \right)}}^{{A_{2} }}} \right)f_{{{\upgamma }_{{1{\text{r}}}} }} \left( \beta \right)d\beta = \overbrace {{\frac{{{{\bar{\upgamma }}}_{r2} }}{{{{\bar{\upgamma }}}_{1r} }}\left( {\frac{1}{{\left( {x + \frac{{{{\bar{\upgamma }}}_{r2} }}{{{{\bar{\upgamma }}}_{1r} }}} \right)^{2} }}} \right)}}^{{A_{3} }} = \frac{{{{\bar{\upgamma }}}_{rr} }}{{\left( {x + {{\bar{\upgamma }}}_{rr} } \right)^{2} }} , \\ \end{aligned}$$

(66)

where ${{\bar{\upgamma }}}_{rr} \triangleq \frac{{{{\bar{\upgamma }}}_{r2} }}{{{{\bar{\upgamma }}}_{1r} }}$. We remark that $A_{1}$ is valid since $\gamma_{1r}$ and $\gamma_{r2}$ are independent random variables. Moreover, $A_{2}$ follows from using [26, Eq. 0.410] and $A_{3}$ follows from substituting the pdfs of $\gamma_{1r}$ and $\gamma_{r2}$, and applying [26, Eq. 4.381.4].

Let consider $\gamma_{rr} + \gamma_{e} = \frac{{\gamma_{r2} }}{{\gamma_{1r} }} + \gamma_{e} = \vartheta$ and $\gamma_{rr} \le 1.\,{\text{The pdf of }}\vartheta$ is given by

$$\begin{aligned} F_{\vartheta } \left( \gamma \right) & = \Pr \left\{ {\vartheta < \gamma } \right\} \\ & = \Pr \left\{ {\left( {{\upgamma }_{\text{rr}} + \gamma_{e} } \right) < \gamma } \right\} = \Pr \left\{ {\gamma_{e} < \left( {\gamma - {\upgamma }_{\text{rr}} } \right){| \upgamma }_{\text{rr}} } \right\} \\ & = E_{{{\upgamma }_{\text{rr}} }} \left\{ {F_{{{\upgamma }_{e} }} \left( {\gamma - {\upgamma }_{\text{rr}} } \right)} \right\} = \overbrace {{1 - E_{{{\upgamma }_{\text{rr}} }} \left\{ {e^{{ - \frac{{\left( {\gamma - {\upgamma }_{\text{rr}} } \right)}}{{{{\bar{\upgamma }}}_{e} }}}} } \right\}}}^{{A_{4} }} \\ & = 1 - \mathop \int \limits_{0}^{\infty } e^{{ - \frac{{\left( {\gamma - {\upgamma }_{\text{rr}} } \right)}}{{{{\bar{\upgamma }}}_{e} }}}} f_{{{\upgamma }_{\text{rr}} }} \left( x \right)dx = 1 - \overbrace {{{{\bar{\upgamma }}}_{rr} e^{{ - \frac{\gamma }{{{{\bar{\upgamma }}}_{e} }}}} \mathop \int \limits_{0}^{1} e^{{\frac{x}{{{{\bar{\upgamma }}}_{e} }}}} \left( {\frac{1}{{\left( {x + {{\bar{\upgamma }}}_{rr} } \right)^{2} }}} \right)dx}}^{{A_{5} }}, \\ \end{aligned}$$

(67)

where $A_{4}$ follows from substituting the cdf of $\gamma_{e}$ and, $A_{5}$ follows from replacing the pdf of $\gamma_{rr} .$ Now, using Eqs. (29) and (31), we have

$$\begin{aligned} I_{{d_{2} }} & = E\left[ {\ln \left( {1 + (\gamma_{rr} + \gamma_{e} } \right))} \right] \\ & = \mathop \int \limits_{0}^{\infty } \frac{{1 - F_{\vartheta } \left( \gamma \right)}}{1 + \gamma }d\gamma \\ & = \bar{\gamma }_{rr} \left( {\underbrace {{\mathop \int \limits_{0}^{1} \frac{{e^{{\frac{x}{{\bar{\gamma }_{e} }}}} }}{{\left( {x + \bar{\gamma }_{rr} } \right)^{2} }}dx}}_{{i_{{A_{1} }} }} \times \underbrace {{\mathop \int \limits_{0}^{\infty } \frac{{e^{{ - \frac{\gamma }{{\bar{\gamma }_{e} }}}} }}{1 + \gamma }d\gamma }}_{{i_{{A_{2} }} }}} \right), \\ \end{aligned}$$

(68)

where using [26, Eq. 3.385], the phrase $i_{{A_{1} }}$ is obtained as follows

$$i_{{A_{1} }} = \left( {\frac{{\bar{\gamma }_{1r} }}{{\bar{\gamma }_{r2} }}} \right)^{2} {\rm B}\left( {1,1} \right)\varPhi_{1} \left( {1,2,2, - \frac{{\bar{\gamma }_{1r} }}{{\bar{\gamma }_{r2} }},\frac{1}{{\bar{\gamma }_{e} }}} \right) = \left( {\frac{1}{{\bar{\gamma }_{rr} }}} \right)^{2} {\rm B}\left( {1,1} \right)\varPhi_{1} \left( {1,2,2, - \frac{1}{{\bar{\gamma }_{rr} }},\frac{1}{{\bar{\gamma }_{e} }}} \right),$$

(69)

where $B\left( {x,y} \right)$ is the Beta function [26]

$${\rm B}\left( {x,y} \right) = \mathop \int \limits_{0}^{1} t^{x - 1} \left( {1 - t} \right)^{y - 1} dt,\quad Re\left( x \right),\quad Re\left( y \right) > 0,$$

(70)

where ${\rm B}\left( {1,1} \right) = 1.$ In Eq. (68), the phrase $i_{{A_{2} }}$ can be obtained as follows using [26, Eq. 3.382.4]

$$i_{{A_{2} }} = e^{{\frac{1}{{{{\bar{\upgamma }}}_{e} }}}} \varGamma \left( {0,\frac{1}{{{{\bar{\upgamma }}}_{e} }}} \right) ,$$

(71)

where $\varGamma \left( {0, x} \right)$ is in Eq. (34). Therefore, by substituting Eqs. (69) and (71) into Eq. (68), $I_{{d_{2} }}$ is derived as

$$I_{{d_{2} }} = \frac{1}{{{{\bar{\upgamma }}}_{rr} }}\varPhi_{1} \left( {1,2,2, - \frac{1}{{{{\bar{\upgamma }}}_{rr} }},\frac{1}{{{{\bar{\upgamma }}}_{e} }}} \right)e^{{\frac{1}{{{{\bar{\upgamma }}}_{e} }}}} 4\sqrt 2 \pi a_{1} a_{2} \mathop \sum \limits_{p = 1}^{T + 1} \mathop \sum \limits_{q = 1}^{{T^{{\prime }} + 1}} \sqrt {b_{p} } e^{{ - 4b_{p} b_{q} x}} .$$

(72)

Appendix 2

Let define ${\upgamma }_{\text{rr}} \triangleq \frac{{{\upgamma }_{{{\text{r}}2}} }}{{{\upgamma }_{{1{\text{r}}}} }}$, then the pdf of $\gamma_{rr}$ is as Eq. (66). Under the assumption of $\gamma_{rr} \le 1,$ the cdf of $\gamma_{R}$ in Eq. (40) is calculated as

$$\begin{aligned} F_{{{\upgamma }_{R} }} \left( \gamma \right) & = \Pr \left\{ {{\upgamma }_{R} < \gamma } \right\} \\ & = \Pr \left\{ {\sqrt {\frac{{1 + \gamma_{e} }}{{1 + {\upgamma }_{\text{rr}} }}} < \gamma } \right\} = \Pr \left\{ {\gamma_{e} < \gamma^{2} \left( {1 + {\upgamma }_{\text{rr}} } \right) - 1 {| \upgamma }_{\text{rr}} } \right\} \\ & = E_{{{\upgamma }_{\text{rr}} }} \left\{ {F_{{{\upgamma }_{e} }} \left( {\gamma^{2} \left( {1 + {\upgamma }_{\text{rr}} } \right) - 1} \right)} \right\} = \overbrace {{1 - E_{{{\upgamma }_{\text{rr}} }} \left\{ {e^{{ - \frac{{\left( {\gamma^{2} \left( {1 + {\upgamma }_{\text{rr}} } \right) - 1} \right)}}{{{{\bar{\upgamma }}}_{e} }}}} } \right\}}}^{{B_{1} }} \\ & = 1 - \mathop \int \limits_{0}^{\infty } e^{{ - \frac{{\left( {\gamma^{2} \left( {1 + x} \right) - 1} \right)}}{{{{\bar{\upgamma }}}_{e} }}}} f_{{{\upgamma }_{\text{rr}} }} \left( x \right)dx \\ & = 1 - \overbrace {{{{\bar{\upgamma }}}_{rr} e^{{\frac{1}{{{{\bar{\upgamma }}}_{e} }}}} \mathop \int \limits_{0}^{1} e^{{ - \frac{{\gamma^{2} \left( {1 + {\text{x}}} \right)}}{{{{\bar{\upgamma }}}_{e} }}}} \left( {\frac{1}{{\left( {x + {{\bar{\upgamma }}}_{rr} } \right)^{2} }}} \right)dx}}^{{B_{2} }}, \\ \end{aligned}$$

(73)

where $B_{1}$ and $B_{2}$ follow from substituting the cdf of $\gamma_{e}$ and the pdf of $\gamma_{rr} ,$ respectively. Now, using Eq. (31), we get

$$\begin{aligned} I_{R}^{\prime} & = E\left[ {\ln \left( {1 + {\upgamma }_{\text{R}} } \right)} \right] \\ & = \mathop \int \limits_{0}^{\infty } \frac{{1 - F_{{{\upgamma }_{R} }} \left( \gamma \right)}}{1 + \gamma }d\gamma \\ & = {{\bar{\upgamma }}}_{rr} e^{{\frac{1}{{{{\bar{\upgamma }}}_{e} }}}} \left( {\mathop \int \limits_{0}^{1} \mathop \int \limits_{0}^{\infty } \frac{{e^{{ - \frac{{\gamma^{2} \left( {1 + x} \right)}}{{{{\bar{\upgamma }}}_{e} }}}} }}{{\left( {1 + \gamma } \right)\left( {x + {{\bar{\upgamma }}}_{rr} } \right)^{2} }}d\gamma dx} \right) \\ & = {{\bar{\upgamma }}}_{rr} e^{{\frac{1}{{{{\bar{\upgamma }}}_{e} }}}} \left( {\mathop \int \limits_{0}^{1} \frac{B}{{\left( {x + {{\bar{\upgamma }}}_{rr} } \right)^{2} }}dx} \right) , \\ \end{aligned}$$

(74)

where

$$B = \underbrace {{\mathop \int \limits_{0}^{\infty } \frac{{e^{{ - \frac{{\left( {1 + x} \right)u}}{{{{\bar{\upgamma }}}_{e} }}}} }}{\sqrt u }du}}_{{i_{{B_{1} }} }} - \underbrace {{\mathop \int \limits_{0}^{\infty } \frac{{e^{{ - \frac{{\left( {1 + x} \right)u}}{{{{\bar{\upgamma }}}_{e} }}}} }}{\sqrt u + 1}du}}_{{i_{{B_{2} }} }},$$

(75)

where we applied the change of variable $u = \gamma^{2} .$ Moreover, regarding [26, Eq. 3.361.1], the phrase $i_{{B_{1} }}$ is calculated as

$$i_{{B_{1} }} = \sqrt {\frac{{\pi {{\bar{\upgamma }}}_{e} }}{x + 1}} ,$$

(76)

and by using the MAPLE, $i_{{B_{2} }}$ can be expressed as

$$i_{{B_{2} }} = \frac{{{{\bar{\upgamma }}}_{e} G_{2 3}^{3 2} \left( {\frac{x + 1}{{{{\bar{\upgamma }}}_{e} }}{|}\begin{array}{*{20}c} {\frac{1}{2},1} \\ {1,1,\frac{1}{2}} \\ \end{array} } \right)}}{{\pi \left( {x + 1} \right)}} ,$$

(77)

where $G_{2 3}^{3 2} \left( {\frac{x + 1}{{{{\bar{\upgamma }}}_{e} }}{|}\begin{array}{*{20}c} {\frac{1}{2},1} \\ {1,1,\frac{1}{2}} \\ \end{array} } \right)$ is expressed as [26, Eq. 9.301]

$$G_{2 3}^{3 2} \left( {\frac{x + 1}{{{{\bar{\upgamma }}}_{e} }}{|}\begin{array}{*{20}c} {\frac{1}{2},1} \\ {1,1,\frac{1}{2}} \\ \end{array} } \right) = \frac{1}{2\pi i}\mathop \int \limits_{ - \infty }^{\infty } \varGamma \left( {1 - s} \right)\varGamma \left( {\frac{1}{2} - s} \right)\varGamma \left( s \right)\left( {\frac{x + 1}{{{{\bar{\upgamma }}}_{e} }}} \right)^{s} ds,$$

(78)

where the Gamma function $\varGamma \left( z \right)$ is as follows

$$\varGamma \left( z \right) = \mathop \int \limits_{0}^{\infty } t^{z - 1} e^{ - t} dt.$$

(79)

As such, $I_{R}^{\prime}$ can be evaluated as

$$I_{R}^{\prime} = {{\bar{\upgamma }}}_{rr} e^{{\frac{1}{{{{\bar{\upgamma }}}_{e} }}}} \left( {\eta - \mathop \int \limits_{0}^{1} \frac{{{{\bar{\upgamma }}}_{e} G_{2 3}^{3 2} \left( {\frac{x + 1}{{{{\bar{\upgamma }}}_{e} }}{|}\begin{array}{*{20}c} {\frac{1}{2},1} \\ {1,1,\frac{1}{2}} \\ \end{array} } \right)}}{{\pi \left( {x + 1} \right)\left( {x + {{\bar{\upgamma }}}_{rr} } \right)^{2} }}dx} \right)$$

(80)

where

$$\eta = \sqrt {\pi {{\bar{\upgamma }}}_{e} } \times \left( {\frac{{\sqrt 2 {{\bar{\upgamma }}}_{rr} ({{\bar{\upgamma }}}_{rr} - 1)^{{\frac{3}{2}}} + {{\bar{\upgamma }}}_{rr}^{3} k_{2} - {{\bar{\upgamma }}}_{rr}^{3} k_{1} - {{\bar{\upgamma }}}_{rr} \left( {{{\bar{\upgamma }}}_{rr} - 1} \right)^{{\frac{3}{2}}} - \left( {{{\bar{\upgamma }}}_{rr} - 1} \right)^{{\frac{3}{2}}} - {{\bar{\upgamma }}}_{rr} k_{2} + {{\bar{\upgamma }}}_{rr} k_{1} }}{{{{\bar{\upgamma }}}_{rr} \left( {{{\bar{\upgamma }}}_{rr} + 1} \right)\left( {{{\bar{\upgamma }}}_{rr} - 1} \right)^{{\frac{5}{2}}} }}} \right)$$

(81)

where $k_{1} = \arctan \left( {\frac{1}{{\sqrt {{{\bar{\upgamma }}}_{rr} - 1} }}} \right)$ and $k_{2} = {\text{arctan}}\left( {\frac{\sqrt 2 }{{\sqrt {{{\bar{\upgamma }}}_{rr} - 1} }}} \right)$.

Appendix 3

Let define $A = 1 + \varOmega$ and $z = \frac{{{\upgamma }_{r2} }}{{{\upgamma }_{1r} }}.$ By applying the change of variable $U = \sqrt {\frac{A}{1 + z}} ,$ the cdf of $U$ is given by

$$\begin{aligned} F_{U} \left( u \right) & = \Pr \left\{ {U \le u} \right\} \\ & = \Pr \left\{ {\sqrt {\frac{A}{1 + z}} \le u} \right\} \\ & = \Pr \left\{ {z \ge \frac{A}{{u^{2} }} - 1} \right\} = 1 - \Pr \left\{ {z < \frac{A}{{u^{2} }} - 1} \right\} \\ & = 1 - F_{Z} \left( {\frac{A}{{u^{2} }} - 1} \right) = 1 - F_{Z} \left( z \right), \\ \end{aligned} .$$

(82)

where $F_{Z} \left( z \right)$ was calculated in [25]. Using Eq. (31), $I_{R}^{\prime L}$ is obtained as

$$\begin{aligned} I_{R}^{\prime L} & = E\left[ {\ln \left( {1 + \gamma_{R} } \right)} \right] \\ & = E\left[ {\ln \left( {1 + \sqrt {\frac{1 + \varOmega }{{1 + \frac{{\gamma_{r2} }}{{\gamma_{1r} }}}}} } \right)} \right] = E\left[ {\ln \left( {1 + \sqrt {\frac{A}{1 + z}} } \right)} \right] \\ & = E\left[ {\ln \left( {1 + U} \right)} \right] = \int \frac{{1 - F_{U} \left( u \right)}}{1 + u}du \\ & = \underbrace {{\mathop \int \limits_{0}^{\infty } \frac{ - \sqrt A }{{\left( {1 + \sqrt {\frac{A}{1 + z}} } \right)\left( {2\sqrt {1 + z} } \right)\left( {1 + z} \right)}}dz}}_{{i_{{C_{1} }} }} \\ & \quad + \underbrace {{\mathop \int \limits_{0}^{\infty } \frac{{\sqrt A {{\bar{\upgamma }}}_{r2} }}{{\left( {z{{\bar{\upgamma }}}_{1r} + {{\bar{\upgamma }}}_{r2} } \right)\left( {1 + \sqrt {\frac{A}{1 + z}} } \right)\left( {2\sqrt {1 + z} } \right)\left( {1 + z} \right)}}dz}}_{{i_{{C_{2} }} }}, \\ \end{aligned}$$

(83)

where the integral expressions $i_{{C_{1} }}$ and $i_{{C_{2} }}$ can be obtained by using the MAPLE software, as follows

$$i_{{C_{1} }} = - \ln \left( {1 + \sqrt A } \right) ,$$

(84)

$$i_{{C_{2} }} = \frac{1}{2}\frac{{{{\bar{\upgamma }}}_{rr} \sqrt A \left( { - \pi \left( {{{\bar{\upgamma }}}_{rr} - 1} \right) + 2\ln \left( 2 \right)({{\bar{\upgamma }}}_{rr} - 1)^{{\frac{3}{2}}} + 2\left( {{{\bar{\upgamma }}}_{rr} - 1} \right)k_{1} + \sqrt {{{\bar{\upgamma }}}_{rr} - 1} \ln \left( {{{\bar{\upgamma }}}_{rr} } \right)} \right)}}{{{{\bar{\upgamma }}}_{rr} ({{\bar{\upgamma }}}_{rr} - 1)^{{\frac{3}{2}}} }}.$$

(85)

Therefore, by substituting Eqs. (84) and (85) into Eq. (83), $I_{R}^{\prime L}$ is obtained as follows

$$\begin{aligned} I_{R}^{\prime L} & = - \ln \left( {1 + \sqrt {1 + \varOmega } } \right) \\ & \quad + \frac{1}{2}\frac{{{{\bar{\upgamma }}}_{rr} \sqrt {1 + \varOmega } \left( { - \pi \left( {{{\bar{\upgamma }}}_{rr} - 1} \right) + 2\ln \left( 2 \right)({{\bar{\upgamma }}}_{rr} - 1)^{{\frac{3}{2}}} + 2\left( {{{\bar{\upgamma }}}_{rr} - 1} \right)k_{1} + \sqrt {{{\bar{\upgamma }}}_{rr} - 1} \ln \left( {{{\bar{\upgamma }}}_{rr} } \right)} \right)}}{{{{\bar{\upgamma }}}_{rr} ({{\bar{\upgamma }}}_{rr} - 1)^{{\frac{3}{2}}} }} . \\ \end{aligned}$$

(86)