Abstract
This paper deals with the phenomenology of magnetic reconnection during reversed-field pinch helical self-organization. Numerical results obtained by solving a three-dimensional nonlinear visco-resistive fluid model to describe the hot current-carrying plasma are summarized. Magnetic reconnection manifests itself during the plasma dynamics, interrupting the persistence of quasi-helical states. The main signatures of magnetic reconnection in reversed-field pinches are discussed: partial conversion of magnetic into kinetic energy, current sheet formation, steepening of plasma current profiles, locking of the angular phases between different Fourier components of the magnetic field. The latter is recognized as the three-dimensional trigger of the reconnection events. Then the paper deals with the temporal scales of the process: low visco-resistive dissipation in the model, corresponding to high plasma current in the experiments, results in longer characteristic time between reconnection events. Furthermore, it is confirmed that the scaling of the reconnection rate is compatible with a modified Sweet–Parker model. A discussion of magnetic reconnection during the 2D simplified tokamak internal kink mode evolution, showing the development of secondary tearing instabilities, is presented and the similarities with RFP evolution are highlighted.












Similar content being viewed by others
Notes
During a solar flare some magnetic energy stored in the sun is released, magnetic field lines change their topology and plasma jets are ejected.
They are called magnetars: magnetic energy dissipation is observed as short-duration bursts of gamma-rays.
Topological changes in the magnetic field are fundamental to sustain the dynamo activity.
The study of the simple model for magnetic reconnection described at the beginning of this section explains that in the process of resistive magnetic reconnection, a component of plasma current density in the same direction of the total magnetic field arises. This leads naturally to the analysis of the “parallel current density”, \(J_{\parallel }\).
In Veranda et al. (2019), the same quantity was computed for a database of tokamak-like simulations displaying sawtoothing activity indicating a lower sensitivity to plasma resistivity (i.e., \(\alpha _{\mathrm{tok}}=0.58\)) on the characteristic time between reconnection events \(\varDelta t\). The role of viscosity instead was more relevant there, \(\beta _{\mathrm{tok}}=-0.26\).
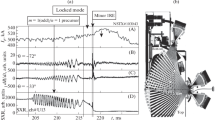
The amplitude of the modes with helicity different from the dominant \(n=7\) was divided by a factor of 2 to highlight the helical structure in a clearer way. The separatrix of the dominant mode is expelled.
The reconnection rate can be defined as the time rate of change of the magnetic flux, across the X point (Park et al. 1984).
References
Aydemir AY, Kim JY, Park BH, Seol J (2015) On resistive magnetohydrodynamic studies of sawtooth oscillations in tokamaks. Phys Plasmas 22(3):032304. https://doi.org/10.1063/1.4914090. https://aip.scitation.org/doi/10.1063/1.4914090
Bhattacharjee A, Huang YM, Yang H, Rogers B (2009) Fast reconnection in high-Lundquist-number plasmas due to the plasmoid instability. Phys Plasmas 16(11):112102. https://doi.org/10.1063/1.3264103
Biskamp D (1986) Magnetic reconnection via current sheets. Phys Fluids 29(5):1520–1531. https://doi.org/10.1063/1.865670. https://aip.scitation.org/doi/abs/10.1063/1.865670
Biskamp D (1993) Magnetic reconnection. Cambridge monographs on plasma physics. Cambridge University Press, Cambridge, pp 127–174. https://doi.org/10.1017/CBO9780511599965.007
Biskamp D (1994) Magnetic reconnection. Phys Rep 237(4):179–247. https://doi.org/10.1016/0370-1573(94)90110-4
Biskamp D (2000) Magnetic reconnection in plasmas. Cambridge monographs on plasma physics. Cambridge University Press, Cambridge. https://doi.org/10.1017/CBO9780511599958
Biskamp D, Schwarz E, Drake JF (1995) Ion-controlled collisionless magnetic reconnection. Phys Rev Lett 75:3850–3853. https://doi.org/10.1103/PhysRevLett.75.3850
Bonfiglio D, Cappello S, Escande DF (2005) Dominant electrostatic nature of the Reversed Field Pinch dynamo. Phys Rev Lett 94:145001. https://doi.org/10.1103/PhysRevLett.94.145001
Bonfiglio D, Chacón L, Cappello S (2010) Nonlinear three-dimensional verification of the SpeCyl and PIXIE3D magnetohydrodynamics codes for fusion plasmas. Phys Plasmas 17:082501. https://doi.org/10.1063/1.3462908
Bonfiglio D, Escande D, Zanca P et al (2011) Necessary criterion for magnetic field reversal in the reversed-field pinch. Nucl Fusion 51:063016
Bonfiglio D, Veranda M, Cappello S, Escande DF, Chacón L (2013) Experimental-like helical self-organization in reversed-field pinch modeling. Phys Rev Lett 111:085002. https://doi.org/10.1103/PhysRevLett.111.085002
Boozer AH (2012) Magnetic reconnection in space. Phys Plasmas 19(9):092902. https://doi.org/10.1063/1.4754715
Boozer AH (2018) Why fast magnetic reconnection is so prevalent. J Plasma Phys 84(1):715840102. https://doi.org/10.1017/S0022377818000028
Borgogno D, Grasso D, Porcelli F, Califano F, Pegoraro F, Farina D (2005) Aspects of three-dimensional magnetic reconnection. Phys Plasmas 12(3):032309. https://doi.org/10.1063/1.1857912
Borgogno D, Perona A, Grasso D (2017) Test-electron analysis of the magnetic reconnection topology. Phys Plasmas 24(12):122303. https://doi.org/10.1063/1.5004613
Cappello S (2004) Bifurcation in the MHD behaviour of a self-organizing system: the reversed-field pinch (RFP). Plasma Phys Controll Fusion 46(12B):B313
Cappello S, Biskamp D (1996) Reconnection processes and scaling laws in reversed-field pinch magnetohydrodynamics. Nucl Fusion 36(5):571
Cappello S, Escande DF (2000) Bifurcation in viscoresistive MHD: the Hartmann number and the reversed-field pinch. Phys Rev Lett 85(18):3838
Cappello S, Bonfiglio D, Escande DF, Guo SC, Alfier A, RLR Team (2008) The reversed-field pinch toward magnetic order: a genuine self-organization. AIP Conf Proc 1069(1):27–39. https://doi.org/10.1063/1.3033714. http://link.aip.org/link/?APC/1069/27/1
Cappello S, Bonfiglio D, Escande D, Guo S, Predebon I, Sattin F, Veranda M, Zanca P, Angioni C, Chacón L, Dong J, Garbet X, Liu S (2011) Equilibrium and transport for quasi-helical reversed-field pinches. Nucl Fusion 51(10):103012. http://stacks.iop.org/0029-5515/51/i=10/a=103012
Caramana E, Nebel R, Schnack D (1983) Nonlinear, single-helicity magnetic reconnection in the reversed-field-pinch. Phys Fluids 26(5):1305
Chacón L (2008) An optimal, parallel, fully implicit Newton–Krylov solver for three-dimensional viscoresistive magnetohydrodynamics. Phys Plasmas 15:056103
Chen F (2016) Introduction to plasma physics and controlled fusion, 3rd edn. Springer International Publishing, Berlin. https://doi.org/10.1007/978-3-319-22309-4
Chen L, Bhattacharjee A, Puhl-Quinn P, Yang H, Bessho N, Imada S, Mühlbachler S, Daly P, Lefebvre B, Khotyaintsev Y, Vaivads A, Fazakerley A, Georgescu E (2008) Observation of energetic electrons within magnetic islands. Nat Phys 4:19. https://doi.org/10.1038/nphys777
Ciaccio G, Veranda M, Bonfiglio D, Cappello S, Spizzo G, Chacón L, White RB (2013) Numerical verification of Orbit and Nemato codes for magnetic topology diagnosis. Phys Plasmas 20(6):062505. https://doi.org/10.1063/1.4811380. http://link.aip.org/link/?PHP/20/062505/1
Comisso L, Bhattacharjee A (2016) On the value of the reconnection rate. J Plasma Phys 82(6):595820601. https://doi.org/10.1017/S002237781600101X
Comisso L, Grasso D (2016) Visco-resistive plasmoid instability. Phys Plasmas 23(3):032111. https://doi.org/10.1063/1.4942940
Cowling T (1934) The magnetic field of sunspots. Mon Not R Astron Soc 94:39
Crocker NA, Fiksel G, Prager SC, Sarff JS (2003) Measurement of the current sheet during magnetic reconnection in a toroidal plasma. Phys Rev Lett 90:035003. https://doi.org/10.1103/PhysRevLett.90.035003
Del Sarto D, Ottaviani M (2017) Secondary fast reconnecting instability in the sawtooth crash. Phys Plasmas 24(1):012102. https://doi.org/10.1063/1.4973328
Delzanno G, Chacón L, Finn J (2008) Electrostatic mode associated with the pinch velocity in reversed-field pinch simulations. Phys Plasmas 15:122102
D’Haeseleer W, Hitchon W, Called J, Shohet J (1991) Flux coordinates and magnetic field structure. Springer, Berlin
Di Giannatale G (2019) Magnetic confinement properties of 3d equilibria for fusion plasmas: non linear MHD modelling and experimental comparisons. PhD thesis, Joint Research Doctorate in Fusion Science and Engineering, URL Presently under a three years embargo
Ebrahimi F, Mirnov VV, Prager SC (2008) Momentum transport from tearing modes with shear flow. Phys Plasmas 15(5):055701. https://doi.org/10.1063/1.2838247
Escande D, Benisti D (1998) Dynamical interpretation of dynamo the RFP. In: Proceedings of the 7th European fusion theory conference, Juelich
Escande D, Martin P, Ortolani S, Buffa A, Franz P, Marrelli L, Martines E, Spizzo G, Cappello S, Murari A, Pasqualotto R, Zanca P (2000a) Quasi-single-helicity reversed-field-pinch plasmas. Phys Rev Lett 85(8):1662
Escande D, Paccagnella R, Cappello S, Marchetto C, D’Angelo F (2000b) Chaos healing by separatrix disappearance in quasi single helicity states of the reversed-field pinch. Phys Rev Lett 85(15):3169
Falessi M, Pegoraro F, Schep T (2015) Lagrangian coherent structures and plasma transport processes. J Plasma Phys 81(5):495810505. https://doi.org/10.1017/S0022377815000690
Fiksel G, Almagri AF, Chapman BE, Mirnov VV, Ren Y, Sarff JS, Terry PW (2009) Mass-dependent ion heating during magnetic reconnection in a laboratory plasma. Phys Rev Lett 103:145002. https://doi.org/10.1103/PhysRevLett.103.145002
Finn J, Chacón L (2005) Volume preserving integrators for solenoidal fields on a grid. Phys Plasmas 12:054503. https://doi.org/10.1063/1.1889156
Finn JM, Kaw PK (1977) Coalescence instability of magnetic islands. Phys Fluids 20(1):72–78. https://doi.org/10.1063/1.861709
Fitzpatrick R (1999) Formation and locking of the slinky mode reversed-field pinches. Phys Plasmas 6(4):1168–1193. https://doi.org/10.1063/1.873361
Furth H, Killeen J, Rosenbluth M (1963) Finite-resistivity instabilities of a sheet pinch. Phys Fluids 6(4):459–484. https://doi.org/10.1063/1.1706761
Garofalo AM, Burrell KH, Eldon D, Grierson BA, Hanson JM, Holland C, Huijsmans GTA, Liu F, Loarte A, Meneghini O, Osborne TH, Paz-Soldan C, Smith SP, Snyder PB, Solomon WM, Turnbull AD, Zeng L (2015) The quiescent h-mode regime for high performance edge localized mode-stable operation in future burning plasmas. Phys Plasmas 22(5):056116. https://doi.org/10.1063/1.4921406
Grasso D, Pegoraro F, Porcelli F, Califano F (1999) Hamiltonian magnetic reconnection. Plasma Phys Controll Fusion 41(12):1497–1515. https://doi.org/10.1088/0741-3335/41/12/306
Grasso D, Borgogno D, Tassi E, Perona A (2020) Asymmetry effects driving secondary instabilities in two-dimensional collisionless magnetic reconnection. Phys Plasmas 27(1):012302. https://doi.org/10.1063/1.5125122
Graves JP, Brunetti D, Chapman IT, Cooper WA, Reimerdes H, Halpern F, Pochelon A, Sauter O, The TCV Team, The MAST Team (2013) Magnetohydrodynamic helical structures in nominally axisymmetric low-shear tokamak plasmas. Plasma Phys Controll Fusion 55(1):014005. http://stacks.iop.org/0741-3335/55/i=1/a=014005
Günter S, Yu Q, Lackner K, Bhattacharjee A, Huang Y (2014) Fast sawtooth reconnection at realistic Lundquist numbers. Plasma Phys Controll Fusion 57(1):014017. https://iopscience.iop.org/article/10.1088/0741-3335/57/1/014017
Haller G (2015) Lagrangian coherent structures. Annu Rev Fluid Mech 47:137–162
Hender T, Gimblett C, Robinson D (1989) Effects of a resistive wall on magnetohydrodynamic instabilities. Nucl Fusion 29(8):1279. http://stacks.iop.org/0029-5515/29/i=8/a=004
Ho YL, Craddock GG (1991) Nonlinear dynamics of field maintenance and quasiperiodic relaxation in reversed-field pinches. Phys Fluids B Plasma Phys 3(3):721–734. https://doi.org/10.1063/1.859868
Holmes JA, Carreras BA, Diamond PH, Lynch VE (1988) Nonlinear dynamics of tearing modes in the reversed field pinch. Phys Fluids 31(5):1166–1179. https://doi.org/10.1063/1.866746
Hurley K, Boggs S, Smith D, Duncan R, Lin R, Zoglauer A, Krucker S, Hurford G, Hudson H, Wigger C, Hajdas W, Thompson C, Mitrofanov I, Sanin A, Boynton W, Fellows C, vonKienlin A, Lichti G, Rau A, Cline T (2005) An exceptionally bright flare from sgr 1806-20 and the origins of short-duration gamma-ray bursts. Nature 434:1098–1103. https://doi.org/10.1038/nature03519
Igochine V, Boom J, Classen I, Dumbrajs O, Günter S, Lackner K, Pereverzev G, Zohm H (2010) Structure and dynamics of sawteeth crashes in ASDEX upgrade. Phys Plasmas 17(12):122506. aip.scitation.org/doi/full/10.1063/1.3529363.
Innes D, Inhester B, Axford W, Wilhelm K (1997) Bi-directional plasma jets produced by magnetic reconnection on the sun. Nature 368:811. https://doi.org/10.1038/386811a0
Jardin SC, Ferraro N, Krebs I (2015) Self-organized stationary states of tokamaks. Phys Rev Lett 115:215001. https://doi.org/10.1103/PhysRevLett.115.215001
King J, Sovinec C, Mirnov V (2012) First-order finite-Larmor-radius fluid modeling of tearing and relaxation in a plasma pinch. Phys Plasmas 19:055905
Kleva RG, Drake JF, Waelbroeck FL (1995) Fast reconnection in high temperature plasmas. Phys Plasmas 2(1):23–34. https://doi.org/10.1063/1.871095
Kryzhanovskyy A, Bonfiglio D, Zuin M, Cappello S, Veranda M (2019) Alfvèn waves in nonlinear 3D MHD modelling of RFP plasmas. In: Proceedings of the 2019 European fusion theory conference, pp 1–10. http://www.eftc2019.ugent.be/docs-upload/Book_of_abstracts_EFTC_2019_final.pdf
Kusano K, Sato T (1986) Simulation study of the self-reversal process in the reversed-field pinch based on a non-linearly driven reconnection model. Nucl Fusion 26(8):1051
Kusano K, Sato T (1990) Simulation study of the self-sustainment mechanism in the reversed-field pinch configuration. Nucl Fusion 30(10):2075
Kusano K, Tamano T, Sato T (1991) MHD simulation of the toroidal phase locking mechanism in a reversed field pinch plasma. Nucl Fusion 31(10):1923–1932. https://doi.org/10.1088/0029-5515/31/10/010
Lapenta G (2008) Self-feeding turbulent magnetic reconnection on macroscopic scales. Phys Rev Lett 100:235001. https://doi.org/10.1103/PhysRevLett.100.235001
Lazarian A, Eyink G, Vishniac E, Kowal G (2015) Turbulent reconnection and its implications. Philos Trans R Soc A Math Phys Eng Sci 373(2041):20140144. https://doi.org/10.1098/rsta.2014.0144
Lazarian A, Eyink GL, Jafari A, Kowal G, Li H, Xu S, Vishniac ET (2020) 3D turbulent reconnection: theory, tests, and astrophysical implications. Phys Plasmas 27(1):012305. https://doi.org/10.1063/1.5110603
Lorenzini R, Martines E, Piovesan P, Terranova D, Zanca P, Zuin M, Alfier A, Bonfiglio D, Bonomo F, Canton A, Cappello S, Carraro L, Cavazzana R, Escande D, Fassina A, Franz P, Gobbin M, Innocente P, Marrelli L, Pasqualotto R, Puiatti M, Spolaore M, Valisa M, Vianello N, Martin P (2009) Self organized helical equilibria as a new paradigm for ohmically heated fusion plasmas. Nat Phys 5:570
Loureiro NF, Uzdensky DA (2015) Magnetic reconnection: from the Sweet–Parker model to stochastic plasmoid chains. Plasma Phys Controll Fusion 58(1):014021. https://doi.org/10.1088/0741-3335/58/1/014021
Loureiro NF, Schekochihin AA, Cowley SC (2007) Instability of current sheets and formation of plasmoid chains. Phys Plasmas 14(10):100703. https://doi.org/10.1063/1.2783986
Magee RM, Den Hartog DJ, Kumar STA, Almagri AF, Chapman BE, Fiksel G, Mirnov VV, Mezonlin ED, Titus JB (2011) Anisotropic ion heating and tail generation during tearing mode magnetic reconnection in a high-temperature plasma. Phys Rev Lett 107:065005. https://doi.org/10.1103/PhysRevLett.107.065005
Malara F, Veltri P, Carbone V (1992) Competition among nonlinear effects in tearing instability saturation. Phys Fluids B Plasma Phys 4(10):3070–3086. https://doi.org/10.1063/1.860477
Malmberg JA, Brzozowski J, Brunsell PR, Cecconello M, Drake JR (2004) Mode- and plasma rotation in a resistive shell reversed-field pinch. Phys Plasmas 11(2):647–658. https://doi.org/10.1063/1.1639016
Marrelli L, Cavazzana R, Bonfiglio D, Gobbin M, Marchiori G, Peruzzo S, Puiatti ME, Spizzo G, Voltolina D, Zanca P, Zuin M, Berton G, Bettini P, Bolzonella T, Canton A, Cappello S, Carraro L, Cordaro L, Dal Bello S, Dalla Palma M, De Masi G, Fassina A, Gnesotto F, Grando L, Innocente P, Lunardon F, Manduchi G, Marcuzzi D, Marconato N, Piovan R, Pomaro N, Rigoni A, Rizzolo A, Scarin P, Siragusa M, Sonato P, Spagnolo S, Spolaore M, Terranova D (2019) Upgrades of the RFX-mod reversed field pinch and expected scenario improvements. Nucl Fusion 59(7):076027. https://doi.org/10.1088/1741-4326/ab1c6a
Martin P, Marrelli L, Spizzo G, Franz P, Piovesan P, Predebon I, Bolzonella T, Cappello S, Cravotta A, Escande D, Frassinetti L, Ortolani S, Paccagnella R, Terranova D, Chapman B, Craig D, Prager S, Sarff J, Brunsell P, Malmberg J, Drake J, Yagi Y, Koguchi H, Hirano Y, White R, Sovinec C, Xiao C, Nebel R, Schnack D (2003) Overview of quasi-single helicity experiments in reversed-field pinches. Nucl Fusion 43(12):1855
Masamune S, Sanpei A, Ikezoe R, Onchi T, Murata K, Oki K, Shimazu H, Yamashita T, Himura H (2007) Characterization of initial low-aspect ratio RFP plasmas in RELAX. J Phys Soc Jpn 76:123501. https://doi.org/10.1143/JPSJ.76.123501
Matthaeus WH, Lamkin SL (1986) Turbulent magnetic reconnection. Phys Fluids 29(8):2513–2534. https://doi.org/10.1063/1.866004
Nicolas T, Lütjens H, Luciani J, Garbet X, Sabot R (2014) Impurity behavior during sawtooth activity in tokamak plasmas. Phys Plasmas 21(1):012507. https://doi.org/10.1063/1.4861859
Onofri M, Malara F, Veltri P (2008) Compressibility effects in the dynamics of the reversed-field pinch. Phys Rev Lett 101:255002. https://doi.org/10.1103/PhysRevLett.101.255002
Ottaviani M, Porcelli F (1993) Nonlinear collisionless magnetic reconnection. Phys Rev Lett 71:3802–3805. https://doi.org/10.1103/PhysRevLett.71.3802
Paccagnella R, Terranova D, Zanca P (2007) Modelling and interpretation of MHD active control experiments in RFX-mod. Nucl Fusion 47(8):990. http://stacks.iop.org/0029-5515/47/i=8/a=033
Park W, Monticello DA, White RB (1984) Reconnection rates of magnetic fields including the effects of viscosity. Phys Fluids 27(1):137–149. https://doi.org/10.1063/1.864502
Parker EN (1955) Hydromagnetic dynamo models. Astrophys J 122:293
Parker EN (1957) Sweet’s mechanism for merging magnetic fields in conducting fluids. J Geophys Res (1896–1977) 62(4):509–520. https://doi.org/10.1029/JZ062i004p00509
Pasqualini D, Koguchi H, Spizzo G, Yagi Y, Hirano Y, Martin P, Sakakita H (2006) Plasma-wall interaction and locked modes in the toroidal pinch experiment TPE-RX reversed-field pinch. J Phys Soc Jpn 75(2):023501. https://doi.org/10.1143/JPSJ.75.023501
Pegoraro F, Bonfiglio D, Cappello S, Giannatale GD, Falessi MV, Grasso D, Veranda M (2019) Coherent magnetic structures in self-organized plasmas. Plasma Phys Controll Fusion 61(4):044003. https://doi.org/10.1088/1361-6587/ab03b5
Peruzzo S, Agostini M, Agostinetti P, Bernardi M, Bettini P, Bolzonella T, Canton A, Carraro L, Cavazzana R, Dal Bello S et al (2017) Design concepts of machine upgrades for the RFX-mod experiment. Fusion Eng Des 123:59–62
Petschek HE (1963) Magnetic field annihilation. In: Hess WN (ed) AAS‐NASA symposium on the physics of solar flares. NASA Special Publication, SP 50
Piovesan P, Craig D, Marrelli L, Cappello S, Martin P (2004) Measurements of the MHD dynamo in the quasi-single-helicity reversed-field pinch. Phys Rev Lett 93:235001. https://doi.org/10.1103/PhysRevLett.93.235001
Piovesan P, Zuin M, Alfier A, Bonfiglio D, Bonomo F, Canton A, Cappello S, Carraro L, Cavazzana R, Escande D, Fassina A, Gobbin M, Lorenzini R, Marrelli L, Martin P, Martines E, Pasqualotto R, Puiatti M, Spolaore M, Valisa M, Vianello N, Zanca P (2009) Magnetic order and confinement improvement in high-current regimes of RFX-mod with MHD feedback control. Nucl Fusion 49:085036
Piovesan P, Bonfiglio D, Cianciosa M, Luce T, Taylor N, Terranova D, Turco F, Wilcox R, Wingen A, Cappello S, Chrystal C, Escande D, Holcomb C, Marrelli L, Paz-Soldan C, Piron L, Predebon I, Zaniol B, DIII-D T, Teams RM (2017) Role of a continuous MHD dynamo in the formation of 3D equilibria in fusion plasmas. Nucl Fusion 57(7):076014. http://stacks.iop.org/0029-5515/57/i=7/a=076014
Puiatti M, Cappello S, Lorenzini R, Martini S, Ortolani S, Paccagnella R, Sattin F, Terranova D, Bolzonella T, Buffa A, Canton A, Carraro L, Escande D, Garzotti L, Innocente P, Marrelli L, Martines E, Scarin P, Spizzo G, Valisa M, Zanca P, Antoni V, Apolloni L, Bagatin M, Baker W, Barana O, Bettella D, Bettini P, Cavazzana R, Cavinato M, Chitarin G, Cravotta A, D’Angelo F, Bello SD, Lorenzi AD, Desideri D, Fiorentin P, Franz P, Frassinetti L, Gaio E, Giudicotti L, Gnesotto F, Grando L, Guo S, Luchetta A, Malesani G, Manduchi G, Marchiori G, Marcuzzi D, Martin P, Masiello A, Milani F, Moresco M, Murari A, Nielsen P, Pasqualotto R, Pégourie B, Peruzzo S, Piovan R, Piovesan P, Pomaro N, Preti G, Regnoli G, Rostagni G, Serianni G, Sonato P, Spada E, Spolaore M, Taliercio C, Telesca G, Toigo V, Vianello N, Zaccaria P, Zaniol B, Zanotto L, Zilli E, Zollino G, Zuin M (2003a) Analysis and modelling of the magnetic and plasma profiles during PPCD experiments in RFX. Nucl Fusion 43(10):1057. http://stacks.iop.org/0029-5515/43/i=10/a=006
Puiatti M, Valisa M, Agostini M, Auriemma F, Bonomo F, Carraro L, Fassina A, Gobbin M, Lorenzini R, Momo B, Scaggion A, Zaniol B, Alfier A, Apolloni L, Baruzzo M, Bolzonella T, Bonfiglio D, Canton A, Cappello S, Cavazzana R, Bello SD, Masi GD, Escande D, Franz P, Gazza E, Guo S, Innocente P, Marchiori G, Marrelli L, Martin P, Martines E, Martini S, Menmuir S, Novello L, Paccagnella R, Piovesan P, Piron L, Predebon I, Ruzzon A, Sattin F, Scarin P, Soppelsa A, Spizzo G, Spagnolo S, Spolaore M, Terranova D, Veranda M, Vianello N, Zanca P, Zanotto L, Zuin M (2011) Internal and external electron transport barriers in the RFX-mod reversed-field pinch. Nucl Fusion 51(7):073038. http://stacks.iop.org/0029-5515/51/i=7/a=073038
Puiatti ME, Valisa M, Mattioli M, Bolzonella T, Bortolon A, Coffey I, Dux R, von Hellermann M, Monier-Garbet P, Nave MFF, Ongena J, Contributors to the EFDA-JET Workprogramme (2003b) Simulation of the time behaviour of impurities in JET Ar-seeded discharges and its relation with sawtoothing and RF heating. Plasma Phys Controll Fusion 45(12):2011–2024. https://doi.org/10.1088/0741-3335/45/12/003
Pustovitov V (2011) Integral torque balance in tokamaks. Nucl Fusion 51(1):013006. http://stacks.iop.org/0029-5515/51/i=1/a=013006
Romanova MM, Lovelace RVE (1992) Magnetic field, reconnection and particle acceleration in extragalactic jets. Astron Astrophys 262:26–36
Rubino G, Borgogno D, Veranda M, Bonfiglio D, Cappello S, Grasso D (2015) Detection of magnetic barriers in a chaotic domain: first application of finite time Lyapunov exponent method to a magnetic confinement configuration. Plasma Phys Controll Fusion 57(8):085004. http://stacks.iop.org/0741-3335/57/i=8/a=085004
Sarff J, Almagri A, Anderson J, Borchardt M, Carmody D, Caspary K, Chapman B, Hartog DD, Duff J, Eilerman S, Falkowski A, Forest C, Goetz J, Holly D, Kim JH, King J, Ko J, Koliner J, Kumar S, Lee J, Liu D, Magee R, McCollam K, McGarry M, Mirnov V, Nornberg M, Nonn P, Oliva S, Parke E, Reusch J, Sauppe J, Seltzman A, Sovinec C, Stephens H, Stone D, Theucks D, Thomas M, Triana J, Terry P, Waksman J, Bergerson W, Brower D, Ding W, Lin L, Demers D, Fimognari P, Titus J, Auriemma F, Cappello S, Franz P, Innocente P, Lorenzini R, Martines E, Momo B, Piovesan P, Puiatti M, Spolaore M, Terranova D, Zanca P, Belykh V, Davydenko V, Deichuli P, Ivanov A, Polosatkin S, Stupishin N, Spong D, Craig D, Harvey R, Cianciosa M, Hanson J (2013) Overview of results from the MST reversed field pinch experiment. Nucl Fusion 53(10):104017. http://stacks.iop.org/0029-5515/53/i=10/a=104017
Scarin P, Agostini M, Spizzo G, Veranda M, Zanca P (2019) Helical plasma-wall interaction in the RFX-mod: effects of high-n mode locking. Nucl Fusion 59(8):086008. https://doi.org/10.1088/1741-4326/ab2071
Scheffel J, Schnack D (2000) Numerical studies of confinement scaling in the conventional reversed field pinch. Nucl Fusion 40(11):1885. http://stacks.iop.org/0029-5515/40/i=11/a=308
Schnack D, Caramana E, Nebel R (1985) Three-dimensional magnetohydrodynamic studies of the reversed-field pinch. Phys Fluids 28(1):321
Seguin FH, Petrasso R, Marmar ES (1983) Effects of internal disruptions on impurity transport in tokamaks. Phys Rev Lett 51:455–458. https://doi.org/10.1103/PhysRevLett.51.455
Sonato P, Chitarin G, Zaccaria P, Gnesotto F, Ortolani S, Buffa A, Bagatin M, Baker W, Dal Bello S, Fiorentin P, Grando L, Marchiori G, Marcuzzi D, Masiello A, Peruzzo S, Pomaro N, Serianni G (2003) Machine modification for active MHD control in RFX. Fusion Eng Des 66:161
Sovinec CR, Finn JM, del Castillo-Negrete D (2001) Formation and sustainment of electrostatically driven spheromaks in the resistive magnetohydrodynamic model. Phys Plasmas 8(2):475–490. https://doi.org/10.1063/1.1335585
Sovinec CR, Gianakon TA, Held ED, Kruger SE, Schnack DD (2003) Nimrod: a computational laboratory for studying nonlinear fusion magnetohydrodynamics. Phys Plasmas 10(5):1727–1732. https://doi.org/10.1063/1.1560920
Spagnolo S, Zuin M, Auriemma F, Cavazzana R, Martines E, Spolaore M, Vianello N (2011) Alfven eigenmodes in the RFX-mod reversed-field pinch plasma. Nucl Fusion. https://doi.org/10.1088/0029-5515/51/8/083038
Spizzo G, Cappello S, Cravotta A, Escande D, Predebon I, Marrelli L, Martin P, White R (2006) Transport barriers inside the reversal surface in the chaotic regime of the reversed-field pinch. Phys Rev Lett 96:025001
Spizzo G, Agostini M, Scarin P, White R, Schmitz O, Spolaore M, Terranova D, Veranda M, Vianello N (2017) Toroidal coupling in the kinetic response to edge magnetic perturbations. Nucl Fusion 57(12):126055. https://doi.org/10.1088/1741-4326/aa8a9c
Stanier A, Daughton W, Le A, Li X, Bird R (2019) Influence of 3D plasmoid dynamics on the transition from collisional to kinetic reconnection. Phys Plasmas 26(7):072121. https://doi.org/10.1063/1.5100737
Tamano T, Bard WD, Chu C, Kondoh Y, La Haye RJ, Lee PS, Saito M, Schaffer MJ, Taylor PL (1987) Observation of a new toroidally localized kink mode and its role in reverse-field-pinch plasmas. Phys Rev Lett 59:1444–1447. https://doi.org/10.1103/PhysRevLett.59.1444
Taylor J (1974) Relaxation of toroidal plasma and generation of reversed magnetic fields. Phys Rev Lett 33(19):1139
Terranova D, Bolzonella T, Cappello S, Innocente P, Marrelli L, Pasqualotto R (2000) Study of the scaling of magnetic fluctuations in the RFX reversed-field pinch. Plasma Phys Controll Fusion 42(7):843. http://stacks.iop.org/0741-3335/42/i=7/a=307
Veranda M, Bonfiglio D, Cappello S, Escande D, Auriemma F, Borgogno D, Chacón L, Fassina A, Franz P, Gobbin M et al (2017) Magnetohydrodynamics modelling successfully predicts new helical states in reversed-field pinch fusion plasmas. Nucl Fusion 57(11):116029. https://doi.org/10.1088/1741-4326/aa7f46
Veranda M, Bonfiglio D, Cappello S, Giannatale GD, Escande DF (2019) Helically self-organized pinches: dynamical regimes and magnetic chaos healing. Nucl Fusion 60(1):016007. https://doi.org/10.1088/1741-4326/ab4863
Wainwright JP, Haines MG, Gimblett CG (1995) Fluctuation-driven mean flows and their effect on the dynamo mechanism in the reversed-field pinch. Plasma Phys Controll Fusion 37(12):1449–1458. https://doi.org/10.1088/0741-3335/37/12/007
Weller A, Cheetham AD, Edwards AW, Gill RD, Gondhalekar A, Granetz RS, Snipes J, Wesson JA (1987) Persistent density perturbations at rational-q surfaces following pellet injection in the Joint European Torus. Phys Rev Lett 59:2303–2306. https://doi.org/10.1103/PhysRevLett.59.2303
White R (2006) The theory of toroidally confined plasmas. Imperial College Press, London
White RB (2014) The theory of toroidally confined plasmas, chap 5, 3rd edn. Imperial College, London, pp 194–201. https://doi.org/10.1142/p916
Yamada M, Kulsrud R, Ji H (2010) Magnetic reconnection. Rev Mod Phys 82:603–664. https://doi.org/10.1103/RevModPhys.82.603
Yi L, Shen B, Pukhov A, Fülöp T (2018) Relativistic magnetic reconnection driven by a laser interacting with a micro-scale plasma slab. Nat Commun 9:1601. https://doi.org/10.1038/386811a0. https://doi.org/10.1038/s41467-018-04065-3
Yu Q, Günter S, Lackner K (2014) Formation of plasmoids during sawtooth crashes. Nucl Fusion 54(7):072005. https://doi.org/10.1088/0029-5515/54/7/072005
Zanca P, Martines E, Bolzonella T, Cappello S, Guo SC, Martin P, Martini S, Ortolani S, Paccagnella R, Terranova D, Viterbo M (2001) Analysis of phase locking of tearing modes in reversed field pinch plasmas. Phys Plasmas 8(2):516–524. https://doi.org/10.1063/1.1339835
Zohm H (2015) Magnetohydrodynamic stability of tokamaks, 1st edn. Wiley, Hoboken
Zuin M, Vianello N, Spolaore M, et al. (2009) Current sheets during spontaneous reconnection in a current-carrying fusion plasma. Plasma Phys Controll Fusion 51(3):035012. http://stacks.iop.org/0741-3335/51/i=3/a=035012
Zuin M, Stevanato L, Martines E, Auriemma F, Momo B, Cavazzana R, Masi GD, Gonzalez W, Lorenzini R, Scarin P, Spagnolo S, Spolaore M, Vianello N, Schneider W, Cester D, Nebbia G, Sajo-Bohus L, Viesti G (2015) Characterization of particle dynamics and magnetic reconnection in the RFX-mod plasmas. In: Proceedings of the 2015 EPS conference. http://ocs.ciemat.es/EPS2015PAP/pdf/P4.158.pdf
Zweibel E, Yamada M (2009) Magnetic reconnection in astrophysical and laboratory plasmas. Annu Rev Astron Astrophys 47(1):291–332. https://doi.org/10.1146/annurev-astro-082708-101726
Acknowledgements
The work originates from the participation to a workshop named “Plasma physics and astrophysics up to 2020 and beyond”, in honor of the 70th birthday of Prof. Pierluigi Veltri. The workshop was held at the Department of Physics at the University of Calabria, Rende, Italy on 7th and 8th October 2019. We thank Prof. Pierluigi Veltri and the local organizing committee for the warm hospitality. The authors would like to acknowledge interesting discussions with M. Valisa about the interplay between tokamak sawtoothing and impurities concentration in tokamaks. The authors would like to thank the two referees for their useful suggestions and advices.
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
This paper is a peer-reviewed version of a contribution at the International Conference “Plasma Physics and Astrophysics up to 2020 and beyond” organized by the Department of Physics of Università della Calabria in honor of Pierluigi Veltri’s 70th birthday and held October 7–8, 2019 at Università della Calabria, Rende (Italy)
Appendices
Appendix 1: On the reconnection rate and possible modeling approaches
In this appendix a long-standing problem in reconnection theory is briefly reviewed, i.e., the fast reconnection ratesFootnote 7 (proportional to the inverse Alfvén time) observed in astrophysical and laboratory systems like in the solar flares and in the tokamak sawtoothing instability. The quest for a solution to this problem helps in categorizing the possible strategies for modeling magnetic reconnection. A resistive diffusion process would give too slow reconnection rates, \(\tau ^{-1} \propto \eta\) (\(\eta\) is the plasma dimensionless resistivity), at low values of resistivity measured in astrophysical or laboratory plasmas. A modeling strategy is to describe magnetic reconnection in the magnetohydrodynamic (MHD) regime, and consider it as a phenomenon localized in the vicinity of the region where magnetic field lines break, while elsewhere magnetic field lines are frozen into the plasma (Parker 1957): Sweet and Parker consider a two-dimensional current sheet and an incompressible inflow of plasma in the reconnection region gives a faster rate \(\tau ^{-1} \propto \eta ^{0.5}\), a result later modified including viscous effects (Park et al. 1984) (the “modified Sweet–Parker scaling” \(\tau ^{-1} \propto \eta ^{0.5}(1+\nu /\eta )^{-0.25}\), with \(\nu\) plasma scalar viscosity, discussed in Sect. 4.4).
Petschek proposed a scenario in which reconnection proceeds at faster rates, with \(\tau ^{-1}\propto 1/\ln {\eta }\) (Petschek 1963), by assuming a shorter reconnection layer and that the plasma inflow was redirected outside the reconnection region by shock waves, converting magnetic energy into ions kinetic energy (see Zweibel and Yamada 2009; Biskamp 2000 for a good description of the Sweet–Parker and Petschek models).
Later, the occurrence of instabilities of the current sheet itself (the so-called plasmoids) has been studied, starting from Furth et al. (1963), Biskamp (1986) and continuing in the next decades (Loureiro et al. 2007; Bhattacharjee et al. 2009; Comisso and Grasso 2016; Günter et al. 2014; Loureiro and Uzdensky 2015; Comisso and Bhattacharjee 2016; Del Sarto and Ottaviani 2017; Stanier et al. 2019) discovering the possibility of reconnection rates independent of resistive effects \(\tau ^{-1} \propto \eta ^0\).
Furthermore, it was also shown that collisionless effects could speed-up the reconnection rate to values compatible with observations (Ottaviani and Porcelli 1993; Biskamp et al. 1995; Grasso et al. 1999; Borgogno et al. 2005, 2017; Grasso et al. 2020). Other MHD effects have also been investigated, in particular the role of turbulence in influencing the current sheet diffusion (Matthaeus and Lamkin 1986; Lapenta 2008), or the study of Hall-MHD (Biskamp et al. 1995) or two-fluid (Kleva et al. 1995) or kinetic effects, in particular when the current sheet transverse dimension gets smaller than length scales related to the ion sound Larmor radius or the ion skin depth (Yamada et al. 2010).
Boozer (2018) claims that the most overlooked feature in modeling fast magnetic reconnection is three-dimensionality. Restricting the evolution to two-dimensional spaces obscures the ideal evolution of magnetic fields lines, and in particular their exponential sensitivity to parallel electric fields which can give Alfvén speed reconnection with elementary magnetohydrodynamics modeling.
Appendix 2: Helical flux function \(\chi\)
The computation of the helical flux function \(\chi (\mathbf {r})\), discussed in Sect. 1 and used in Sect. 3.1, proceeds as follows. Let us consider a magnetic field \(\mathbf {B}(\mathbf {r})\) in a three-dimensional space with a curvilinear coordinate system whose coordinates are labeled as \(u^i=(u^1,u^2,u^3)\). Let us suppose that the system has a symmetry, i.e., \(\partial / \partial u^3=0\). The magnetic field can be written using the vector potential \(\mathbf {A}(\mathbf {r})\) as:
where \(\varepsilon ^{ijk}\) represents the Levi-Civita tensor, J the jacobian of the coordinate transformation, \(A_j\) the covariant component of the vector potential and \(\mathbf {e}_k\) is the covariant basis vector. Imposing the relation \(\mathbf {B} \cdot \nabla \chi =0\) choosing a gauge \(A_1=0\) and remembering that \(\partial / \partial u^3=0\) it is found that
meaning that the equality is satisfied if
In helical geometry the helical flux function is given by the third covariant component of the vector potential, that will be indicated as \(A_{3\mathrm{h}}\). The next step is the determination of the helical flux function in cylindrical geometry, using the transformation law of vector between different geometries. Indicating with \(A_{i\mathrm{h}}\) the covariant components of the vector potential in helical geometry and with \(A_{i\mathrm{c}}\) the covariant components of the vector potential in cylindrical geometry the transformation law can be written as (D’Haeseleer et al. 1991) \(A_{i\mathrm{c}}=A_{i\mathrm{h}}\frac{\partial u^{i\mathrm{h}}}{\partial u^{i\mathrm{c}}}\). The helical geometry is defined by the three coordinates \(u^{i\mathrm{h}}=(\rho ,u,l)\), while the cylindrical one is defined by \(u^{i\mathrm{c}}=(r,\theta ,z)= m\theta +\frac{n}{R_0} z\). The quantity \(\rho\) represents a radial coordinate, u represents a helical angle and the third coordinate l is ignorable in the case of helical symmetry under analysis. The coordinates of the helical geometry can be written in terms of the cylindrical coordinates as \(\rho =r\), \(u = m\theta +\frac{n}{R_0} z\) and \(l = z\). Other choices are possible, in particular for the ignorable coordinate.
This identification allows transforming the helical flux function in helical geometry to cylindrical geometry.
Simple calculations show that \(\chi =A_{3\mathrm{h}}=A_{3\mathrm{c}}-\frac{n}{mR_0}A_{2\mathrm{c}}\), that, in terms of the physical cylindrical components of the vector potential \((\tilde{A}_\mathrm{r},\tilde{A}_{\theta },\tilde{A}_z)\) the helical flux function (multiplied by m) is given by:
Rights and permissions
About this article
Cite this article
Veranda, M., Cappello, S., Bonfiglio, D. et al. Magnetic reconnection in three-dimensional quasi-helical pinches. Rend. Fis. Acc. Lincei 31, 963–984 (2020). https://doi.org/10.1007/s12210-020-00944-4
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s12210-020-00944-4