A Conjecture on the Relationship Between Critical Residual Entropy and Finite Temperature Pseudo-transitions of One-dimensional Models

Abstract

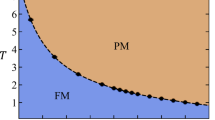
Recently pseudo-critical temperature clues were observed in one-dimensional spin models, such as the Ising-Heisenberg spin models, among others. Here we report a relationship between the zero-temperature phase boundary residual entropy (critical residual entropy) and pseudo-transition. Usually, the residual entropy increases in the phase boundary, which means the system becomes more degenerate at the phase boundary compared with its adjacent states. However, this is not always the case; at zero temperature, there are some phase boundaries where the entropy holds the largest residual entropy of the adjacent states. Therefore, we can propose the following conjecture: If residual entropy at zero temperature is a continuous function at least from the one-sided limit at a critical point, then pseudo-transition evidence will appear at finite temperature near the critical point. We expect that this argument would apply to study more realistic models. Only by analyzing the residual entropy at zero temperature, one could identify a priori whether the system will exhibit the pseudo-transition at finite temperature. To strengthen our conjecture, we use two examples of Ising-Heisenberg models, which exhibit pseudo-transition behavior: one frustrated coupled tetrahedral chain and another unfrustrated diamond chain.

Appendix. Discontinuous Residual Entropy

When CRE is larger than its neighboring residual entropy at zero temperature, it means a discontinuous residual entropy because of $G_{c}>\max \limits (g_{1,0},g_{-1,0})$. Then, to obtain the residual entropy we can use the free energy (4) in the limit $T\rightarrow 0$. Below we get for some particular cases, the residual entropy at zero temperature.

1.1 A.1. Phase Boundary Between States of Sector n = 0 and n = ± 1

Here let us consider the sector n = 1 and n = 0, where the lowest energies are given by $\varepsilon _{_{1,0}}(x)$ and $\varepsilon _{_{0,0}}(x)$. Then the phase boundary occurs when $\varepsilon _{_{1,0}}(x_{c})=\varepsilon _{_{0,0}}(x_{c})=\varepsilon _{c}$ with corresponding degeneracies $g_{1,0}^{c}$ and $g_{-1,0}^{c}$. Eventually, we could have $g_{1,0}^{c}\geqslant g_{1,0}$ and $g_{0,0}^{c}\geqslant g_{0,0}$. The lowest energy ε_− 1,0(x) in sector n = − 1, will be strictly higher than ε_c (ε_− 1,0 > ε_c), what means $w_{-1}/w_{0}\rightarrow 0$ and $w_{-1}/w_{1}\rightarrow 0$ when $T\rightarrow 0$. So the free energy in (4) at sufficiently low temperature becomes

$$ f=-\frac{1}{\beta}\ln\left[\frac{1}{2}\left( g_{1,0}^{c}+\sqrt{(g_{1,0}^{c})^{2}+4(g_{0,0}^{c})^{2}}\right)\mathrm{e}^{-\beta\varepsilon_{c}}\right]. $$

(A.1)

Consequently, the CRE turns in

$$ \mathcal{S}_{c}=\ln\left[\frac{1}{2}\left( g_{1,0}^{c}+\sqrt{(g_{1,0}^{c})^{2}+4(g_{0,0}^{c})^{2}}\right)\right], $$

(A.2)

where the critical degeneracy results in $G_{c}=\frac {1}{2}\left (g_{1,0}^{c}+\sqrt {(g_{1,0}^{c})^{2}+4(g_{0,0}^{c})^{2}}\right )$.

We can observe the critical degeneracy is strictly larger than its adjacent degeneracies: G_c > g_1,0 and G_c > g_0,0. The residual entropy is reported schematically in Fig. 1c. Note that the, residual entropy ${\mathcal {S}}_{a}$ and ${\mathcal {S}}_{b}$ denote in general a residual entropy between adjacent states.

For sector n = − 1 and n = 0, the result of free energy will be equivalent to the previous case. Therefore we can obtain merely by exchanging $\varepsilon _{_{1,0}}(x_{c})\rightarrow \varepsilon _{_{-1,0}}(x_{c})$, in expression (A.2).

1.2 A.2. Phase Boundary Lying in a Single Sector

Assuming that occurs a phase transition between two states with energies ε_1,0(x) and ε_1,1(x), then the critical energy is given by ε_1,0(x_c) = ε_1,1(x_c) = ε_c at T = 0. In general, it is possible that some additional states can coincide at the phase boundary, then the degeneracies can be eventually expressed satisfying the condition $g_{1,0}^{c}\geqslant g_{1,0}$ and $g_{1,1}^{c}\geqslant g_{1,1}$. Therefore, all other energy levels must be higher than ε_c, so when $T\rightarrow 0$, the spectral energy in other sectors can be neglected ($w_{0}/w_{1}\rightarrow 0$ and $w_{-1}/w_{1}\rightarrow 0$). Hence, the free energy in the low-temperature limit is expressed as

$$ \begin{array}{@{}rcl@{}} f&=& -\frac{1}{\beta}\ln\left( w_{1}\right)=-\frac{1}{\beta}\ln\left( g_{1,0}^{c}\mathrm{e}^{-\beta\varepsilon_{c}}+g_{1,1}^{c}\mathrm{e}^{-\beta\varepsilon_{c}}\right) \\ &=& -\frac{1}{\beta}\ln\left[\left( g_{1,0}^{c}+g_{1,1}^{c}\right)\mathrm{e}^{-\beta\varepsilon_{c}}\right]. \end{array} $$

(A.3)

Whereas, the corresponding critical residual entropy, reduces to

$$ \mathcal{S}_{c}=\ln\left( g_{1,0}^{c}+g_{1,1}^{c}\right). $$

(A.4)

Thereby, the critical degeneracy is given by $G_{c}=(g_{1,0}^{c}+g_{1,1}^{c})$.

Once again, the CRE is strictly higher than any residual entropy of adjacent states, because $G_{c}>g_{1,0}^{c}$ and $G_{c}>g_{1,1}^{c}$. A schematic representation of this type of CRE is illustrated in Fig. 1c.

1.3 A.3. Phase Boundary Lying in Three Sectors

When three sectors can constitute the phase boundary, the states with energies $\varepsilon _{_{1,0}}(x)$, $\varepsilon _{_{0,0}}(x)$ and $\varepsilon _{_{-1,0}}(x)$, can coexist for a particular x_c (a critical point). So we must assume $\varepsilon _{_{1,0}}(x_{c})=\varepsilon _{_{0,0}}(x_{c})=\varepsilon _{_{-1,0}}(x_{c})=\varepsilon _{c}$, and the respective degeneracies are $g_{1,0}^{c}$, $g_{0,0}^{c}$ and $g_{-1,0}^{c}$. In this case, the free energy becomes

$$ \begin{array}{@{}rcl@{}} f&=& -\frac{1}{\beta}\ln\left[\frac{1}{2}\left( g_{1,0}^{c}+g_{-1,0}^{c}+\right.\right. \\ &&\left.\left.+\sqrt{(g_{1,0}^{c}-g_{-1,0}^{c})^{2}+4(g_{0,0}^{c})^{2}}\right)\mathrm{e}^{-\beta\varepsilon_{c}}\right]. \end{array} $$

(A.5)

Finally, the CRE reads

$$ \mathcal{S}_{c}=\ln\left[\frac{1}{2}\left( g_{1,0}^{c}+g_{-1,0}^{c}+\sqrt{(g_{1,0}^{c}-g_{-1,0}^{c})^{2}+4(g_{0,0}^{c})^{2}}\right)\right]. $$

(A.6)

Then, the critical degeneracy is $G_{c}=\frac {1}{2}\left (g_{1,0}^{c}+g_{-1,0}^{c}+\sqrt {(g_{1,0}^{c}-g_{-1,0}^{c})^{2}+4(g_{0,0}^{c})^{2}}\right )$.

Similar to the previous cases, the CRE is strictly larger than its adjacent states residual entropies, because $G_{c}>g_{1,0}^{c}$, $G_{c}>g_{0,0}^{c}$ and $G_{c}>g_{-1,0}^{c}$.

In all aforementioned cases, the CRE inevitably exhibits a jump-point discontinuity or a point discontinuity at x_c (see Fig. 1c, d). Because the CRE is strictly larger than its adjacent states residual entropy.