An error bound for the slender body approximation of a thin, rigid fiber sedimenting in Stokes flow

Abstract

We investigate the motion of a thin rigid body in Stokes flow and the corresponding slender body approximation used to model sedimenting fibers. In particular, we derive a rigorous error bound comparing a regularized version of the rigid slender body approximation to the classical PDE for rigid motion in the case of a closed loop with constant radius. Our main tool is the slender body PDE framework established by Mori et al. (Commun Pure Appl Math, 2019. https://doi.org/10.1002/cpa.21872; Arch Ration Mech Anal 235(3):1905–1978, 2020), which we adapt to the rigid setting.

Appendix

Here, we provide proofs for the $L^2(\Gamma _\epsilon )$ trace inequality (Lemma 5) and the higher regularity estimate (Lemma 9).

We first recall the following lemma, which will be used throughout “Appendix.”

Lemma 11

(Sobolev inequality) Let $\varOmega _{\epsilon }=\mathbb {R}^3\backslash \overline{\varSigma _{\epsilon }}$ be as in Sect. 2.1. For any ${\varvec{u}}\in D^{1,2}(\varOmega _{\epsilon })$, we have

$$\begin{aligned} \Vert {\varvec{u}}\Vert _{L^6(\varOmega _{\epsilon })} \le C\Vert \nabla {\varvec{u}}\Vert _{L^2(\varOmega _{\epsilon })}, \end{aligned}$$

(A.1)

where C depends only on $c_\Gamma$ and $\kappa _{\max }$.

The proof of $\epsilon$-independence of C appears in Appendix A.2.4 of [18].

1.1 Proof of Lemma 5

The proof of the $L^2(\Gamma _\epsilon )$ trace inequality follows the same outline as the proof of Lemma 4, contained in Appendix A.2.1 of [18]. In particular, using the $\epsilon$-independent $C^2$-diffeomorphisms $\psi _j$ (defined in Appendix A.2.1, [18]) which map segments of the curved slender body $\varSigma _\epsilon$ to a straight cylinder, it suffices to show the $\sqrt{\epsilon }\left|\log \epsilon \right|$ dependence of the trace constant for a straight cylinder.

Accordingly, let $\mathcal {D}_\rho \subset \mathbb {R}^2$ denote the open disk of radius $\rho$ in $\mathbb {R}^2$, centered at the origin, and, for some $a<\infty$, define the cylindrical surface $\Gamma _{\epsilon ,a}=\partial \mathcal {D}_\epsilon \times [-a,a]$ and the cylindrical shell $\mathcal {C}_{\epsilon ,a}= (\mathcal {D}_1\backslash \overline{\mathcal {D}_\epsilon }) \times [-a,a]$. Consider the function space

$$\begin{aligned} D^{1,2}_\Gamma (\mathcal {C}_{\epsilon ,a})= \big \{ {\varvec{u}}\in D^{1,2}(\mathcal {C}_{\epsilon ,a}) \; : \; {\varvec{u}}\big |_{\partial \mathcal {C}_{\epsilon ,a}\backslash \Gamma _{\epsilon ,a}} = 0 \big \}. \end{aligned}$$

As in the proof of Lemma 4, it suffices to show the $\sqrt{\epsilon }\left|\log \epsilon \right|$ dependence of the $L^2(\Gamma _{\epsilon ,a})$ trace constant for functions belonging to $D^{1,2}_\Gamma (\mathcal {C}_{\epsilon ,a})$.

By estimate (A.4) in [18], any ${\varvec{u}}\in C^1(\mathcal {C}_{\epsilon ,a}) \cap C^0(\overline{\mathcal {C}_{\epsilon ,a}})\cap D^{1,2}_\Gamma (\mathcal {C}_{\epsilon ,a})$ satisfies

$$\begin{aligned} \left|\mathrm{Tr}({\varvec{u}}) \right|^2 \le \left|\log \epsilon \right| \int _\epsilon ^1 \left|\frac{\partial {\varvec{u}}}{\partial \rho } \right|^2 \rho \, {\text {d}}\rho . \end{aligned}$$

Then, noting that the surface element on $\Gamma _{\epsilon ,a}$ is simply $\epsilon$, we have

$$\begin{aligned} \left\Vert \mathrm{Tr}({\varvec{u}}) \right\Vert _{L^2(\Gamma _{\epsilon ,a})}^2&= \int _{-a}^a \int _0^{2\pi } \left|\mathrm{Tr}({\varvec{u}}) \right|^2 \epsilon \, {\text {d}}\theta \, {\text {d}}s \\&\le \epsilon \left|\log \epsilon \right| \int _{-a}^a \int _0^{2\pi }\int _\epsilon ^1 \left|\frac{\partial {\varvec{u}}}{\partial \rho } \right|^2 \rho \, {\text {d}}\rho \, {\text {d}}\theta \, {\text {d}}s \le \epsilon \left|\log \epsilon \right|\left\Vert \nabla {\varvec{u}} \right\Vert _{L^2(\mathcal {C}_{\epsilon ,a})}^2. \end{aligned}$$

The same result for ${\varvec{u}}\in D^{1,2}_\Gamma (\mathcal {C}_{\epsilon ,a})$ follows by density.

1.2 Proof of Lemma 9

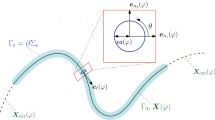
To determine the $\epsilon$-dependence of the constant in (5.3), it suffices to work locally near the slender body surface and show that Lemma 9 holds within an $\epsilon$-independent region about the slender body centerline. We define the region

$$\begin{aligned} \mathcal {O} = \big \{{\varvec{x}}\in \varOmega _{\epsilon } \; : \; {\varvec{x}}= {\varvec{X}}(s) + \rho {\varvec{e}}_{\rho }(s,\theta ), \quad \epsilon< \rho <r_{\max } \big \}, \end{aligned}$$

(A.2)

where $r_{\max }$ is as in Sect. 2.1. Within $\mathcal {O}$, we can use the orthonormal frame (2.2). We will use the notation $\partial _s,\partial _\theta ,\partial _\rho$ to denote derivatives $\partial /\partial s$, $\partial /\partial \theta$, $\partial /\partial \rho$ with respect to the variables $s,\theta ,\rho$, defined with respect to the orthonormal frame. We verify the $\epsilon$-dependence in the bound for $\nabla ^2{\varvec{u}}^{\mathrm{p}}$ and $\nabla p^{\mathrm{p}}$ in two parts: we first show an $L^2$ bound for derivatives $\nabla (\partial _s{\varvec{u}}^{\mathrm{p}})$, $\nabla (\partial _\theta {\varvec{u}}^{\mathrm{p}})$, $\partial _s p^{\mathrm{p}}$, and $\partial _\theta p^{\mathrm{p}}$ in directions tangent to the slender body surface $\Gamma _\epsilon$, and then use these bounds to estimate the derivatives $\nabla (\partial _\rho {\varvec{u}}^{\mathrm{p}})$, $\partial _\rho p^{\mathrm{p}}$ normal to $\Gamma _\epsilon$.

We begin by estimating the tangential derivatives $\nabla (\partial _s{\varvec{u}}^{\mathrm{p}})$ and $\nabla (\partial _\theta {\varvec{u}}^{\mathrm{p}})$. Since the derivatives $\partial _s$ and $\partial _\theta$ with respect to the orthonormal frame (2.2) do not commute with the “straight” differential operators $\nabla$ and $\mathrm{{div}\,}$, we will need to make use of the following commutator bounds.

Proposition 1

(Commutator estimates) For any function ${\varvec{u}}\in D^{1,2}_0(\mathcal {O})$ and for each of the differential operators $D=\mathrm{{div}\,},\, \nabla ,\, \mathcal {E}(\cdot )$, the following commutator estimates hold:

$$\begin{aligned} \left\Vert [D,\partial _\theta ]{\varvec{u}} \right\Vert _{L^2(\mathcal {O})}&\le C\left\Vert \nabla {\varvec{u}} \right\Vert _{L^2(\mathcal {O})}, \quad \left\Vert [D,\partial _s]{\varvec{u}} \right\Vert _{L^2(\mathcal {O})} \le C\left\Vert \nabla {\varvec{u}} \right\Vert _{L^2(\mathcal {O})}, \end{aligned}$$

where the constant C depends only on $c_\Gamma$, $\kappa _{\max }$, and $\xi _{\max }$.

Proof

We begin by denoting

$$\begin{aligned} {\varvec{e}}_\theta (s,\theta )&= -\sin \theta {\varvec{e}}_{n_1}(s) + \cos \theta {\varvec{e}}_{n_2}(s),\\ u_\rho&= {\varvec{u}}\cdot {\varvec{e}}_\rho , \; u_\theta ={\varvec{u}}\cdot {\varvec{e}}_\theta , \; u_s = {\varvec{u}}\cdot {\varvec{e}}_t. \end{aligned}$$

Then, with respect to the orthonormal frame (2.2), the divergence and gradient are given by

$$\begin{aligned} \mathrm{{div}\,}{\varvec{u}}&= \frac{1}{1-\rho \widehat{\kappa }} \bigg ( \frac{1}{\rho } \frac{\partial (\rho (1-\rho \widehat{\kappa })u_\rho )}{\partial \rho } + \frac{1}{\rho }\frac{\partial ((1-\rho \widehat{\kappa })u_\theta )}{\partial \theta } + \frac{\partial u_s}{\partial s} \bigg ), \\ \nabla {\varvec{u}}&= {\varvec{e}}_\rho (s,\theta )\frac{\partial {\varvec{u}}}{\partial \rho }^{\mathrm{T}} + {\varvec{e}}_\theta (s,\theta )\frac{1}{\rho }\frac{\partial {\varvec{u}}}{\partial \theta }^{\mathrm{T}} + {\varvec{e}}_t(s)\frac{1}{1-\rho \widehat{\kappa }} \bigg (\frac{\partial {\varvec{u}}}{\partial s} -\kappa _3 \frac{\partial {\varvec{u}}}{\partial \theta } \bigg )^{\mathrm{T}}, \end{aligned}$$

where

$$\begin{aligned} \widehat{\kappa }(s,\theta ) = \kappa _1(s)\cos \theta + \kappa _2(s)\sin \theta . \end{aligned}$$

(A.3)

Direct computation of the commutators yields

$$\begin{aligned}{}[\mathrm{{div}\,},\partial _\theta ]{\varvec{u}}&= \frac{(\partial _\theta \widehat{\kappa })}{1-\rho \widehat{\kappa }}\bigg ( \rho \,\mathrm{{div}\,}{\varvec{u}}- \frac{1}{\rho }\frac{\partial }{\partial \rho } \big (\rho ^2 u_\rho \big ) - \frac{\partial u_\theta }{\partial \theta } \bigg ) - \frac{(\partial _\theta ^2\widehat{\kappa })}{1-\rho \widehat{\kappa }}u_\theta , \\ [\mathrm{{div}\,},\partial _s]{\varvec{u}}&= \frac{(\partial _s\widehat{\kappa })}{1-\rho \widehat{\kappa }}\bigg ( \rho \,\mathrm{{div}\,}{\varvec{u}}- \frac{1}{\rho }\frac{\partial }{\partial \rho } \big (\rho ^2 u_\rho \big ) - \frac{\partial u_\theta }{\partial \theta } \bigg ) - \frac{(\partial _\theta \partial _s\widehat{\kappa })}{1-\rho \widehat{\kappa }}u_\theta , \\ [\nabla ,\partial _\theta ]{\varvec{u}}&= {\varvec{e}}_\theta \frac{\partial {\varvec{u}}}{\partial \rho }^{\mathrm{T}} - {\varvec{e}}_\rho \frac{1}{\rho }\frac{\partial {\varvec{u}}}{\partial \theta }^{\mathrm{T}} + {\varvec{e}}_t\frac{\rho (\partial _\theta \widehat{\kappa })}{(1-\rho \widehat{\kappa })^2} \bigg (\frac{\partial {\varvec{u}}}{\partial s} -\kappa _3 \frac{\partial {\varvec{u}}}{\partial \theta } \bigg )^{\mathrm{T}}, \\ [\nabla ,\partial _s]{\varvec{u}}&= (\partial _s{\varvec{e}}_\rho )\frac{\partial {\varvec{u}}}{\partial \rho }^{\mathrm{T}} + (\partial _s{\varvec{e}}_\theta )\frac{1}{\rho }\frac{\partial {\varvec{u}}}{\partial \theta }^{\mathrm{T}} + \bigg ({\varvec{e}}_t \frac{\rho (\partial _s\widehat{\kappa })}{1-\rho \widehat{\kappa }}+(\partial _s{\varvec{e}}_t) \bigg )\\&\qquad \frac{1}{1-\rho \widehat{\kappa }} \bigg (\frac{\partial {\varvec{u}}}{\partial s} -\kappa _3 \frac{\partial {\varvec{u}}}{\partial \theta } \bigg )^{\mathrm{T}}. \end{aligned}$$

Using (A.3) and the orthonormal frame ODEs (2.2), we have

$$\begin{aligned} \left|\partial _\theta \widehat{\kappa } \right|&= \left|-\kappa _1\sin \theta + \kappa _2\cos \theta \right|\le \kappa _{\max }, \quad \left|\partial _s \widehat{\kappa } \right| = \left|\kappa _1'\cos \theta +\kappa _2'\sin \theta \right|\\&\le \xi _{\max } + 2(\kappa _{\max }+\pi ), \\ \left|\partial _\theta ^2\widehat{\kappa } \right|&= \left|-\widehat{\kappa } \right|\le \kappa _{\max }, \quad \left|\partial _\theta \partial _s \widehat{\kappa } \right| = \left|-\kappa _1'\sin \theta + \kappa _2'\cos \theta \right|\\&\le \xi _{\max }+ 2(\kappa _{\max }+\pi ), \\ \left|\partial _s {\varvec{e}}_\rho \right|&= \left|-\widehat{\kappa }{\varvec{e}}_t + \kappa _3{\varvec{e}}_\theta \right|\le \kappa _{\max } +\pi , \quad \left|\partial _s{\varvec{e}}_\theta \right| = \left|-(\partial _\theta \widehat{\kappa }){\varvec{e}}_t -\kappa _3{\varvec{e}}_t \right|\\&\le \kappa _{\max } +\pi , \\ \left|\frac{1}{1-\rho \widehat{\kappa }} \right|&\le \frac{1}{1- r_{\max }\kappa _{\max }(\cos \theta +\sin \theta ) } \le \frac{1}{1- \frac{1}{2\kappa _{\max }}\kappa _{\max }\sqrt{2} } \le 4 . \end{aligned}$$

Finally, noting that, by Lemma 11,

$$\begin{aligned} \left\Vert u_\theta \right\Vert _{L^2(\mathcal {O})} \le \left|\mathcal {O} \right|^{1/3} \left\Vert {\varvec{u}} \right\Vert _{L^6(\mathcal {O})} \le C\left\Vert \nabla {\varvec{u}} \right\Vert _{L^2(\mathcal {O})}, \end{aligned}$$

the desired $L^2(\varOmega )$ bounds follow for each of $D=\mathrm{{div}\,},\nabla$. The estimate for the symmetric gradient $\mathcal {E}({\varvec{u}})$ then follows from the gradient commutator bound. $\square$

Now, to derive an estimate for $\nabla (\partial _s{\varvec{u}}^{\mathrm{p}})$, we will make use of Definition 3 with a particular test function ${\varvec{\varphi }}$, which we will construct here. First, we want our test function to be supported only within $\mathcal {O}$. We define a smooth cutoff function

$$\begin{aligned} \psi (\rho ) = {\left\{ \begin{array}{ll} 1, &{} \rho < r_{\max }/4, \\ 0, &{} \rho > r_{\max }/2, \end{array}\right. } \quad \left|\frac{\partial \psi }{\partial \rho } \right| \le C, \end{aligned}$$

(A.4)

where C depends only on $r_{\max }$. Note that $\psi (\rho )$ commutes with both $\partial _\theta$ and $\partial _s$.

We would like to use $\partial _s^2(\psi {\varvec{u}}^{\mathrm{p}})$ as a test function in Definition 3, but it will be more convenient to work with a function which vanishes on $\Gamma _\epsilon$. We therefore construct a correction ${\varvec{g}}\in C^2(\varOmega _\epsilon )$ supported only in $\mathcal {O}$ and satisfying

$$\begin{aligned} {\varvec{g}}\big |_{\Gamma _\epsilon } = (\partial _s {\varvec{u}}^{\mathrm{p}})\big |_{\Gamma _\epsilon } = {\varvec{\omega }}^{\mathrm{p}}\times {\varvec{e}}_t(s), \quad \left\Vert \nabla {\varvec{g}} \right\Vert _{L^2(\mathcal {O})} \le C\left|{\varvec{\omega }} \right|, \end{aligned}$$

(A.5)

where C depends on $c_\Gamma$ and $\kappa _{\max }$. To build ${\varvec{g}}$, we follow a similar construction used in Section 4.1 of [18]. We define

$$\begin{aligned} {\varvec{g}}_0(\rho ,\theta ,s) = {\left\{ \begin{array}{ll} {\varvec{\omega }}^{\mathrm{p}}\times {\varvec{e}}_t(s) &{} \text {if } \rho <4\epsilon , \\ 0 &{} \text {otherwise} \end{array}\right. } \end{aligned}$$

and take

$$\begin{aligned} {\varvec{g}}(\rho ,\theta ,s):= \phi _\epsilon (\rho ){\varvec{g}}_0(\rho ,\theta ,s), \end{aligned}$$

where $\phi _\epsilon (\rho )$ is the smooth cutoff defined in (4.3)–(4.4). Note that ${\varvec{g}}\in C^2$ and is supported within the region

$$\begin{aligned} \mathcal {O}_\epsilon := \big \{ {\varvec{X}}(s) + \rho {\varvec{e}}_\rho (s,\theta ) \; : \; s\in \mathbb {T}, \, \epsilon \le \rho \le 4\epsilon , \, 0\le \theta <2\pi \big \}, \end{aligned}$$

where $\left|\mathcal {O}_\epsilon \right| \le C\epsilon ^2$. Then, using (4.4) and (2.2), we have

$$\begin{aligned} \left\Vert \nabla {\varvec{g}} \right\Vert _{L^2(\mathcal {O})}&\le \sqrt{\left|\mathcal {O}_\epsilon \right|}\left\Vert \nabla {\varvec{g}} \right\Vert _{C(\mathcal {O}_\epsilon )} \\&\le \sqrt{\left|\mathcal {O}_\epsilon \right|}\bigg ( \left\Vert \frac{\partial \phi _\epsilon }{\partial \rho } \right\Vert _{C(\mathcal {O}_\epsilon )}\left\Vert {\varvec{g}}_0 \right\Vert _{C(\mathcal {O}_\epsilon )}+ \left\Vert \frac{1}{1-\rho \widehat{\kappa }}\frac{\partial {\varvec{g}}_0}{\partial s} \right\Vert _{C(\mathcal {O}_\epsilon )} \bigg ) \le C\left|{\varvec{\omega }}^{\mathrm{p}} \right|. \end{aligned}$$

Now, we could just use $\partial _s(\partial _s(\psi {\varvec{u}}^{\mathrm{p}}) -{\varvec{g}})$ as a test function in Definition 3, but it will actually be useful to include a second correction term in the following way. We consider ${\varvec{z}}\in D^{1,2}_0(\mathcal {O})$ satisfying

$$\begin{aligned} \begin{aligned} \mathrm{{div}\,}{\varvec{z}}&= \mathrm{{div}\,}(\psi \partial _s{\varvec{u}}^{\mathrm{p}} -{\varvec{g}}) \quad \text {in } \mathcal {O}, \\ \left\Vert \nabla {\varvec{z}} \right\Vert _{L^2(\mathcal {O})}&\le C\left\Vert \mathrm{{div}\,}(\psi \partial _s{\varvec{u}}^{\mathrm{p}} -{\varvec{g}}) \right\Vert _{L^2(\mathcal {O})} \end{aligned} \end{aligned}$$

(A.6)

for C depending only on $c_\Gamma$ and $\kappa _{\max }$. We know that such a ${\varvec{z}}$ exists due to [6], Section III.3, and the constant C is independence of $\epsilon$ due to Appendix A.2.5 of [18]. Furthermore, since $\mathrm{{div}\,}{\varvec{u}}^{\mathrm{p}}=0$, by Proposition 1 we have

$$\begin{aligned} \left\Vert \mathrm{{div}\,}(\psi \partial _s{\varvec{u}}^{\mathrm{p}}-{\varvec{g}}) \right\Vert _{L^2(\mathcal {O})}&\le \left\Vert \mathrm{{div}\,}(\partial _\theta {\varvec{u}}^{\mathrm{p}}) \right\Vert _{L^2(\mathcal {O})}+ C\left\Vert \partial _\theta {\varvec{u}}^{\mathrm{p}} \right\Vert _{L^2(\mathcal {O})}+\left\Vert \nabla {\varvec{g}} \right\Vert _{L^2(\mathcal {O})} \\&\le \left\Vert [\mathrm{{div}\,},\partial _\theta ]{\varvec{u}}^{\mathrm{p}} \right\Vert _{L^2(\mathcal {O})} + C\left\Vert \partial _\theta {\varvec{u}}^{\mathrm{p}} \right\Vert _{L^2(\mathcal {O})} + C\left|{\varvec{\omega }}^{\mathrm{p}} \right| \\&\le C\left\Vert \nabla {\varvec{u}}^{\mathrm{p}} \right\Vert _{L^2(\mathcal {O})} + C\left|{\varvec{\omega }}^{\mathrm{p}} \right|. \end{aligned}$$

Here, we have also used that $\left\Vert \partial _\theta {\varvec{u}}^{\mathrm{p}} \right\Vert _{L^2(\mathcal {O})} \le \left\Vert \rho \nabla {\varvec{u}}^{\mathrm{p}} \right\Vert _{L^2(\mathcal {O})} \le r_{\max }\left\Vert \nabla {\varvec{u}}^{\mathrm{p}} \right\Vert _{L^2(\mathcal {O})}$. In particular, ${\varvec{z}}$ satisfying (A.6) also satisfies

$$\begin{aligned} \left\Vert \nabla {\varvec{z}} \right\Vert _{L^2(\mathcal {O})} \le C\left\Vert \nabla {\varvec{u}}^{\mathrm{p}} \right\Vert _{L^2(\mathcal {O})} + C\left|{\varvec{\omega }}^{\mathrm{p}} \right|. \end{aligned}$$

(A.7)

Using extension by zero to consider ${\varvec{z}}$ as a function over all $\varOmega _\epsilon$, we can now construct our desired test function for use in Definition 3. In particular, we will use the function $\partial _s(\partial _s(\psi {\varvec{u}}^{\mathrm{p}})-{\varvec{g}}-{\varvec{z}})$ in place of ${\varvec{\varphi }}$ in Definition 3. Note that by definition of ${\varvec{z}}$, this function may only belong to $L^2(\varOmega _\epsilon )$. In this case, we can make sense of the following integration-by-parts argument using finite differences rather than full derivatives (see [2], Section III.2.7 for construction of finite difference operators along a curved boundary). Thus, we really only need $\partial _s(\psi {\varvec{u}}^{\mathrm{p}})-{\varvec{g}}-{\varvec{z}}\in D^{1,2}(\varOmega _\epsilon )$ to make sense of the following result. Note that in integrating by parts, we will also need to make use of the fact that, for $i=s,\theta$,

$$\begin{aligned} \partial _i ({\text {d}}{\varvec{x}}) = -\frac{\rho \partial _i\widehat{\kappa }}{1-\rho \widehat{\kappa }} {\text {d}}{\varvec{x}}:= \mathcal {J}_i \, {\text {d}}{\varvec{x}}, \quad \left|\mathcal {J}_i \right| \le C; \quad i=s,\theta , \end{aligned}$$

(A.8)

where C depends on $c_\Gamma$, $\kappa _{\max }$, and $\xi _{\max }$.

Then, using $\partial _s(\partial _s(\psi {\varvec{u}}^{\mathrm{p}})-{\varvec{g}}-{\varvec{z}})$ in Definition 3, we have

$$\begin{aligned} 0&= \int _{\mathcal {O}} \bigg (2\mathcal {E}({\varvec{u}}^{\mathrm{p}}): \mathcal {E}\big (\partial _s(\partial _s(\psi {\varvec{u}}^{\mathrm{p}})-{\varvec{g}}-{\varvec{z}}) \big ) - p^{\mathrm{p}} \,\mathrm{{div}\,}(\partial _s(\partial _s(\psi {\varvec{u}}^{\mathrm{p}})-{\varvec{g}}-{\varvec{z}})) \bigg ) \, {\text {d}}{\varvec{x}}\\&= \int _{\mathcal {O}} 2\mathcal {E}({\varvec{u}}^{\mathrm{p}}): \partial _s\mathcal {E}(\partial _s(\psi {\varvec{u}}^{\mathrm{p}})-{\varvec{g}}-{\varvec{z}}) \, {\text {d}}{\varvec{x}}+ \int _{\mathcal {O}} 2\mathcal {E}({\varvec{u}}^{\mathrm{p}}): [\mathcal {E}(\cdot ),\partial _s](\partial _s(\psi {\varvec{u}}^{\mathrm{p}})-{\varvec{g}}-{\varvec{z}}) \, {\text {d}}{\varvec{x}}\\&\qquad - \int _{\mathcal {O}} p^{\mathrm{p}} \,\partial _s(\mathrm{{div}\,}(\partial _s(\psi {\varvec{u}}^{\mathrm{p}})-{\varvec{g}}-{\varvec{z}})) \, {\text {d}}{\varvec{x}}- \int _{\mathcal {O}} p^{\mathrm{p}} \,[\mathrm{{div}\,},\partial _s](\partial _s(\psi {\varvec{u}}^{\mathrm{p}})- {\varvec{g}}-{\varvec{z}}) \, {\text {d}}{\varvec{x}}\\&= -\int _{\mathcal {O}} 2\partial _s\mathcal {E}({\varvec{u}}^{\mathrm{p}}): \mathcal {E}(\partial _s(\psi {\varvec{u}}^{\mathrm{p}})-{\varvec{g}}-{\varvec{z}}) \, {\text {d}}{\varvec{x}}-\int _{\mathcal {O}} 2\mathcal {E}({\varvec{u}}^{\mathrm{p}}): \mathcal {E}(\partial _s(\psi {\varvec{u}}^{\mathrm{p}})-{\varvec{g}}-{\varvec{z}}) \, \mathcal {J}_s \, {\text {d}}{\varvec{x}}\\&\qquad + \int _{\mathcal {O}} 2\mathcal {E}({\varvec{u}}^{\mathrm{p}}): [\mathcal {E}(\cdot ),\partial _s](\partial _s(\psi {\varvec{u}}^{\mathrm{p}})-{\varvec{g}}-{\varvec{z}}) \, {\text {d}}{\varvec{x}}- \int _{\mathcal {O}} p^{\mathrm{p}} \,[\mathrm{{div}\,},\partial _s](\partial _s(\psi {\varvec{u}}^{\mathrm{p}})- {\varvec{g}}-{\varvec{z}}) \, {\text {d}}{\varvec{x}}\\&= -\int _{\mathcal {O}} 2\mathcal {E}(\partial _s{\varvec{u}}^{\mathrm{p}}):\mathcal {E}(\partial _s(\psi {\varvec{u}}^{\mathrm{p}})-{\varvec{g}}-{\varvec{z}}) \, {\text {d}}{\varvec{x}}- \int _{\mathcal {O}} 2\mathcal {E}({\varvec{u}}^{\mathrm{p}}): \mathcal {E}(\partial _s(\psi {\varvec{u}}^{\mathrm{p}})-{\varvec{g}}-{\varvec{z}}) \, \mathcal {J}_s \, {\text {d}}{\varvec{x}}\\&\qquad +\int _{\mathcal {O}} 2[\mathcal {E}(\cdot ),\partial _s]{\varvec{u}}^{\mathrm{p}}: \mathcal {E}(\partial _s(\psi {\varvec{u}}^{\mathrm{p}})- {\varvec{g}}-{\varvec{z}}) \, {\text {d}}{\varvec{x}}\\&\qquad + \int _{\mathcal {O}} 2\mathcal {E}({\varvec{u}}^{\mathrm{p}}): [\mathcal {E}(\cdot ),\partial _s](\partial _s(\psi {\varvec{u}}^{\mathrm{p}})-{\varvec{g}}-{\varvec{z}}) \, {\text {d}}{\varvec{x}}\\&\qquad - \int _{\mathcal {O}} p^{\mathrm{p}} \,[\mathrm{{div}\,},\partial _s](\partial _s(\psi {\varvec{u}}^{\mathrm{p}})- {\varvec{g}}-{\varvec{z}}) \, {\text {d}}{\varvec{x}}. \end{aligned}$$

Note that the first integral in the third line vanishes due to the definition of ${\varvec{z}}$. In this way, we can avoid having to deal with a $\partial _s p^{\mathrm{p}}$ term in the resulting estimate.

Then, using Proposition 1, estimates (A.7) and (A.5), and Lemma 6, we have

$$\begin{aligned}&\left\Vert \mathcal {E}(\partial _s{\varvec{u}}^{\mathrm{p}}) \right\Vert _{L^2(\mathcal {O})}^2 \\&\quad \le C\left\Vert \mathcal {E}(\partial _s{\varvec{u}}^{\mathrm{p}}) \right\Vert _{L^2(\mathcal {O})} \big ( \left\Vert \partial _s {\varvec{u}}^{\mathrm{p}} \right\Vert _{L^2(\mathcal {O})} + \left\Vert \mathcal {E}({\varvec{z}}) \right\Vert _{L^2(\mathcal {O})} + \left\Vert \mathcal {E}({\varvec{g}}) \right\Vert _{L^2(\mathcal {O})} \big ) \\&\qquad + C\left\Vert \mathcal {E}({\varvec{u}}^{\mathrm{p}}) \right\Vert _{L^2(\mathcal {O})} \big (\left\Vert \mathcal {E}(\psi \partial _s{\varvec{u}}^{\mathrm{p}}) \right\Vert _{L^2(\mathcal {O})} +\left\Vert \mathcal {E}({\varvec{g}}) \right\Vert _{L^2(\mathcal {O})} +\left\Vert \mathcal {E}({\varvec{z}}) \right\Vert _{L^2(\mathcal {O})} \big ) \\&\qquad + 2\left\Vert [\mathcal {E}(\cdot ),\partial _s]{\varvec{u}}^{\mathrm{p}} \right\Vert _{L^2(\mathcal {O})}\big ( \left\Vert \mathcal {E}(\psi \partial _s{\varvec{u}}^{\mathrm{p}}) \right\Vert _{L^2(\mathcal {O})} + \left\Vert \mathcal {E}({\varvec{z}}) \right\Vert _{L^2(\mathcal {O})} + \left\Vert \mathcal {E}({\varvec{g}}) \right\Vert _{L^2(\mathcal {O})} \big ) \\&\qquad +2\left\Vert \mathcal {E}({\varvec{u}}^{\mathrm{p}}) \right\Vert _{L^2(\mathcal {O})} \big ( \left\Vert [\mathcal {E}(\cdot ),\partial _s](\psi \partial _s{\varvec{u}}^{\mathrm{p}}) \right\Vert _{L^2(\mathcal {O})} + \left\Vert [\mathcal {E}(\cdot ),\partial _s]({\varvec{z}}) \right\Vert _{L^2(\mathcal {O})} \\&\qquad + \left\Vert [\mathcal {E}(\cdot ),\partial _s]({\varvec{g}}) \right\Vert _{L^2(\mathcal {O})} \big ) \\&\qquad + \left\Vert p^{\mathrm{p}} \right\Vert _{L^2(\mathcal {O})} \big ( \left\Vert [\mathrm{{div}\,},\partial _s](\psi \partial _s{\varvec{u}}^{\mathrm{p}}) \right\Vert _{L^2(\mathcal {O})} + \left\Vert [\mathrm{{div}\,},\partial _s]({\varvec{z}}) \right\Vert _{L^2(\mathcal {O})} +\left\Vert [\mathrm{{div}\,},\partial _s]({\varvec{g}}) \right\Vert _{L^2(\mathcal {O})} \big ) \\&\quad \le C(\left\Vert \mathcal {E}(\partial _s{\varvec{u}}^{\mathrm{p}}) \right\Vert _{L^2(\mathcal {O})} + \left\Vert \nabla {\varvec{u}}^{\mathrm{p}} \right\Vert _{L^2(\mathcal {O})} +\left|{\varvec{\omega }} \right|)\big (\left\Vert \nabla {\varvec{u}}^{\mathrm{p}} \right\Vert _{L^2(\mathcal {O})}+\left\Vert p^{\mathrm{p}} \right\Vert _{L^2(\mathcal {O})} +\left|{\varvec{\omega }}^{\mathrm{p}} \right| \big ) \\&\quad \le \delta \left\Vert \mathcal {E}(\partial _s{\varvec{u}}^{\mathrm{p}}) \right\Vert _{L^2(\mathcal {O})}^2 + C(\delta ) \big (\left\Vert \nabla {\varvec{u}}^{\mathrm{p}} \right\Vert _{L^2(\mathcal {O})}^2+\left\Vert p^{\mathrm{p}} \right\Vert _{L^2(\mathcal {O})}^2 + \left|{\varvec{\omega }}^{\mathrm{p}} \right|^2\big ) \end{aligned}$$

for any $0<\delta \in \mathbb {R}$, by Young’s inequality. Taking $\delta =\frac{1}{2}$ and using Lemma 6, we obtain

$$\begin{aligned} \begin{aligned} \left\Vert \nabla (\partial _s{\varvec{u}}^{\mathrm{p}}) \right\Vert _{L^2(\mathcal {O})}&\le \left\Vert \mathcal {E}(\partial _s{\varvec{u}}^{\mathrm{p}}) \right\Vert _{L^2(\mathcal {O})} \le C \big (\left\Vert \nabla {\varvec{u}}^{\mathrm{p}} \right\Vert _{L^2(\mathcal {O})}+\left\Vert p^{\mathrm{p}} \right\Vert _{L^2(\mathcal {O})} + \left|{\varvec{\omega }}^{\mathrm{p}} \right| \big ) \\&\le C\left|\log \epsilon \right|^{1/2} \big (\left\Vert \nabla {\varvec{u}}^{\mathrm{p}} \right\Vert _{L^2(\varOmega _\epsilon )}+\left\Vert p^{\mathrm{p}} \right\Vert _{L^2(\varOmega _\epsilon )} \big ), \end{aligned} \end{aligned}$$

(A.9)

where we have used Corollary 1 to bound $\left|{\varvec{\omega }}^{\mathrm{p}} \right|$. Here C depends only on $c_\Gamma$, $\kappa _{\max }$, and $\xi _{\max }$.

We may estimate $\partial _\theta {\varvec{u}}^{\mathrm{p}}$ in a similar way. In fact, the construction of the analogous test function is simpler since $(\partial _\theta {\varvec{u}}^{\mathrm{p}})\big |_{\Gamma _\epsilon } = \partial _\theta ({\varvec{v}}+{\varvec{\omega }}\times {\varvec{X}}(s))=0$ and thus we do not need to correct for a nonzero boundary value. Following the same steps used to estimate $\partial _s{\varvec{u}}^{\mathrm{p}}$, we obtain

$$\begin{aligned} \left\Vert \nabla (\partial _\theta {\varvec{u}}^{\mathrm{p}}) \right\Vert _{L^2(\mathcal {O})} \le C \big (\left\Vert \nabla {\varvec{u}}^{\mathrm{p}} \right\Vert _{L^2(\varOmega _\epsilon )}+\left\Vert p^{\mathrm{p}} \right\Vert _{L^2(\varOmega _\epsilon )} \big ), \end{aligned}$$

(A.10)

where C depends only on $c_\Gamma$, $\kappa _{\max }$, and $\xi _{\max }$.

In addition to estimates (A.9) and (A.10), we need bounds for the tangential derivatives $\partial _s p^{\mathrm{p}}$ and $\partial _\theta p^{\mathrm{p}}$ of the pressure. We begin by estimating $\partial _s p^{\mathrm{p}}$; the bound for $\partial _\theta p^{\mathrm{p}}$ is similar. Since we already know that $\partial _s p^{\mathrm{p}}\in L^2(\varOmega _\epsilon )$, we may consider $\widetilde{{\varvec{z}}}\in D^{1,2}_0(\mathcal {O})$ satisfying

$$\begin{aligned} \begin{aligned} \mathrm{{div}\,}\widetilde{{\varvec{z}}}&= \psi \partial _s p^{\mathrm{p}} \quad \text {in }\mathcal {O}, \\ \left\Vert \nabla \widetilde{{\varvec{z}}} \right\Vert _{L^2(\mathcal {O})}&\le C\left\Vert \psi \partial _s p^{\mathrm{p}} \right\Vert _{L^2(\mathcal {O})}, \end{aligned} \end{aligned}$$

(A.11)

where $\psi$ is as in (A.4). Again, we know that such a $\widetilde{{\varvec{z}}}$ exists due to [6], Section III.3 and [18], Appendix A.2.5.

Using $\partial _s\widetilde{{\varvec{z}}}$ as a test function in Definition 3 (again, we can make sense of the following computation using finite differences, and thus only require $\widetilde{{\varvec{z}}}\in D^{1,2}(\mathcal {O})$), we have

$$\begin{aligned} 0&= \int _{\mathcal {O}} \bigg (2\mathcal {E}({\varvec{u}}^{\mathrm{p}}): \mathcal {E}(\partial _s \widetilde{{\varvec{z}}}) - p^{\mathrm{p}}\, \mathrm{{div}\,}(\partial _s\widetilde{{\varvec{z}}})\bigg ) \, {\text {d}}{\varvec{x}}= \int _{\mathcal {O}}2\mathcal {E}({\varvec{u}}^{\mathrm{p}}): \partial _s\mathcal {E}(\widetilde{{\varvec{z}}}) \, {\text {d}}{\varvec{x}}\\&\quad + \int _{\mathcal {O}}2\mathcal {E}({\varvec{u}}^{\mathrm{p}}): [\mathcal {E}(\cdot ),\partial _s]\widetilde{{\varvec{z}}}\, {\text {d}}{\varvec{x}}- \int _{\mathcal {O}} p^{\mathrm{p}} \,\partial _s\mathrm{{div}\,}\widetilde{{\varvec{z}}}\, {\text {d}}{\varvec{x}}- \int _{\mathcal {O}} p^{\mathrm{p}} \, [\mathrm{{div}\,},\partial _s]\widetilde{{\varvec{z}}}\, {\text {d}}{\varvec{x}}\\&= -\int _{\mathcal {O}}2\partial _s\mathcal {E}({\varvec{u}}^{\mathrm{p}}): \mathcal {E}(\widetilde{{\varvec{z}}}) \, {\text {d}}{\varvec{x}}- \int _{\mathcal {O}}2\mathcal {E}({\varvec{u}}^{\mathrm{p}}): \mathcal {E}(\widetilde{{\varvec{z}}}) \, \mathcal {J}_s \, {\text {d}}{\varvec{x}}+ \int _{\mathcal {O}}2\mathcal {E}({\varvec{u}}^{\mathrm{p}}): [\mathcal {E}(\cdot ),\partial _s]\widetilde{{\varvec{z}}}\, {\text {d}}{\varvec{x}}\\&\quad - \int _{\mathcal {O}} p^{\mathrm{p}} \, [\mathrm{{div}\,},\partial _s]\widetilde{{\varvec{z}}}\, {\text {d}}{\varvec{x}}+ \int _{\mathcal {O}}(\partial _s p)\mathrm{{div}\,}\widetilde{{\varvec{z}}}\, {\text {d}}{\varvec{x}}+ \int _{\mathcal {O}} p^{\mathrm{p}} \,\mathrm{{div}\,}\widetilde{{\varvec{z}}}\, \mathcal {J}_s \, {\text {d}}{\varvec{x}}\\&= \int _{\mathcal {O}}\psi (\partial _s p)^2 \, {\text {d}}{\varvec{x}}-\int _{\mathcal {O}}2\partial _s\mathcal {E}({\varvec{u}}^{\mathrm{p}}): \mathcal {E}(\widetilde{{\varvec{z}}}) \, {\text {d}}{\varvec{x}}- \int _{\mathcal {O}}2\mathcal {E}({\varvec{u}}^{\mathrm{p}}): \mathcal {E}(\widetilde{{\varvec{z}}}) \, \mathcal {J}_s \, {\text {d}}{\varvec{x}}\\&\quad + \int _{\mathcal {O}}2\mathcal {E}({\varvec{u}}^{\mathrm{p}}): [\mathcal {E}(\cdot ),\partial _s]\widetilde{{\varvec{z}}}\, {\text {d}}{\varvec{x}}- \int _{\mathcal {O}} p^{\mathrm{p}} \, [\mathrm{{div}\,},\partial _s]\widetilde{{\varvec{z}}}\, {\text {d}}{\varvec{x}}+ \int _{\mathcal {O}} p^{\mathrm{p}} \,\mathrm{{div}\,}\widetilde{{\varvec{z}}}\, \mathcal {J}_s \, {\text {d}}{\varvec{x}}, \end{aligned}$$

where $\mathcal {J}_s \, {\text {d}}{\varvec{x}}$ is as in (A.8) and we have used (A.11). Then, using that $\psi ^2\le \psi$, we have

$$\begin{aligned} \left\Vert \psi \partial _s p^{\mathrm{p}} \right\Vert _{L^2(\mathcal {O})}^2&\le 2\left\Vert \mathcal {E}(\partial _s{\varvec{u}}^{\mathrm{p}}) \right\Vert _{L^2(\mathcal {O})} \left\Vert \mathcal {E}(\widetilde{{\varvec{z}}}) \right\Vert _{L^2(\mathcal {O})} + 2\left\Vert [\mathcal {E}(\cdot ),\partial _s]{\varvec{u}}^{\mathrm{p}} \right\Vert _{L^2(\mathcal {O})} \left\Vert \mathcal {E}(\widetilde{{\varvec{z}}}) \right\Vert _{L^2(\mathcal {O})} \\&\quad + C\left\Vert \mathcal {E}({\varvec{u}}^{\mathrm{p}}) \right\Vert _{L^2(\mathcal {O})} \left\Vert \mathcal {E}(\widetilde{{\varvec{z}}}) \right\Vert _{L^2(\mathcal {O})} + 2\left\Vert \mathcal {E}({\varvec{u}}^{\mathrm{p}}) \right\Vert _{L^2(\mathcal {O})}\left\Vert [\mathcal {E}(\cdot ),\partial _s]\widetilde{{\varvec{z}}} \right\Vert _{L^2(\mathcal {O})} \\&\quad +\left\Vert p^{\mathrm{p}} \right\Vert _{L^2(\mathcal {O})} \left\Vert [\mathrm{{div}\,},\partial _s]\widetilde{{\varvec{z}}} \right\Vert _{L^2(\mathcal {O})} + C\left\Vert p^{\mathrm{p}} \right\Vert _{L^2(\mathcal {O})} \left\Vert \mathrm{{div}\,}\widetilde{{\varvec{z}}} \right\Vert _{L^2(\mathcal {O})}\\&\le C\big (\left\Vert \nabla (\partial _s{\varvec{u}}^{\mathrm{p}}) \right\Vert _{L^2(\mathcal {O})} + \left\Vert \nabla {\varvec{u}}^{\mathrm{p}} \right\Vert _{L^2(\mathcal {O})} +\left\Vert p^{\mathrm{p}} \right\Vert _{L^2(\mathcal {O})} \big ) \left\Vert \psi \partial _s p^{\mathrm{p}} \right\Vert _{L^2(\mathcal {O})}\\&\le \delta \left\Vert \psi \partial _s p^{\mathrm{p}} \right\Vert _{L^2(\mathcal {O})}^2 + C(\delta )\big (\left\Vert \nabla (\partial _s{\varvec{u}}^{\mathrm{p}}) \right\Vert _{L^2(\mathcal {O})}^2 + \left\Vert \nabla {\varvec{u}}^{\mathrm{p}} \right\Vert _{L^2(\mathcal {O})}^2 +\left\Vert p^{\mathrm{p}} \right\Vert _{L^2(\mathcal {O})}^2 \big ) \end{aligned}$$

for $0<\delta \in \mathbb {R}$. Here, we have used (A.8), (A.11), Proposition 1, and Young’s inequality. Taking $\delta =\frac{1}{2}$ and using (A.9), we obtain

$$\begin{aligned} \left\Vert \psi \partial _s p^{\mathrm{p}} \right\Vert _{L^2(\mathcal {O})} \le C\left|\log \epsilon \right|^{1/2}\big ( \left\Vert \nabla {\varvec{u}}^{\mathrm{p}} \right\Vert _{L^2(\varOmega _\epsilon )} +\left\Vert p^{\mathrm{p}} \right\Vert _{L^2(\varOmega _\epsilon )} \big ). \end{aligned}$$

Then, using (A.4), within the region

$$\begin{aligned} \mathcal {O}' = \bigg \{{\varvec{x}}\in \varOmega _{\epsilon } \; : \; {\varvec{x}}= {\varvec{X}}(s) + \rho {\varvec{e}}_{\rho }(s,\theta ), \quad \epsilon< \rho <\frac{r_{\max }}{4} \bigg \}, \end{aligned}$$

we have

$$\begin{aligned} \left\Vert \partial _s p^{\mathrm{p}} \right\Vert _{L^2(\mathcal {O}')} \le C\left|\log \epsilon \right|^{1/2}\big ( \left\Vert \nabla {\varvec{u}}^{\mathrm{p}} \right\Vert _{L^2(\varOmega _\epsilon )} +\left\Vert p^{\mathrm{p}} \right\Vert _{L^2(\varOmega _\epsilon )} \big ) \end{aligned}$$

(A.12)

for C depending only on $c_\Gamma$, $\kappa _{\max }$, and $\xi _{\max }$.

We can similarly use (A.10) to show

$$\begin{aligned} \left\Vert \partial _\theta p^{\mathrm{p}} \right\Vert _{L^2(\mathcal {O}')} \le C\big ( \left\Vert \nabla {\varvec{u}}^{\mathrm{p}} \right\Vert _{L^2(\varOmega _\epsilon )} +\left\Vert p^{\mathrm{p}} \right\Vert _{L^2(\varOmega _\epsilon )} \big ). \end{aligned}$$

(A.13)

Now we can use the tangential bounds (A.9), (A.10), (A.12), and (A.13) to obtain an estimate for derivatives $\nabla (\partial _\rho {\varvec{u}}^{\mathrm{p}})$ normal to $\Gamma _\epsilon$. For this, we will use the full Stokes Eqs. (1.8), written with respect to the orthonormal frame ${\varvec{e}}_t$, ${\varvec{e}}_\rho$, ${\varvec{e}}_\theta$ in $\mathcal {O}$ as

$$\begin{aligned} - \varDelta {\varvec{u}}^{\mathrm{p}} +\nabla p^{\mathrm{p}}&= -\varDelta {\varvec{u}}^{\mathrm{p}} + \frac{\partial p^{\mathrm{p}}}{\partial \rho }{\varvec{e}}_{\rho } + \frac{1}{\rho }\frac{\partial p^{\mathrm{p}}}{\partial \theta }{\varvec{e}}_{\theta } + \frac{1}{1-\rho \widehat{\kappa }}\bigg (\frac{\partial p^{\mathrm{p}}}{\partial s}-\kappa _3\frac{\partial p^{\mathrm{p}}}{\partial \theta }\bigg ){\varvec{e}}_t =0, \\ \mathrm{{div}\,}{\varvec{u}}^{\mathrm{p}}&= \frac{1}{1-\rho \widehat{\kappa }}\bigg (\frac{1}{\rho }\frac{\partial (\rho (1-\rho \widehat{\kappa }) u_{\rho })}{\partial \rho }+\frac{1}{\rho }\frac{\partial ((1-\rho \widehat{\kappa }) u_{\theta })}{\partial \theta } + \frac{\partial u_s}{\partial s} \bigg ) = 0. \end{aligned}$$

Here, $\widehat{\kappa }$ is as in (A.3) and we recall the notation $u_\rho = {\varvec{u}}^{\mathrm{p}}\cdot {\varvec{e}}_\rho$, $u_\theta ={\varvec{u}}^{\mathrm{p}}\cdot {\varvec{e}}_\theta$, $u_s={\varvec{u}}^{\mathrm{p}}\cdot {\varvec{e}}_t$.

From the divergence-free condition on ${\varvec{u}}^{\mathrm{p}}$, after multiplying through by $\rho (1-\rho \widehat{\kappa })$ and differentiating once with respect to $\rho$, we obtain

$$\begin{aligned} \left\Vert \frac{\partial ^2 u_{\rho }}{\partial ^2 \rho } \right\Vert _{L^2(\mathcal {O})}&\le C\bigg (\left\Vert \frac{1}{\rho } \nabla {\varvec{u}}^{\mathrm{p}} \right\Vert _{L^2(\mathcal {O})} + \bigg \Vert \frac{1}{\rho }\bigg \Vert _{L^{\infty }(\mathcal {O})}|\mathcal {O}|^{1/3}\big \Vert {\varvec{u}}^{\mathrm{p}} \big \Vert _{L^6(\mathcal {O})} \\&\quad + \bigg \Vert \frac{1}{\rho }\frac{\partial }{\partial \rho }\bigg (\frac{\partial u_{\theta }}{\partial \theta }\bigg )\bigg \Vert _{L^2(\mathcal {O})} +\bigg \Vert \frac{\partial }{\partial \rho }\bigg (\frac{\partial u_s}{\partial s}\bigg )\bigg \Vert _{L^2(\mathcal {O})} \bigg )\\&\le \frac{C}{\epsilon }\left|\log \epsilon \right|^{1/2}\big ( \Vert \nabla {\varvec{u}}^{\mathrm{p}}\Vert _{L^2(\varOmega _\epsilon )} +\left\Vert p^{\mathrm{p}} \right\Vert _{L^2(\varOmega _\epsilon )} \big ), \end{aligned}$$

where we have used (A.9) and (A.10) along with the Sobolev inequality on $\varOmega _\epsilon$.

Furthermore, using the ${\varvec{e}}_\rho$ component of $-\varDelta {\varvec{u}}^{\mathrm{p}} +\nabla p=0$, we have

$$\begin{aligned} \frac{\partial p^{\mathrm{p}}}{\partial \rho }&= (\varDelta {\varvec{u}}^{\mathrm{p}}) \cdot {\varvec{e}}_{\rho } \\&= \frac{1}{\rho (1-\rho \widehat{\kappa })}\frac{\partial }{\partial \rho }\left( \rho (1-\rho \widehat{\kappa })\frac{\partial {\varvec{u}}^{\mathrm{p}}}{\partial \rho }\right) \cdot {\varvec{e}}_{\rho } +\frac{1}{\rho ^2(1-\rho \widehat{\kappa })}\frac{\partial }{\partial \theta }\bigg ((1-\rho \widehat{\kappa })\frac{\partial {\varvec{u}}^{\mathrm{p}}}{\partial \theta }\bigg )\cdot {\varvec{e}}_{\rho } \\&\quad +\frac{1}{1-\rho \widehat{\kappa }} \frac{\partial }{\partial s}\bigg ( \frac{1}{1-\rho \widehat{\kappa }}\bigg [ \frac{\partial {\varvec{u}}^{\mathrm{p}}}{\partial s}- \kappa _3\frac{\partial {\varvec{u}}^{\mathrm{p}}}{\partial \theta } \bigg ] \bigg )\cdot {\varvec{e}}_{\rho } \\&= \frac{1}{\rho (1-\rho \widehat{\kappa })}\frac{\partial }{\partial \rho }\left( \rho (1-\rho \widehat{\kappa })\frac{\partial u_{\rho }}{\partial \rho }\right) +\frac{1}{\rho ^2(1-\rho \widehat{\kappa })}\frac{\partial }{\partial \theta }\bigg ((1-\rho \widehat{\kappa })\frac{\partial {\varvec{u}}^{\mathrm{p}}}{\partial \theta }\bigg )\cdot {\varvec{e}}_{\rho } \\&\quad +\frac{1}{1-\rho \widehat{\kappa }} \frac{\partial }{\partial s}\bigg ( \frac{1}{1-\rho \widehat{\kappa }}\bigg [ \frac{\partial {\varvec{u}}^{\mathrm{p}}}{\partial s}- \kappa _3\frac{\partial {\varvec{u}}^{\mathrm{p}}}{\partial \theta } \bigg ] \bigg )\cdot {\varvec{e}}_{\rho }, \end{aligned}$$

since ${\varvec{e}}_{\rho }(s,\theta )$ does not vary with $\rho$. Therefore, using (A.9), (A.10), (A.12), and (A.13), along with the bound on $\frac{\partial ^2 u_{\rho }}{\partial \rho ^2}$, we have

$$\begin{aligned} \Vert \nabla p^{\mathrm{p}}\Vert _{L^2(\mathcal {O}')} \le \frac{C}{\epsilon }\left|\log \epsilon \right|^{1/2}\big ( \Vert \nabla {\varvec{u}}^{\mathrm{p}}\Vert _{L^2(\varOmega _\epsilon )} +\left\Vert p^{\mathrm{p}} \right\Vert _{L^2(\varOmega _\epsilon )} \big ). \end{aligned}$$

Finally, to estimate $\frac{\partial ^2 u_j}{\partial \rho ^2}$, $j=\theta ,s$, we again use that

$$\begin{aligned} \nabla p^{\mathrm{p}}\cdot {\varvec{e}}_j&= (\varDelta {\varvec{u}}^{\mathrm{p}})\cdot {\varvec{e}}_j(s,\theta ) \\&= \frac{1}{\rho (1-\rho \widehat{\kappa })}\frac{\partial }{\partial \rho }\bigg (\rho (1-\rho \widehat{\kappa })\frac{\partial u_j}{\partial \rho }\bigg ) +\frac{1}{\rho ^2(1-\rho \widehat{\kappa })}\frac{\partial }{\partial \theta }\bigg ((1-\rho \widehat{\kappa })\frac{\partial {\varvec{u}}^{\mathrm{p}}}{\partial \theta }\bigg )\cdot {\varvec{e}}_j \\&\quad +\frac{1}{1-\rho \widehat{\kappa }} \frac{\partial }{\partial s}\bigg ( \frac{1}{1-\rho \widehat{\kappa }}\bigg [ \frac{\partial {\varvec{u}}^{\mathrm{p}}}{\partial s}- \kappa _3\frac{\partial {\varvec{u}}^{\mathrm{p}}}{\partial \theta } \bigg ] \bigg )\cdot {\varvec{e}}_j , \quad j=\theta ,s, \end{aligned}$$

since each of ${\varvec{e}}_t(s)$, ${\varvec{e}}_\rho (s,\theta )$ and ${\varvec{e}}_\theta (s,\theta )$ are independent of $\rho$. Then, we have

$$\begin{aligned} \bigg \Vert \frac{\partial ^2 u_j}{\partial \rho ^2}\bigg \Vert _{L^2(\mathcal {O}')}&\le C \bigg ( \bigg \Vert \frac{1}{\rho }\bigg \Vert _{L^{\infty }(\mathcal {O}')} \Vert \nabla {\varvec{u}}^{\mathrm{p}}\Vert _{L^2(\mathcal {O}')} + \bigg \Vert \frac{\partial ^2{\varvec{u}}^{\mathrm{p}}}{\partial s^2}\bigg \Vert _{L^2(\mathcal {O}')} \\&\quad + \bigg \Vert \frac{\partial ^2{\varvec{u}}^{\mathrm{p}}}{\partial s\partial \theta }\bigg \Vert _{L^2(\mathcal {O}')} +\bigg \Vert \frac{\partial ^2{\varvec{u}}^{\mathrm{p}}}{\partial \theta ^2}\bigg \Vert _{L^2(\mathcal {O}')}+\Vert \nabla p^{\mathrm{p}}\Vert _{L^2(\mathcal {O}')} \bigg ) \\&\le \frac{C}{\epsilon }\left|\log \epsilon \right|^{1/2} \big (\left\Vert \nabla {\varvec{u}}^{\mathrm{p}} \right\Vert _{L^2(\varOmega _\epsilon )} + \left\Vert p^{\mathrm{p}} \right\Vert _{L^2(\varOmega _\epsilon )} \big ), \quad j=\theta , s, \end{aligned}$$

where C depends only on $c_\Gamma$, $\kappa _{\max }$, and $\xi _{\max }$. Altogether, we obtain Lemma 9. $\square$

Remark 2

We note that the factor of $\frac{1}{\epsilon }$ in Lemma 9 is necessary. As a heuristic, we consider an infinite straight cylinder of radius $\epsilon$ and take ${\varvec{u}}= (\frac{1}{\rho }-\frac{1}{\epsilon }) {\varvec{e}}_{\theta }$, where ${\varvec{e}}_{\theta }$ is now the (constant) angular vector in straight cylindrical coordinates, and $p\equiv$ constant. Ignoring decay conditions toward infinity along the cylinder, $({\varvec{u}},p)$ solves the Stokes equations with ${\varvec{u}}=0$ on the cylinder surface. Then,

$$\begin{aligned} |\nabla ^2{\varvec{u}}| =\bigg | \frac{\partial ^2}{\partial \rho ^2} \frac{1}{\rho }\bigg | = \bigg |\frac{2}{\rho ^3}\bigg | = \frac{2}{\rho }\big | \nabla {\varvec{u}}\big |, \end{aligned}$$

and within the region $\epsilon < \rho \le 2 \epsilon$, we have $|\nabla ^2{\varvec{u}}| \ge \frac{1}{\epsilon }|\nabla {\varvec{u}}|$.