Skip to main content
SpringerLink
Log in

Thermo-rotational buckling and post-buckling analyses of rotating functionally graded microbeams

  • Published:
International Journal of Mechanics and Materials in Design Aims and scope Submit manuscript

Abstract

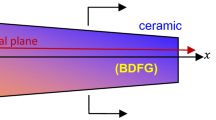
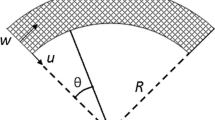
Buckling and post-buckling responses of rotating clamped–clamped functionally graded microbeams in thermal environment are examined on the basis of the Euler–Bernoulli beam assumption. To enrich the formulation with the size effect the modified couple stress theory is employed. The temperature dependency of material properties is considered. The nonlinear finite element technique alongside with the Newton–Raphson technique is utilized to extract the prestressed deformation. Moreover, the direct iteration method is employed to determine the buckling point and post-buckling equilibrium path. The impacts of material length scale parameter, volume fraction exponent, rotor radius, microbeam length to its thickness proportion and rotation speed on the presented outcomes are examined.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15
Fig. 16

Similar content being viewed by others

Abbreviations

\(A_{11}\) :

Axial rigidity

\(B_{11}\) :

Axial-bending rigidity

\(b\) :

Microbeam width

\(D_{11}\) :

Bending rigidity

\(E(z)\) :

FGM Young’s modulus

\(E_{mR}\) :

Metal phase Young’s modulus in reference temperate

\({\mathbf{F}}\) :

Force vector

\({\mathbf{F}}^{e}\) :

kth element force vector

\(h\) :

Microbeam thickness

\(h_{b}\) :

Bottom layer thickness

\(h_{c}\) :

Core layer thickness

\(h_{t}\) :

Top layer thickness

\(I_{0}\) :

Microbeam mass per its length

\(I_{1}\) :

First-order mass moment of inertia

\(I_{2}\) :

Second-order mass moment of inertia

\({\mathbf{K}}_{(1)}\) :

Linear stiffness matrix

\({\mathbf{K}}_{(2)}\) :

Second order stiffness matrix

\({\mathbf{K}}_{(3)}\) :

Third order stiffness matrix

\({\mathbf{K}}^{e}\) :

kth element stiffness matrix

\({\mathbf{K}}_{G}\) :

Geometric stiffness matrix

\({\mathbf{K}}_{NL}\) :

Nonlinear stiffness matrix

\({\mathbf{K}}_{(1)}^{El}\) :

Linear elastic stiffness matrix

\({\mathbf{K}}_{NL}^{EL}\) :

Nonlinear elastic stiffness matrix

\(L\) :

Micro-beam length

\(l\) :

Material length scale parameter

\(l_{e}\) :

Element length

\(M_{xx}\) :

Moment resultant

\(M_{xx}^{T}\) :

Thermal moment resultant

\(m_{xy}\) :

Couple stress moment

\({\mathbf{N}}_{u}\) :

Axial displacement shape function

\({\mathbf{N}}_{w}\) :

Deflection shape function

\(N_{xx}\) :

Axial force resultant

\(N_{xx}^{T}\) :

Thermal force resultant

\(N_{xx}^{T,cr}\) :

Critical thermal force resultant

\(n\) :

Volume fraction exponent

\(P_{c}\) :

Ceramic material property

\(P_{m}\) :

Metal material property

\(P_{xy}\) :

Couple stress moment resultant

\(Q_{11} (z)\) :

FGM elastic stiffness

\({\mathbf{q}}\) :

Microbeam generalized coordinate vector

\({\mathbf{q}}^{e}\) :

Element generalized coordinate vector

\(R\) :

Rotor radius

\({\mathbf{R}}_{NL}\) :

Prestressed residual vector

\(T\) :

Microbeam kinetic energy

\(T_{ref}\) :

Temperature reference (300 K)

\(U\) :

Microbeam strain energy

\(U_{0}\) :

Axial displacement of a typical particle

\(u(x,t)\) :

Axial displacement of a typical particle lied on geometrical middle plane of core layer

\(V_{c}\) :

Volume faction of ceramic phase

\({\mathbf{v}}\) :

Critical buckling eigenvector

\(W\) :

Deflection of a typical particle

\(w(x,t)\) :

Deflection of a typical particle lied on geometrical middle plane of core layer

\(w_{mid}\) :

Deflection of the microbeam mid-point

\(\hat{w}_{mid}\) :

Nondimensional deflection of the microbeam mid-point

\(\alpha (z)\) :

FGM thermal expansion coefficient

\(\Delta T\) :

Temperature increment

\(\Delta T_{Cr}\) :

Critical buckling temperature increment

\(\delta {\mathbf{q}}_{ps}\) :

Incremental prestressed generalized coordinate vector

\(\varepsilon^{T}\) :

Thermal strain

\(\varepsilon_{xx}\) :

Total strain

\(\varepsilon_{xx}^{0}\) :

Membrane strain

\(\varepsilon_{xx}^{1}\) :

Flexural strain (curvature)

\({\rm M}\) :

FGM size-dependent rigidity

\(\mu (z)\) :

FGM shear modulus

\(\nu\) :

FGM Poisson’s ratio

\(\xi\) :

Normalized local axial coordinate for an element

\(\rho (z)\) :

FGM mass density

\(\sigma_{xx}\) :

Cauchy stress

χ xy :

Curvature

\(\hat{\varOmega }\) :

Nondimensional rotation speed

\(\omega_{R}\) :

Rotation speed

References

  • Ahangar, S., Rezazadeh, G., Shabani, R., Ahmadi, G., Toloei, A.: On the stability of a microbeam conveying fluid considering modified couple stress theory. Int. J. Mech. Mater. Des. 7(4), 327 (2011)

    Article  Google Scholar 

  • Allahkarami, F., Nikkhah-bahrami, M., Ghassabzadeh Saryazdi, M.: Magneto-thermo-mechanical dynamic buckling analysis of a FG-CNTs-reinforced curved microbeam with different boundary conditions using strain gradient theory. Int. J. Mech. Mater. Des. 14(2), 243–261 (2018)

    Article  Google Scholar 

  • Arvin, H.: Free vibration analysis of micro rotating beams based on the strain gradient theory using the differential transform method: timoshenko versus Euler–Bernoulli beam models. Eur. J. Mech. A/Solids 65, 336–348 (2017)

    Article  MathSciNet  Google Scholar 

  • Arvin, H.: The flapwise bending free vibration analysis of micro-rotating Timoshenko beams using the differential transform method. J. Vib. Control 24(20), 4868–4884 (2018)

    Article  MathSciNet  Google Scholar 

  • Arvin, H.: On parametrically excited vibration and stability of beams with varying rotating speed. Iran. J. Sci. Technol. Trans. Mech. Eng. 43(2), 177–185 (2019)

    Article  Google Scholar 

  • Arvin, H., Sadighi, M., Ohadi, A.: A numerical study of free and forced vibration of composite sandwich beam with viscoelastic core. Compos. Struct. 92(4), 996–1008 (2010)

    Article  Google Scholar 

  • Arvin, H., Tang, Y.-Q., Ahmadi Nadooshan, A.: Dynamic stability in principal parametric resonance of rotating beams: method of multiple scales versus differential quadrature method. Int. J. Non-Linear Mech. 85, 118–125 (2016)

    Article  Google Scholar 

  • Arvin, H., Arena, A., Lacarbonara, W.: Nonlinear vibration analysis of rotating beams undergoing parametric instability: lagging-axial motion. Mech. Syst. Signal Process. 144, 106892 (2020)

    Article  Google Scholar 

  • Bayat, M., Sahari, B.B., Saleem, M., Hamouda, A.M.S., Reddy, J.N.: Thermo elastic analysis of functionally graded rotating disks with temperature-dependent material properties: uniform and variable thickness. Int. J. Mech. Mater. Des. 5(3), 263–279 (2009)

    Article  Google Scholar 

  • Chen, X., Li, Y.: Size-dependent post-buckling behaviors of geometrically imperfect microbeams. Mech. Res. Commun. 88, 25–33 (2018)

    Article  Google Scholar 

  • Esfahani, S.E., Kiani, Y., Eslami, M.R.: Non-linear thermal stability analysis of temperature dependent FGM beams supported on non-linear hardening elastic foundations. Int. J. Mech. Sci. 69, 10–20 (2013)

    Article  Google Scholar 

  • Heidari, M., Arvin, H.: Nonlinear free vibration analysis of functionally graded rotating composite Timoshenko beams reinforced by carbon nanotubes. J. Vib. Control 25(14), 2063–2078 (2019)

    Article  MathSciNet  Google Scholar 

  • Hieu, D.V.: Postbuckling and free nonlinear vibration of microbeams based on nonlinear elastic foundation. Math. Probl. Eng. 2018, 1031237 (2018)

    Article  MathSciNet  Google Scholar 

  • Kang, S., Lee, S.J., Prinz, F.B.: Size does matter: the pros and cons of miniaturization. ABB Rev. 2, 54–62 (2001)

    Google Scholar 

  • Khosravi, S., Arvin, H., Kiani, Y.: Interactive thermal and inertial buckling of rotating temperature-dependent FG-CNT reinforced composite beams. Compos. B Eng. 175, 107178 (2019a)

    Article  Google Scholar 

  • Khosravi, S., Arvin, H., Kiani, Y.: Vibration analysis of rotating composite beams reinforced with carbon nanotubes in thermal environment. Int. J. Mech. Sci. 164, 105187 (2019b)

    Article  Google Scholar 

  • Kiani, Y., Rezaei, M., Taheri, S., Eslami, M.R.: Thermo-electrical buckling of piezoelectric functionally graded material Timoshenko beams. Int. J. Mech. Mater. Des. 7(3), 185–197 (2011)

    Article  Google Scholar 

  • Meirovitch, L.: Principles and Techniques of Vibrations. Prentice Hall, Upper Saddle River (1997)

    Google Scholar 

  • Ramesh, M.N.V., Rao, N.M.: Free vibration analysis of pre-twisted rotating FGM beams. Int. J. Mech. Mater. Des. 9(4), 367–383 (2013)

    Article  Google Scholar 

  • Reddy, J.N.: Mechanics of Laminated Composite Plates and Shells: Theory and Analysis. CRC Press, Boca Raton (2003)

    Book  Google Scholar 

  • Reddy, J.N.: An Introduction to Nonlinear Finite Element Analysis. Oxford Univeristy Press, New York (2005)

    Google Scholar 

  • She, G.L., Yuan, F.G., Ren, Y.R.: Thermal buckling and post-buckling analysis of functionally graded beams based on a general higher-order shear deformation theory. Appl. Math. Model. 47, 340–357 (2017)

    Article  MathSciNet  Google Scholar 

  • Sun, Y., Li, S.R., Batra, R.C.: Thermal buckling and post-buckling of FGM Timoshenko beams on nonlinear elastic foundation. J. Therm. Stresses 39(1), 11–26 (2016)

    Article  Google Scholar 

  • Taati, E.: On buckling and post-buckling behavior of functionally graded micro-beams in thermal environment. Int. J. Eng. Sci. 128, 63–78 (2018)

    Article  MathSciNet  Google Scholar 

  • Wang, Y.G., Lin, W.H., Liu, N.: Nonlinear bending and post-buckling of extensible microscale beams based on modified couple stress theory. Appl. Math. Model. 39(1), 117–127 (2015)

    Article  MathSciNet  Google Scholar 

  • Zhang, D.G.: Thermal post-buckling and nonlinear vibration analysis of FGM beams based on physical neutral surface and high order shear deformation theory. Meccanica 49(2), 283–293 (2014)

    Article  MathSciNet  Google Scholar 

  • Zhang, D.G., Zhou, H.M.: Nonlinear bending and thermal post-buckling analysis of FGM beams resting on nonlinear elastic foundations. Comput. Model. Eng. Sci. 100(3), 201–222 (2014)

    MathSciNet  MATH  Google Scholar 

Download references

Funding

This research did not receive any specific grant from funding agencies in the public, commercial or not-for profit sectors.

Author information

Authors and Affiliations

  1. Faculty of Engineering, Shahrekord University, Shahrekord, Iran

    Seyyed Mohammad Hossein Hosseini & Hadi Arvin

Authors
  1. Seyyed Mohammad Hossein Hosseini
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Hadi Arvin
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Hadi Arvin.

Ethics declarations

Conflict of interest/Competing interests

The authors declare that they have no significant competing financial, professional, or personal interests that might have influenced the performance or presentation of the work described in this manuscript.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Appendix

Appendix

  1. a

    The element stiffness matrix

$${\mathbf{K}}^{e} = \sum\limits_{i = 1}^{15} {{\mathbf{K}}_{i} }$$
(A.1)
$$\begin{aligned} & {\mathbf{K}}_{1} = (l_{e} )\int_{0}^{1} {\left( {\frac{1}{{l_{e}^{2} }}} \right)A_{11} {\mathbf{N}}_{u}^{{{\prime }{\text{T}}}} {\mathbf{N}}_{u}^{{\prime }} } d\xi ,\quad {\mathbf{K}}_{2} = - (l_{e} )\int_{0}^{1} {\left( {\frac{1}{{l_{e}^{3} }}} \right)B_{11} } {\mathbf{N}}_{u}^{{{\prime }{\text{T}}}} {\mathbf{N}}_{w}^{{\prime \prime }} d\xi , \\ & {\mathbf{K}}_{3} = - (l_{e} )\int_{0}^{1} {\left( {\frac{1}{{l_{e}^{2} }}} \right)N_{xx}^{T} {\mathbf{N}}_{w}^{{{\prime }{\text{T}}}} } {\mathbf{N}}_{w}^{{\prime }} d\xi ,\quad {\mathbf{K}}_{4} = - (l_{e} )\int_{0}^{1} {\left( {\frac{1}{{l_{e}^{3} }}} \right)B_{11} } {\mathbf{N}}_{w}^{{{\prime \prime }{\text{T}}}} {\mathbf{N}}_{u}^{{\prime }} d\xi , \\ & {\mathbf{K}}_{5} = (l_{e} )\int_{0}^{1} {\left( {\frac{1}{{l_{e}^{4} }}} \right)D_{11} } {\mathbf{N}}_{w}^{{{\prime \prime }{\text{T}}}} {\mathbf{N}}_{w}^{{\prime \prime }} d\xi ,\quad {\mathbf{K}}_{6} = - (l_{e} )\int_{0}^{1} {I_{0} \omega_{R}^{2} {\mathbf{N}}_{u}^{\text{T}} {\mathbf{N}}_{u} d\xi } , \\ & {\mathbf{K}}_{7} = (l_{e} )\int_{0}^{1} {\left( {\frac{1}{{l_{e} }}} \right)I_{1} \omega_{R}^{2} {\mathbf{N}}_{u}^{\text{T}} {\mathbf{N}}_{w}^{{\prime }} d\xi } ,\quad {\mathbf{K}}_{8} = (l_{e} )\int_{0}^{1} {\left( {\frac{1}{{l_{e} }}} \right)I_{1} \omega_{R}^{2} {\mathbf{N}}_{w}^{{{\prime }{\text{T}}}} {\mathbf{N}}_{u} d\xi } , \\ & {\mathbf{K}}_{9} = - (l_{e} )\int_{0}^{1} {\left( {\frac{1}{{l_{e}^{2} }}} \right)I_{2} \omega_{R}^{2} {\mathbf{N}}_{w}^{{{\prime }{\text{T}}}} {\mathbf{N}}_{w}^{{\prime }} d\xi } ,\quad {\mathbf{K}}_{10} = (l_{e} )\int_{0}^{1} {\left( {\frac{1}{{l_{e}^{4} }}} \right){\rm M}{\mathbf{N}}_{w}^{{{\prime \prime }{\text{T}}}} {\mathbf{N}}_{w}^{{\prime \prime }} d\xi } , \\ & {\mathbf{K}}_{11} = (l_{e} )\int_{0}^{1} {\left( {\frac{1}{{l_{e}^{2} }}} \right)A_{11} } \frac{du}{dx}{\mathbf{N}}_{w}^{{{\prime }{\text{T}}}} {\mathbf{N}}_{w}^{{\prime }} d\xi ,\quad {\mathbf{K}}_{12} = \frac{1}{2}(l_{e} )\int_{0}^{1} {\left( {\frac{1}{{l_{e}^{2} }}} \right)A_{11} } \frac{dw}{dx}{\mathbf{N}}_{u}^{{{\prime }{\text{T}}}} {\mathbf{N}}_{w}^{{\prime }} d\xi , \\ & {\mathbf{K}}_{13} = - (l_{e} )\int_{0}^{1} {\left( {\frac{1}{{l_{e}^{3} }}} \right)B_{11} } \frac{dw}{dx}{\mathbf{N}}_{w}^{{{\prime }{\text{T}}}} {\mathbf{N}}_{w}^{{\prime \prime }} d\xi ,\quad {\mathbf{K}}_{14} = - \frac{1}{2}(l_{e} )\int_{0}^{1} {\left( {\frac{1}{{l_{e}^{3} }}} \right)B_{11} } \frac{dw}{dx}{\mathbf{N}}_{w}^{{{\prime \prime }{\text{T}}}} {\mathbf{N}}_{w}^{{\prime }} d\xi , \\ & {\mathbf{K}}_{15} = \frac{1}{2}(l_{e} )\int_{0}^{1} {\left( {\frac{1}{{l_{e}^{2} }}} \right)A_{11} } (\frac{dw}{dx})^{2} {\mathbf{N}}_{w}^{{{\prime }{\text{T}}}} {\mathbf{N}}_{w}^{{\prime }} d\xi , \\ \end{aligned}$$
(A.2)
  1. b

    The kth element force vector

$${\mathbf{F}}^{e} = \sum\limits_{i = 1}^{6} {{\mathbf{F}}_{i} }$$
(A.3)
$$\begin{aligned} & {\mathbf{F}}_{1} = (l_{e} )\int_{0}^{1} {\left( {\frac{1}{{l_{e} }}} \right)N_{xx}^{T} } {\mathbf{N}}_{u}^{{{\prime }{\text{T}}}} d\xi ,\quad {\mathbf{F}}_{2} = - (l_{e} )\int_{0}^{1} {\left( {\frac{1}{{l_{e}^{2} }}} \right)M_{xx}^{T} } {\mathbf{N}}_{w}^{{{\prime \prime }{\text{T}}}} d\xi ,\quad {\mathbf{F}}_{3} = (l_{e} )\int_{0}^{1} {I_{0} \omega_{R}^{2} R} {\mathbf{N}}_{u}^{\text{T}} d\xi , \\ & {\mathbf{F}}_{4} = (l_{e} )\int_{0}^{1} {I_{0} \omega_{R}^{2} \left( {(k - 1) + \xi } \right)} l_{e} {\mathbf{N}}_{u}^{\text{T}} d\xi ,\quad {\mathbf{F}}_{5} = - (l_{e} )\int_{0}^{1} {\left( {\frac{1}{{l_{e} }}} \right)I_{1} \omega_{R}^{2} R} {\mathbf{N}}_{w}^{{{\prime }{\text{T}}}} d\xi , \\ & {\mathbf{F}}_{6} = - (l_{e} )\int_{0}^{1} {\left( {\frac{1}{{l_{e} }}} \right)I_{1} \omega_{R}^{2} \left( {(k - 1) + \xi } \right)l_{e} } {\mathbf{N}}_{w}^{{{\prime }{\text{T}}}} d\xi \\ \end{aligned}$$
(A.4)

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Hosseini, S.M.H., Arvin, H. Thermo-rotational buckling and post-buckling analyses of rotating functionally graded microbeams. Int J Mech Mater Des 17, 55–72 (2021). https://doi.org/10.1007/s10999-020-09509-7

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10999-020-09509-7

Keywords

Search

Navigation