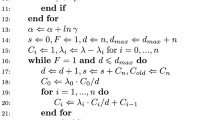Abstract
In this article, we consider random occupancy models and the related problems based on the methods of generating functions. The waiting time distributions associated with sequential random occupancy models are investigated through the probability generating functions. We provide the effective computational tools for the evaluation of the probability functions by making use of the Bell polynomials. The results presented here provide a wide framework for developing the theory of occupancy models. Finally, we treat several examples in order to demonstrate how our theoretical results are employed for the investigation of the random occupancy models along with numerical results.
Similar content being viewed by others
References
Billingsley P (1995) Probability and measure, 3rd edn. Wiley, New York, . Inc
Balakrishnan N, Koutras MV, Milienos FS (2014) Some binary start-up demonstration tests and associated inferential methods. Ann Inst Stat Math 66:759–787
Boutsikas MV, Antzoulakos DL, Rakitzis AC (2014) On the joint distribution of stopping times and stopped sums in multistate exchangeable trials. J Appl Probab 51:483–491
Charalambides CHA (2002) Enumerative combinatorics. Chapman & Hall/CRC, Boca Raton
Charalambides CHA (2005) Combinatorial methods in discrete distributions. Wiley, New York
Charalambides CHA (2006) A unified derivation of the complementary waiting time distribution in sequential occupancy. Methodol Comput Appl Probab 8:345–356
Durrett R (1996) Probability: Theory and Examples, 2nd edn. Duxbury Press, Belmont
Eryilmaz S (2008) Distribution of runs in a sequence of exchangeable multi-state trials. Stat Prob Lett 78:1505–1513
Feller W (1968) An introduction to probability theory and its applications, vol I, 3rd edn. Wiley, New York
Frankowski K, Sobel M (2005) Solutions of some birthday problems using Dirichlet integrals. Commun Stat Theor Meth 34:1393–1410
Fu JC, Lee WC (2017) On coupon collector’s and Dixie cup problems under fixed and random sample size sampling schemes. Ann Inst Stat Math 69:1129–1139
Gadrich T, Ravid R (2011) The sequential occupancy problem through group throwing of indistinguishable balls. Methodol Comput Appl Probab 13:433–448
Glaz J, Naus J, Wallenstein S (2001) Scan Statistics. Springer, New York
Inoue K, Aki S (2009) On waiting time distributions associated with compound patterns in a sequence of multi-state trials. Ann Inst Stat Math 61:499–516
Inoue K, Aki S, Hirano K (2011) Distributions of simple patterns in some kinds of exchangeable sequence. J Stat Plan Inf 141:2532–2544
Inoue K, Aki S (2018) On discrete distributions generated from drawing balls with bivariate labels. Commun Stat Theor Meth 47:3532–3546
Johnson NL, Kotz S (1977) Urn models and their applications. Wiley, New York
Johnson NL, Kotz S, Balakrishnan N (1997) Discrete multivariate distributions. Wiley, New York
Johnson NL, Kemp AW, Kotz S (2005) Univariate discrete distributions, 3rd edn. Wiley, New York
Kemp AW (1980) Conditionally properties for the bivariate logarithmic distribution with an application to goodness of fit. Stat Distribut Sci Work 5:57–73
Kemp AW (1981) Computer sampling from homogeneous bivariate discrete distributions. In: ASA Proceedings of the Statistical Computing Section, pp 173–175
Kobza JE, Jacobson SH, Vaughan DE (2007) A survey of the coupon collector’s problem with random sample sizes. Methodol Comput Appl Probab 9:573–584
Kotz S, Balakrishnan N (1997). In: Balakrishnan N (ed) Advances in Urn Models During the Past Two Decades, Advances in Combinatorial Methods and Applications to Probability and Statistics. Birkhäuser, Boston, pp 203–257
Krauth J (1992) Bounds for the upper-tail probabilities of the circular ratchet scan statistic. Biometrics 48:1177–1185
Krauth J (1999). In: Glaz J, Balakrishnan N (eds) Ratchet scan and disjoint scan statistics, Scan Statistics and Applications. Birkhäuser, Boston, pp 67–96
Mahmoud H (2008) Pólya Urn Models. Chapman & Hall
Makri FS, Philippou AN, Psillakis ZM (2007) Success run statistics defined on an urn model. Adv Appl Probab 39:991–1019
Riordan (1958) An introduction to combinatorial analysis. Wiley, New York
Sobel M, Frankowski K (2004). In: Gupta AK, Nadarajah S (eds) Extensions of Dirichlet integrals: their computation and probability applications, Handbook of Beta Distribution and Its Applications. Marcel Dekker, New York, pp 319–360
Acknowledgments
The author wishes to thank the editor and the referees for careful reading of our paper and helpful suggestions which led to improved results.
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher’s Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Appendices
Appendix A: Bell Polynomials
We provide the necessary facts of the Bell polynomials briefly. For more details on these polynomials and their generalizations, the interested reader is referred to Charalambides (2002).
The polynomial Bn,k ≡ Bn,k(x1,...,xn) in the variables x1,...,xn of degree k defined by the sum
is called (exponential) partial Bell partition polynomial, where k1, k2,...,kn are nonnegative integers. The Eq. 1 readily yields
The generating function of the partial Bell polynomials Bn,k(x1,...,xn), k = 0, 1,...n, n = 0, 1,..., is given by
The partial Bell polynomials Bn,k(x1,...,xn), k = 0, 1,...,n, n = 0, 1,..., with B0,0 ≡ 1 can be evaluated through the well-known recurrence relations
for k = 0, 1,...,n, n = 0, 1,....
Appendix B: Faa di Bruno Formula
We show that the derivative of order n of a composite function is expressed as a partial Bell polynomial.
Theorem 1
Let f(u) and g(z) be two functions of real variables for which all the derivatives,
exist. Then the derivatives of the composite function \(h(z)=f \left (g \left (z \right ) \right )\) are expressed as the partial Bell polynomials
Appendix C: Proofs
We provide the proofs of the theorems and propositions presented in Sections 3 and 4.
1.1 C.1 Proof of Theorem 1
Observing that
the proof is completed.
1.2 C.2 Proof of Theorem 2
It is easy to see that
Further,
and from Faa di Bruno formula (see Theorem 4 in Appendix B), we have
The proof is completed.
1.3 C.3 Proof of Proposition 1
We see that ϕn(z) = [g(p1z1 + ⋯ + pmzm)]n with [g(p1 + ⋯ + pm)]n = 1. Therefore, the multivariate random variable \(\mathbf {N}_{n}=({N_{n}^{1}},{N_{n}^{2}},...,{N_{n}^{m}})\) is of the homogeneous type from the Definition 1. The proof is completed.
1.4 C.4 Proof of Proposition 3
Observing first that
we get
It is easy to see that
The proof is completed.
1.5 C.5 Proof of Theorem 3
Inoue and Aki (2009) elucidated the relation between the distributions of Tr(x), x = 1,m and \(({N_{n}^{1}},...,{N_{n}^{m}})\) in terms of the double generating functions:
By virtue of Eq. 1, the proof is completed.
Rights and permissions
About this article
Cite this article
Inoue, K. On Homogeneous Multivariate Distributions in Random Occupancy Models and Their Applications. Methodol Comput Appl Probab 23, 1129–1153 (2021). https://doi.org/10.1007/s11009-020-09807-9
Received:
Revised:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11009-020-09807-9
Keywords
- Random occupancy models
- Urn model
- Occupancy numbers
- Waiting times
- Bell polynomials
- Probability function
- Probability generating function
- Double generating function
- Scan statistics
- Birthday problem
- Coupon collection problem
- Pólya urn model.