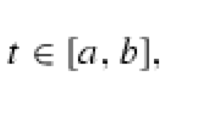Abstract
We consider expressions \(\mathcal {L}_k[y]\) that are ordered products of \(k\), \(k\in \mathbb {N} \), linear symmetric quasidifferential expressions of the second order with matrix coefficients. Asymptotic formulas as \(x\to +\infty \) are derived for one of the fundamental solution systems of the equation \(\mathcal {L}_k[y]=\lambda y \), \(\lambda \in \mathbb {C} \), \(\lambda \ne 0\), under some conditions on the behavior at infinity of its coefficients. The result is applied to the study of spectral properties of operators generated by the expression \(\mathcal {L}_k[y] \), and, in particular, to second-order matrix differential operators of the Sturm–Liouville type.
Similar content being viewed by others
Notes
This implies that the number of Jordan blocks corresponding to the root \(z_{q+p} \) is \(s_p \) and their orders \(i_1 \), \(i_2 \), . . . , \(i_{s_p} \) satisfy the relation \(i_1+i_2+\ldots +i_{s_p}=r_p \); moreover, \(\sum _{p=1}^l r_p+q=2nk \) and \(\max \limits _{\genfrac {}{}{0pt}{1}{1\le m\le s_p}{1\le p\le l}}i_m=r+1 \).
REFERENCES
Coddington, E.A. and Levinson, N., Theory of Ordinary Differential Equations, New York: McGraw-Hill, 1955.
Faedo, S., Proprieta asintotiche delle soluzioni dei sistemi differenziali lineari,Ann. Mat. Pura Appl., 1947, vol. 26, no. 4, pp. 207–215.
Braeutigam, I.N., Mirzoev, K.A., and Safonova, T.A., An analog of Orlov’s theorem on the deficiency index of second-order differential operators, Math. Notes, 2015, vol. 97, no. 2, pp. 300–303.
Braeutigam, I.N., Mirzoev, K.A., and Safonova, T.A., On deficiency index for some second order vector differential operators, Ufa Math. J., 2017, vol. 9, no. 1, pp. 18–28.
Orlov, S.A., On the deficiency index of linear differential equations,Dokl. Akad. Nauk SSSR, 1953, vol. 92, no. 3, pp. 483–486.
Savchuk, A.M. and Shkalikov, A.A., Sturm–Liouville operator with singular potentials, Tr. Mosk. Mat. O-va, 2003, vol. 64, pp. 159–212.
Kostenko, A.S. and Malamud, M.M., 1-D Schrödinger operators with local point interactions on a discrete set, J. Differ. Equat., 2010, vol. 249, no. 2, pp. 253–304.
Mirzoev, K.A. and Safonova, T.A., On the deficiency index of the vector-valued Sturm–Liouville operator, Math. Notes, 2016, vol. 99, no. 2, pp. 290–303.
Kostenko, A.S., Malamud, M.M., and Natyagailo, D.D., Matrix Schrödinger operator with \(\delta \)-interactions,Math. Notes, 2016, vol. 100, no. 1, pp. 49–65.
Braeutigam, I.N. and Mirzoev, K.A., Deficiency indices of the operators generated by infinite Jacobi matrices with operator entries, St. Petersburg Math. J., 2019, vol. 30, pp. 621–638.
Braeutigam, I.N., Limit-point criteria for the matrix Sturm–Liouville operator and its powers, Opuscula Math., 2017, vol. 37, no. 1, pp. 5–19.
Lesch, M. and Malamud, M.M., On the deficiency indices and self-adjointness of symmetric Hamiltonian system, J. Differ. Equat., 2003, vol. 189, no. 2, pp. 556–615.
Braeutigam, I.N. and Mirzoev, K.A., Asymptotics of solutions of matrix differential equations with nonsmooth coefficients, Math. Notes, 2018, vol. 104, no. 1, pp. 150–155.
Anderson, R.L., Limit-point and limit-circle criteria for a class of singular symmetric differential operators, Can. J. Math., 1976, vol. 28, no. 5, pp. 905–914.
Savchuk, A.M. and Shkalikov, A.A., Sturm–Liouville operators with singular potentials, Math. Notes, 1999, vol. 66, no. 6, pp. 897–912.
Everitt, W.N. and Zettl, A., The number of integrable-square solutions of products of differential expressions, Proc. R. Soc. Edinburgh, 1977, vol. 76, pp. 215–226.
Kauffman, R.M., Read, T.T., and Zettl, A., The Deficiency Index Problem for Powers of Ordinary Differential Expressions, Berlin–Heidelberg–New York: Springer, 1977.
Ewans, W.D. and Zettl, A., Interval limit-point criteria for differential expressions and their powers, J. London Math. Soc., 1977, vol. 15, no. 2, pp. 119–133.
Zettl, A., Sturm–Liouville Theory. Mathematical Surveys and Monographs. Vol. 121 , Providence: Am. Math. Soc., 2005.
Weidmann, J., Spectral Theory of Ordinary Differential Operators. Lecture Notes in Mathematics, Berlin–Heidelberg: Springer, 1987.
Akhiezer, N.I. and Glazman, I.M., Teoriya lineinykh operatorov v gil’bertovom prostranstve (Theory of Linear Operators in a Hilbert Space), Moscow: Nauka, 1966.
Naimark, M.A., Lineinye differentsial’nye operatory (Linear Differential Operators), Moscow: Nauka, 1969.
Martynov, V.V., Conditions for the discreteness and continuity of spectrum in the case of a self-adjoint system of equations of even order, Differ. Uravn., 1965, vol. 1, no. 12, pp. 1578–1591.
Gasymov, M.G., Zhikov, V.V., and Levitan, B.M., Conditions for the discreteness and finiteness of the negative spectrum of operator Schrödinger equation,Mat. Zametki, 1967, vol. 2, no. 5, pp. 531–538.
Yafaev, D.R., On the negative spectrum of operator Schrödinger equation, Mat. Zametki, 1970, vol. 7, no. 6, pp. 753–763.
ACKNOWLEDGMENTS
The author is deeply indebted to K.A. Mirzoev for posing this problem and for useful discussions and to the reviewer for valuable comments and additions.
Funding
This work was supported by the joint program “Mikhail Lomonosov” of the Russian Ministry of Education and Science and DAAD under grant no. 1.12791.2018/12.2.
Author information
Authors and Affiliations
Corresponding author
Additional information
Translated by V. Potapchouck
Rights and permissions
About this article
Cite this article
Braeutigam, I.N. Spectral Properties of Matrix Differential Equations with Nonsmooth Coefficients. Diff Equat 56, 685–695 (2020). https://doi.org/10.1134/S0012266120060026
Received:
Revised:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1134/S0012266120060026