Abstract
In this paper the mathematical formulation of the equilibrium problem of high-flexible beams in the framework of fully nonlinear structural mechanics is presented. The analysis is based on the recent model proposed by L. Lanzoni and A.M. Tarantino: The bending of beams in finite elasticity in J. Elasticity (2019) doi:10.1007/s10659-019-09746-8 2019. In this model the complete three-dimensional kinematics of the beam is taken into account, both deformations and displacements are considered large and a nonlinear constitutive law in assumed. After having illustrated and discussed the peculiar mechanical aspects of this special class of structures, the criteria and methods of analysis have been addressed. A classification of the structures based on the degree of kinematic constraints has been proposed, distinguishing between isogeometric and hypergeometric structures. External static loads dependent on deformation (live loads) are also considered. The governing equations are derived on the basis of a moment-curvature relationship obtained in L. Lanzoni and A.M. Tarantino: The bending of beams in finite elasticity in J. Elasticity (2019) doi:10.1007/s10659-019-09746-8 2019. The governing equations take the form of a highly nonlinear coupled system of equations in integral form, which is solved through an iterative numerical procedure. Finally, the proposed analysis is applied to some popular structural systems subjected to dead and live loads. The results are compared and discussed.











Similar content being viewed by others
Notes
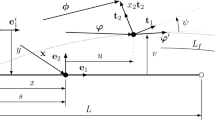
The function \(v(s)\) for \(v(0, 0, Z)\), \(w(s)\) for \(w(0, 0, Z)\) and \(\theta (s)\) for \(\theta (0, 0, Z)\) have been written with abuse of notation. Since the beam axis is inflexed in the vertical plane \(X=0\), the component \(u(s)\) in the first equation of the system (1) is null.
The following derivatives were used to calculate the displacement gradient:
$$\begin{gathered} \frac{\partial v(s)}{\partial s}=-\sin \theta (s),\quad \frac{\partial w(s)}{\partial s}=-1+\cos \theta (s), \\ \frac{\partial \sin \theta (s)}{\partial s}=\frac{1}{R(s)}\cos \theta (s),\quad \frac{\partial \cos \theta (s)}{\partial s}=- \frac{1}{R(s)}\sin \theta (s). \end{gathered}$$In addition, the transversal radius \(r(s)\) must not be understood as an independent function in the variable s. In fact, in the kinematic model the radius \(r(s)\) is evaluated by means of a constitutive relation which exclusively involves the local value of the longitudinal radius \(R(s)\) and is not affected by the latter’s variations along the beam axis, i.e., \(\frac{\partial r(s)}{\partial s}=0\).
A compressible Mooney-Rivlin stored energy function has the following form [31], [32] and [33]:
$$ \omega (I_{1}, I_{2}, I_{3})=a\, I_{1}+b\, I_{2}+c\, I_{3}- \frac{d}{2}\, \ln I_{3}, $$where \(I_{1}=\lambda _{X}^{2}+\lambda _{Y}^{2}+\lambda _{Z}^{2}\), \(I_{2}=\lambda _{X}^{2}\lambda _{Y}^{2}+\lambda _{X}^{2}\lambda _{Z}^{2}+ \lambda _{Y}^{2}\lambda _{Z}^{2}\), \(I_{3}=\lambda _{X}^{2}\lambda _{Y}^{2}\lambda _{Z}^{2}\) and \(d=2 (a+2\, b+c )\). This last condition has been determined by imposing that when the stretches are unitary the stresses are null (see, e.g., [34], [35] and [36]). Other details on the above stored energy function can be found in [37] and [38].
The basic line of the beam is the curve with longitudinal stretch \(\lambda _{Z}\) equal to one. Therefore, in the case of inflexed beams, although the beam itself is not, its basic line is inextensible. In the case of slender beams, the basic line coincides with the beam axis, i.e., with the curve that collects the centroids of the cross sections [1].
By imposing the conditions of smallness of the displacement and deformation fields and adopting the classic Navier constitutive law, formula (8) transforms into the well-known linear moment-curvature relationship [29]
$$ m_{X}(Z)=EJ_{X}\, \frac{d^{2}v}{dZ^{2}}, $$where the curvature \(\frac{1}{R(Z)}=\frac{d^{2}v}{dZ^{2}}\) is evaluated in an approximate way, neglecting \(\frac{dv}{dZ}\) and ignoring the displacement \(w(Z)\); the bending moment \(m_{X}(Z)\) is evaluated with respect to the undeformed configuration of the beam and the constant \(E\) indicates the Young modulus. Since compatibility and equilibrium conditions have been used to obtain this formula, in linear theory, the statically indeterminate structures can be solved using it.
If the rotation \(\theta (s)\) is added to the angle \(\gamma \) then the load retains its direction. In this case, the load can simulate for example the action of a stationary wind.
In a slender beam the deformation effects generated by the bending moment are more important than those produced by normal force and shear force, which, for this reason, are generally neglected.
This first step of the analysis, aimed at the assessing of deflections curves, can also be performed using a nonlinear Elastica equation, i.e. evaluating the curvature in a geometrically exact manner and calculating the bending moment with respect to the deformed axis. This is as long as the curvatures are sufficiently small [1].
For this case, the correctness of the normal and shear force at the clamped end can be checked later. In fact, taking a deflection curve, a straight horizontal segment and a vertical ones can be traced joining the free end of the beam with the clamped section, in order to realize a closed simply connected profile. Now we can think that this profile is subjected to a uniform pressure \(g'\), therefore the normal and shear force at the clamped end are the resultants of the pressures on the vertical and horizontal segments, respectively.
If the bending stiffness tends to zero, the deflection curves approach the shape of the catenary.
If the two ends of a deflection curve are joined with a horizontal segment, a closed profile is obtained. The pressure multiplied by the length of the segment is equal to the sum of the two vertical reactions.
If the flexural stiffness tends to zero, the deflection curves approach the shape assumed by half of the cross section of a thin balloon subjected to internal pressure.
References
Lanzoni, L., Tarantino, A.M.: The bending of beams in finite elasticity. J. Elast. (2019). https://doi.org/10.1007/s10659-019-09746-8
Bernoulli, J.: Specimen alterum calculi differentialis in dimetienda spirali logarithmica, loxodromiis nautarum et areis triangulorum sphaericorum. Una cum additamento quodam ad problema funicularium. aliisque. Acta Eruditorum, Junii (1691) 282-290 – Opera, 442-453
Bernoulli, D.: The 26th letter to Euler (1742). Correspondance mathématique et physique de quelques célèbres géomètres du XVIIIème siècle. Tome 2, P. H. Fuss, St. Pétersbourg (1843)
Euler, L.: Additamentum I de curvis elasticis, methodus inveniendi lineas curvas maximi minimivi proprietate gaudentes. Bousquent, Lausanne (1744)
Euler, L.: Genuina principia doctrinae de statu aequilibrii et motu corporum tam perfecte flexibilium quam elasticorum. Opera Omnia II 11, 37–61 (1771)
de Lagrange, J.L.: Sur la figure des colonnes. Misc. Taurinensia 5, 125–170 (1770–1773)
Born, M.: Untersuchungen über die Stabilität der elastischen Linie in Ebene und Raum, under verschiedenen Grenzbedingungen. PhD thesis, University of Göttingen, Germany (1906)
Barten, H.J.: On the deflection of a cantilever beam. Q. Appl. Math. 2, 168–171 (1944)
Barten, H.J.: On the deflection of a cantilever beam. Q. Appl. Math. 3, 275–276 (1945)
Bisshopf, K.E., Drucker, D.C.: Large deflection of cantilever beams. Q. Appl. Math. 3, 272–275 (1945)
Rohde, F.V.: Large deflections of a cantilever beam with uniformly distributed load. Q. Appl. Math. 11, 337–338 (1953)
Frisch-Fay, R.: Flexible Bars. Butterworths, London (1962)
Wang, J., Chen, J.K., Liao, S.: An explicit solution of the large deformation of a cantilever beam under point load at the free tip. J. Comput. Appl. Math. 212, 320–330 (2008)
Wang, T.M., Lee, S.L., Zienkiewicz, O.C.: Numerical analysis of large deflections of beams. Int. J. Mech. Sci. 3, 219–228 (1961)
Wang, T.M.: Non-linear bending of beams with uniformly distributed loads. Int. J. Non-Linear Mech. 4, 389–395 (1969)
Armanini, C., Dal Corso, F., Misseroni, D., Bigoni, D.: From the elastica compass to the elastica catapult: an essay on the mechanics of soft robot arm. Proc. R. Soc. A 473, 2198 (2017). https://doi.org/10.1098/rspa.2016.0870
Della Corte, A., dell’Isola, F., Esposito, R., Pulvirenti, M.: Equilibria of a clamped Euler beam (Elastica) with distributed load: large deformations. Math. Models Methods Appl. Sci. 27, 1391–1421 (2017)
Silverman, M.P., Farrah, J.: Bending of a tapered rod: modern application and experimental test of elastica theory. World J. Mech. 8, 272–300 (2018)
Batista, M.: Large deflections of an elastic rod in contact with a flat wall. Int. J. Solids Struct. 115–116, 53–60 (2017)
Cazzolli, A., Misseroni, D., Del Corso, F.: Elastica catastrophe machine: theory, design and experiments. J. Mech. Phys. Solids (2019). https://doi.org/10.1016/j.jmps.2019.103735. In press
Elettro, H., Grandgeorge, P., Neukirch, S.: Elastocapillary coiling of an elastic rod inside a drop. J. Elast. 127, 235–247 (2017)
Liakou, A.: Application of optimal control method in buckling analysis of constrained elastica problems. Int. J. Solids Struct. 141–142, 158–172 (2018)
Sano, T.G., Wada, H.: Snap-buckling in asymmetrically constrained elastic strips. Phys. Rev. E 97, 013002 (2018)
Singh, H., Hanna, J.A.: On the planar Elastica, stress, and material stress. J. Elast. 136, 87–101 (2019)
Bigoni, D., Bosi, F., Misseroni, D., Del Corso, F., Novelli, G.: New phenomena in nonlinear elastic structures: from tensile buckling to configurational forces. In: Bigoni, D. (ed.) Extremely Deformable Structures. CISM Lecture Notes, vol. 562. Springer, Berlin (2015). ISBN 978-3-7091-1876-4
O’Reilly, O.M.: Modeling Nonlinear Problems in the Mechanics of Strings and Rod. Springer, Berlin (2017)
Lanzoni, L., Tarantino, A.M.: Finite anticlastic bending of hyperelastic solids and beams. J. Elast. 131, 137–170 (2018). https://doi.org/10.1007/s10659-017-9649-y
Rivlin, R.S.: Large elastic deformations of isotropic materials. V. The problem of flexure. Proc. R. Soc. Lond. A 195, 463–473 (1949)
Falope, F.O., Lanzoni, L., Tarantino, A.M.: The bending of fully nonlinear beams. Theoretical, numerical and experimental analyses. Int. J. Eng. Sci. 145, 103167 (2019)
Falope, F.O., Lanzoni, L., Tarantino, A.M.: Bending device and anticlastic surface measurement of solids under large deformations and displacements. Mech. Res. Commun. 97, 52–56 (2019)
Tarantino, A.M., Lanzoni, L., Falope, F.O.: The Bending Theory of Fully Nonlinear Beams. Springer, Berlin (2019)
Tarantino, A.M.: Crack propagation in finite elastodynamics. Math. Mech. Solids 10, 577–601 (2005)
Tarantino, A.M.: Equilibrium paths of a hyperelastic body under progressive damage. J. Elast. 114, 225–250 (2014)
Lanzoni, L., Tarantino, A.M.: Damaged hyperelastic membranes. Int. J. Non-Linear Mech. 60, 9–22 (2014)
Lanzoni, L., Tarantino, A.M.: Equilibrium configurations and stability of a damaged body under uniaxial tractions. Z. Angew. Math. Phys. 66, 171–190 (2015)
Lanzoni, L., Tarantino, A.M.: A simple nonlinear model to simulate the localized necking and neck propagation. Int. J. Non-Linear Mech. 84, 94–104 (2016)
Pelliciari, M., Tarantino, A.M.: Equilibrium paths for Von Mises trusses in finite elasticity. J. Elast. (2019). https://doi.org/10.1007/s10659-019-09731-1. In press
Pelliciari, M., Tarantino, A.M.: Equilibrium paths of a three-bar truss in finite elasticity with an application to graphene. Math. Mech. Solids (2019). https://doi.org/10.1177/1081286519887470. In press
Acknowledgements
Financial support from the Italian Ministry of Education, University and Research (MIUR) in the framework of the Project PRIN "Modelling of constitutive laws for traditional and innovative building materials" (code 2017HFPKZY) is gratefully acknowledged.
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher’s Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Appendix: Expressions of Derivatives \(S_{X,X}\), \(S_{Y,Y}\) and \(S_{Z,Z}\)
Appendix: Expressions of Derivatives \(S_{X,X}\), \(S_{Y,Y}\) and \(S_{Z,Z}\)
The derivatives of the functions \(S_{J}=2 (\omega _{1}+I_{1}\, \omega _{2} )\lambda _{J}-2 \, \omega _{2}\, \lambda _{J}^{3}+2\, I_{3}\, \omega _{3}\, \frac{1}{\lambda _{J}}\), with \(J=X,Y\) and \(Z\), contained in the system (20), have the following expressions:
with \(\omega _{i}=\frac{\partial \omega }{\partial I_{i}}\), \(I_{i,K}=\frac{\partial I_{i}}{\partial K}\) and \(\omega _{i,K}=\frac{\partial }{\partial K} ( \frac{\partial \omega }{\partial I_{i}} )\) for \(i=1, 2, 3\), \(K=X, Y\) and Z. On the basis of the kinematic model, the following quantities can be computed:
For a Mooney-Rivlin material the following derivatives, contained in the previous equations, are calculated:
Rights and permissions
About this article
Cite this article
Lanzoni, L., Tarantino, A.M. Mechanics of High-Flexible Beams Under Live Loads. J Elast 140, 95–120 (2020). https://doi.org/10.1007/s10659-019-09759-3
Received:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10659-019-09759-3