Skip to main content
SpringerLink
Log in

Symmetry breaking-induced state-dependent aging and chimera-like death state

  • Original paper
  • Published:
Nonlinear Dynamics Aims and scope Submit manuscript

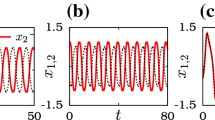
Abstract

Aging transition refers to the manifestation of the homogeneous steady state beyond a critical ratio of the inactive oscillators in a network of active and inactive oscillators. In contrast, symmetry breaking in coupled dynamical systems always results in heterogeneous states. In this work, surprisingly, we find that the symmetry breaking coupling facilitates the onset of the homogeneous steady state among the population at the critical proportion of the inactive oscillators despite the presence of a large number of the active oscillators. Further, increase in the natural frequency of the oscillation and the number of the inactive oscillators are conducive to the onset of the aging transition. Interestingly, chimera-like death state is observed in the study related to the aging transition for the first time in the literature in addition to the actual chimera death states among the active oscillator for the random initial conditions. The critical curves corresponding to the stability of the aging transition and the chimera-like death states are deduced, which agrees perfectly with the simulation results. Even a feeble decrease in the feedback factor in the coupling destabilizes the stable aging state thereby facilitating the oscillatory state in the entire parameter space despite the presence of a large proportion of the inactive oscillators increasing the robustness of the network against disorders.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11

Similar content being viewed by others

References

  1. Pikovsky, A., Rosenblum, M.G., Kurths, J.: Synchronization: A Universal Concept in Nonlinear Sciences. Cambridge University Press, Cambridge (2001)

    MATH  Google Scholar 

  2. Suresh, R., Senthilkumar, D.V., Lakshmanan, M., Kurths, J.: Global and partial phase synchronizations in arrays of piecewise linear time-delay systems. Int. J. Bifurc. Chaos 22, 1250178 (2012)

    MATH  Google Scholar 

  3. Kapitaniak, M., Czolczynski, K., Perlikowski, P., Stefanski, A., Kapitaniak, T.: Synchronous states of slowly rotating pendula. Phys. Rep. 541, 1–44 (2014)

    MathSciNet  MATH  Google Scholar 

  4. Pecora, L.M., Sorrentino, F., Hagerstrom, A.M., Murphy, T.E., Roy, R.: Cluster synchronization and isolated desynchronization in complex networks with symmetries. Nat. Commun. 5, 4079 (2014)

    Google Scholar 

  5. Panaggio, M.J., Abrams, D.M.: Chimera states: coexistence of coherence and incoherence in networks of coupled oscillators. Nonlinearity 28, R67 (2015)

    MathSciNet  MATH  Google Scholar 

  6. Kalle, P., Sawicki, J., Zakharova, A., Schöll, E.: Chimera states and the interplay between initial conditions and non-local coupling. Chaos 27, 033110 (2017)

    Google Scholar 

  7. Mishra, A., Saha, S., Roy, P.K., Kapitaniak, T., Dana, S.K.: Multicluster oscillation death and chimeralike states in globally coupled Josephson Junctions. Chaos 27, 023110 (2017)

    MathSciNet  Google Scholar 

  8. Banerjee, T., Biswas, D., Ghosh, D., Schöll, E., Zakharova, A.: Networks of coupled oscillators: from phase to amplitude chimeras. Chaos 28, 113124 (2018)

    MathSciNet  MATH  Google Scholar 

  9. Sathiyadevi, K., Chandrasekar, V.K., Senthilkumar, D.V., Lakshmanan, M.: Imperfect amplitude mediated chimera states in a nonlocally coupled network front. Appl. Math. Stat. 4, 58 (2018)

    Google Scholar 

  10. Sathiyadevi, K., Chandrasekar, V.K., Senthilkumar, D.V., Lakshmanan, M.: Distinct collective states due to trade-off between attractive and repulsive couplings. Phys. Rev. E 97, 032207 (2018)

    Google Scholar 

  11. Saxena, G., Prasad, A., Ramaswamy, R.: Amplitude death: the emergence of stationarity in coupled nonlinear systems. Phys. Rep. 521, 205–228 (2012)

    Google Scholar 

  12. Banerjee, T., Ghosh, D.: Experimental observation of a transition from amplitude to oscillation death in coupled oscillators. Phys. Rev. E 89, 062902 (2014)

    Google Scholar 

  13. Banerjee, T., Ghosh, D.: Transition from amplitude to oscillation death under mean-field diffusive coupling. Phys. Rev. E 89, 052912 (2014)

    Google Scholar 

  14. Zou, W., Senthilkumar, D.V., Duan, J., Kurths, J.: Emergence of amplitude and oscillation death in identical coupled oscillators. Phys. Rev. E 90, 032906 (2014)

    Google Scholar 

  15. Pahwa, S., Scoglio, C., Scala, A.: Abruptness of cascade failures in power grids. Sci. Rep. 4, 3694 (2014)

    Google Scholar 

  16. Daido, H., Nakanishi, K.: Aging and clustering in globally coupled oscillators. Phys. Rev. E 75, 056206 (2007)

    MathSciNet  Google Scholar 

  17. Daido, H., Nakanishi, K.: Aging transition and universal scaling in oscillator networks. Phys. Rev. Lett. 93, 104101 (2004)

    Google Scholar 

  18. Daido, H.: Suppression and recovery of spatiotemporal chaos in a ring of coupled oscillators with a single inactive site. Europhys. Lett. 87, 40001 (2009)

    Google Scholar 

  19. Daido, H.: Dynamics of a large ring of coupled active and inactive oscillators. Phys. Rev. E 83, 026209 (2011)

    Google Scholar 

  20. Huang, W., Zhang, X., Hu, X., Zou, Y., Liu, Z., Guan, S.: Variation of critical point of aging transition in a networked oscillators system. Chaos 24, 023122 (2014)

    MATH  Google Scholar 

  21. Tanaka, G., Morino, K., Daido, H., Aihara, K.: Dynamical robustness of coupled heterogeneous oscillators. Phys. Rev. E 89, 052906 (2014)

    Google Scholar 

  22. Daido, H.: Aging transition and disorder-induced coherence in locally coupled oscillators. Europhys. Lett. 84, 10002 (2008)

    MathSciNet  Google Scholar 

  23. Morino, K., Tanaka, G., Aihara, K.: Robustness of multilayer oscillator networks. Phys. Rev. E 83, 056208 (2011)

    Google Scholar 

  24. Thakur, B., Sharma, D., Sen, A.: Time-delay effects on the aging transition in a population of coupled oscillators. Phys. Rev. E 90, 042904 (2014)

    Google Scholar 

  25. Kundu, S., Majhi, S., Karmakar, P., Ghosh, D., Rakshit, B.: Augmentation of dynamical persistence in networks through asymmetric interaction. Euro. Phys. Lett. 123, 30001 (2018)

    Google Scholar 

  26. Kundu, S., Majhi, S., Ghosh, D.: Resumption of dynamism in damaged networks of coupled oscillators. Phys. Rev. E 97, 052313 (2018)

    Google Scholar 

  27. Sun, S., Ma, N., Xu, W.: Aging transition by random errors. Sci. Rep. 7, 42715 (2017)

    Google Scholar 

  28. Zakharova, A., Kapeller, M., Schöll, E.: Chimera death: symmetry breaking in dynamical networks. Phys. Rev. Lett. 112, 154101 (2014)

    Google Scholar 

  29. Premalatha, K., Chandrasekar, V.K., Senthilvelan, M., Lakshmanan, M.: Impact of symmetry breaking in networks of globally coupled oscillators. Phys. Rev. E 91, 052915 (2015)

    MathSciNet  Google Scholar 

  30. Zou, W., Senthilkumar, D.V., Nagao, R., Duan, J., Kurths, J.: Restoration of rhythmicity in diffusively coupled dynamical networks. Nat. Commun. 6, 7709 (2015)

    Google Scholar 

  31. Liu, Y., Zou, W., Zhan, M., Duan, J., Kurths, J.: Enhancing dynamical robustness in aging networks of coupled nonlinear oscillators. Europhys. Lett. 114, 40004 (2016)

    Google Scholar 

  32. Mukherjee, R., Sen, A.: Amplitude mediated chimera states with active and inactive oscillators. Chaos 28, 053109 (2018)

    MathSciNet  Google Scholar 

  33. Chandrasekar, V.K., Karthiga, S., Lakshmanan, M.: Feedback as a mechanism for the resurrection of oscillations from death states. Phys. Rev. E 92, 012903 (2015)

    MathSciNet  Google Scholar 

  34. Senthilkumar, D.V., Suresh, K., Chandrasekar, V.K., Zou, W., Dana, S.K., Thamilmaran, K., Kurths, J.: Experimental demonstration of revival of oscillations from death in coupled nonlinear oscillators. Chaos 26, 043112 (2016)

    Google Scholar 

  35. Deng, T., Liu, W., Xiao, J., Kurth, J.: Conjugate coupling-induced symmetry breaking and quenched oscillations. Choas 26, 094813 (2016)

    Google Scholar 

  36. Koseska, A., Volkov, E., Kurths, J.: Transition from amplitude to oscillation death via turing bifurcation. Phys. Rev. Lett. 111, 024103 (2013)

    Google Scholar 

  37. Punetha, N., Varshney, V., Sahoo, S., Saxena, G., Prasad, A., Ramaswamy, R.: Dynamical effects of breaking rotational symmetry in counter-rotating Stuart–Landau oscillators. Phys. Rev. E 98, 022212 (2018)

    Google Scholar 

  38. Daido, H.: Multibranch entrainment and scaling in large populations of coupled oscillators. Phys. Rev. Lett. 77, 1406–1409 (1996)

    Google Scholar 

  39. Kuramoto, Y., Nakao, H.: Origin of power-law spatial correlations in distributed oscillators and maps with nonlocal coupling. Phys. Rev. Lett. 76, 4352 (1996)

    Google Scholar 

  40. Kuramoto, Y., Nakao, H.: Power-law spatial correlations and the onset of individual motions in self-oscillatory media with non-local coupling. Physica D 103, 294–313 (1997)

    MATH  Google Scholar 

  41. Kuramoto, Y., Battogtokh, D., Nakao, H.: Multiaffine chemical turbulence. Phys. Rev. Lett. 81, 3543 (1998)

    Google Scholar 

  42. Kuramoto, Y., Battogtokh, D.: Coexistence of coherence and incoherence in nonlocally coupled phase oscillators. Nonlinear Phenom. Complex Syst. 5, 380 (2002)

    Google Scholar 

  43. Schmidt, L., Schönleber, K., Krischer, K., García-Morales, V.: Coexistence of synchrony and incoherence in oscillatory media under nonlinear global coupling. Chaos 24, 013102 (2014)

    MathSciNet  Google Scholar 

  44. Thompson, M.C., Gal, P.L.: The Stuart–Landau model applied to wake transition revisited. Eur. J. Mech. B Fluids 23, 219–228 (2004)

    MathSciNet  MATH  Google Scholar 

  45. Frasca, M., Bergner, A., Kurths, J., Fortuna, L.: Bifurcations in a star-like network of Stuart–Landau oscillators. Int. J. Bifurc. Chaos 22, 1250173 (2012)

    MATH  Google Scholar 

  46. Moon, J.-Y., Lee, U., Blain-Moraes, S., Mashour, G.A.: General relationship of global topology, local dynamics, and directionality in large-scale brain networks. PLoS Comput. Biol. 11, e1004225 (2015)

    Google Scholar 

  47. Initial condition is chosen as \((x_j , y_j) \in (0, 0)\) for \(j=1, 2, \ldots , N-1\) and \((x_N , y_N) \in (\pm u, 0)\) for uniform random number \(u\) between \(0\) and \(1\)

  48. Initial condition is chosen as \((x_j , y_j) \in (u, -v)\) if \(j\) is odd and \((x_j , y_j) \in (-u, v)\) if \(j\) is even or vice versa for uniform random numbers \(u\) and \(v\) between \(0\) and \(1\)

  49. Loos, S.A.M., Claussen, J.C., Schöll, E., Zakharova, A.: Chimera patterns under the impact of noise. Phys. Rev. E 93, 012209 (2016)

    Google Scholar 

  50. Sathiyadevi, K., Chandrasekar, V.K., Senthilkumar, D.V.: Stable amplitude chimera in a network of coupled Stuart–Landau oscillators. Phys. Rev. E 98, 032301 (2018)

    MathSciNet  Google Scholar 

  51. Ermentrout, B.: Simulating, Analyzing and Animating Dynamical Systems: A Guide to Xppaut for Researchers and Students (Software, Environments, Tools). SIAM Press, Philadelphia, PA (2002)

    MATH  Google Scholar 

Download references

Acknowledgements

IG wishes to thank SASTRA Deemed University for research fund and extending infrastructure support to carry out this work. KS and DVS is supported by the CSIR EMR Grant No. 03(1400)/17/EMR-II. The work of VKC forms part of a research project sponsored by CSIR Project under Grant No. 03(1444)/18/EMR-II.

Author information

Authors and Affiliations

  1. Centre for Nonlinear Science and Engineering, School of Electrical and Electronics Engineering, SASTRA Deemed University, Thanjavur, 613 401, India

    I. Gowthaman & V. K. Chandrasekar

  2. School of Physics, Indian Institute of Science Education and Research, Thiruvananthapuram, Kerala, 695551, India

    K. Sathiyadevi & D. V. Senthilkumar

Authors
  1. I. Gowthaman
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. K. Sathiyadevi
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. V. K. Chandrasekar
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. D. V. Senthilkumar
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding authors

Correspondence to V. K. Chandrasekar or D. V. Senthilkumar.

Ethics declarations

Conflict of interest

The authors declare no conflict of interest.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Appendices

Appendix A: Aging state

The coefficients \(\beta _0,~\beta _1,~\beta _2,\) and \(\beta _3\) for the above characteristic eigenvalue equation (3) are given by

$$\begin{aligned} \beta _0= & {} (a^2+\omega ^2)\beta _{01}+ak\beta _{02}, \nonumber \\ \beta _1= & {} b^2k(p-1+\alpha )+a^2\beta _{11}+\varepsilon \beta _{12}\omega ^2+b\beta _{13}, \nonumber \\ \beta _2= & {} a^2+b^2-\varepsilon ^2\alpha (1-\alpha )+a \beta _{21}+\beta _{22}, \nonumber \\ \beta _3= & {} 2(b-a)+\varepsilon (2\alpha -1), \end{aligned}$$
(11)

where

$$\begin{aligned} \beta _{01}= & {} b^2+b\varepsilon (\alpha -p)+\omega ^2, \\ \beta _{02}= & {} a\varepsilon (b\varepsilon \alpha (\alpha -1)+(b^2+1)(p-1+\alpha )),\\ \beta _{11}= & {} (2 b + \varepsilon (-p + \alpha )),\\ \beta _{12}= & {} (2\alpha -1),\\ \beta _{13}= & {} \varepsilon ^2 (-1 + \alpha ) \alpha + 2 \omega ^2,\\ \beta _{21}= & {} -4b+\varepsilon (1+p-3\alpha ), \\ \beta _{22}= & {} b\varepsilon (p-2+3\alpha )+3\omega ^2. \end{aligned}$$

Appendix B: Chimeralike state

The coefficients \(\gamma _0,~\gamma _1,~\gamma _2,~\gamma _3,~\gamma _4,\) and \(\gamma _5\) for the above characteristic eigenvalue equation (3) are given by

$$\begin{aligned} \gamma _0= & {} \gamma _{01}+(b\varepsilon \gamma _{02}+a^2\gamma _{03}+(b^2+\omega ^2)\gamma _{04}-\gamma _{05}) , \nonumber \\ \gamma _1= & {} a^4\gamma _{11}+a^3 \gamma _{12} +a^2 \gamma _{13}-a\gamma _{14}+b^2\gamma _{15}-b\gamma _{16}+\gamma _{17}, \nonumber \\ \gamma _2= & {} a^4+a^3\gamma _{21} + a^2\gamma _{22}-a\gamma _{23}+b^2\gamma _{24}+b\gamma _{25}+\gamma _{26}, \nonumber \\ \gamma _3= & {} 4a^3-3a^2 \gamma _{31}+a \gamma _{32}-b^2\gamma _{33}-b\gamma _{34}+\gamma _{35},\nonumber \\ \gamma _4= & {} 6 a^2+a \gamma _{41}+\gamma _{42}, \nonumber \\ \gamma _5= & {} 8 r^* -2(2 a + b) - \varepsilon + 3 \varepsilon \alpha , \end{aligned}$$
(12)

where

$$\begin{aligned} \gamma _{01}= & {} a^2+\omega ^2+3r^{*2}+\varepsilon \alpha ({A_1^{(1)}}^2+3{A_1^{(2)}}^2)-a(4r^*_\varepsilon \alpha ), \\ \gamma _{02}= & {} (\alpha -p) (\omega ^2 + 3 r^{*2}) + \varepsilon ({A_1^{(1)}}^2+3{A_1^{(2)}}^2) (\alpha -1)\alpha , \\ \gamma _{03}= & {} (b^2 + \omega ^2 + b \varepsilon ( \alpha -p)), \\ \gamma _{04}= & {} (\omega ^2 + 3 r^{*2} + \varepsilon ({A_1^{(1)}}^2+3{A_1^{(2)}}^2) ( p + \alpha -1)), \\ \gamma _{05}= & {} a(4r^* \gamma _{03}+\varepsilon (\alpha -1)\alpha +(\omega ^2+b^2)(p-1+\alpha )), \\ \gamma _{11}= & {} 2 b + \varepsilon (\alpha -p), \\ \gamma _{12}= & {} -4 b^2 - 4 \omega ^2 + 2 b (-8 r^* + \varepsilon (1 + p - 4 \alpha )) + \\&\varepsilon \alpha (\varepsilon - 8 r^* - 2 \varepsilon \alpha ) + \varepsilon p (8r^* + \varepsilon \alpha ), \\ \gamma _{13}= & {} 24 \omega ^2 r^* - 22 \varepsilon r^{*2} (p - \alpha ) - \varepsilon ^2 (5 {A_1^{(1)}}^2 + 7 {A_1^{(2)}}^2)\\&(1 + p - 2 \alpha ) \alpha + \varepsilon ^3 (-1 + \alpha ) \alpha ^2 + \varepsilon \omega ^2 (p-3 \\&+ 8 \alpha ) + 3 b^2 (8 r^* + \varepsilon (-1 + p + 2 \alpha )) +b (4 \omega ^2 \\&+ 44 r^{*2} - 2 \varepsilon ({A_1^{(2)}}^2 (7 + 5 p - 26 \alpha ) + {A_1^{(1)}}^2 (5 + 7 p \\&- 22 \alpha )) + \varepsilon ^2 \alpha (-5 - p + 8 \alpha )), \\ \gamma _{14}= & {} 4 \omega ^4 + 2 b^2 (2 \omega ^2 + 22 r^{*2} + \varepsilon ^2 \alpha ( p-1 + \alpha ) + \\&\varepsilon (5 {A_1^{(1)}}^2 + 7 {A_1^{(2)}}^2) ( p-1 + 2 \alpha )) + 2 b (8 r (\omega ^2 + 3 r^2) \\&- \varepsilon r ({A_1^{(2)}}^2 (15 + 7 p - 52 \alpha ) + {A_1^{(1)}}^2 (7 + 15 p - 36 \alpha )) \\&+ \omega ^2 (1 + p - 4 \alpha )) + \varepsilon ^3 ( \alpha -1) \alpha ^2 + \varepsilon ^2 \alpha (-{A_1^{(2)}}^2 \\&(13 + p - 20 \alpha ) + {A_1^{(1)}}^2 ( 12 \alpha -7 - 3 p ))) + \omega ^2 \\&(44 r^2 + \varepsilon ^2 \alpha (-3 + p + 4 \alpha ) + 2 \varepsilon ({A_1^{(1)}}^2 ( p + 14 \alpha \\&-5 ) + {A_1^{(2)}}^2 ( 3 p + 18 \alpha -7))) + \varepsilon (\alpha (24 r^3 + 2 \varepsilon ^2 \\&({A_1^{(1)}}^2 + 3 {A_1^{(2)}}^2) ( \alpha -1) \alpha + \varepsilon (7 {A_1^{(1)}}^4 + 22 {A_1^{(1)}}^2 \\&{A_1^{(2)}}^2 + 15 {A_1^{(2)}}^4) ( 2 \alpha -1)) - p r (24 {A_1^{(1)}}^4 +\\&3 {A_1^{(2)}}^2 (8 {A_1^{(2)}}^2 + 5 \varepsilon \alpha ) + {A_1^{(1)}}^2 (48 {A_1^{(2)}}^2 + 7 \varepsilon \alpha ))), \\ \gamma _{15}= & {} 8r^* (\omega ^2 + 3 r^{*2}) + 2\varepsilon ^2 ({A_1^{(1)}}^2 + 3 {A_1^{(2)}}^2) \alpha ( p-1 + \alpha )\\&+ \varepsilon (\omega ^2 + 7 {A_1^{(1)}}^4 + 22 {A_1^{(1)}}^2 {A_1^{(2)}}^2 + 15 {A_1^{(2)}}^4) ( p-1 + 2 \alpha ), \\ \gamma _{16}= & {} 2 \omega ^4 + 18 r^{*4} - 6 \varepsilon r^{*2} ({A_1^{(2)}}^2 (3 + p - 10 \alpha ) + {A_1^{(1)}}^2 \\ \end{aligned}$$
$$\begin{aligned}&(1 + 3 p - 6 \alpha )) + 2 \varepsilon ^3 ({A_1^{(1)}}^2 + 3 {A_1^{(2)}}^2) (\alpha -1) \alpha ^2 - \\&\omega ^2 (-12 r^{*2} + 2 \varepsilon ({A_1^{(2)}}^2 (3 + p - 10 \alpha ) + {A_1^{(1)}}^2 (1 +\\&3 p - 6 \alpha )) + \varepsilon ^2 (1 + p - 2 \alpha ) \alpha ) + \varepsilon ^2 \alpha (-2 {A_1^{(1)}}^2 \\&{A_1^{(2)}}^2 (17 + 5 p - 28\alpha ) + {A_1^{(1)}}^4 ( 16 \alpha -9 - 5 p) + 3 {A_1^{(2)}}^4\\&( p + 16 \alpha -11)), \\ \gamma _{17}= & {} 8 \omega ^2 r^* (\omega ^2 + 3 r^{*2}) + \varepsilon ^3 ({A_1^{(1)}}^2 + 3 {A_1^{(2)}}^2)^2 (\alpha -1)\alpha ^2 \\&+ \varepsilon ^2 ({A_1^{(1)}}^2 + 3 {A_1^{(2)}}^2) \alpha (-3 r^{*2} (1 + p - 2 \alpha ) + \omega ^2\\&( p + 4\alpha -3)) + \varepsilon (-9 r^{*4} (p - \varepsilon ) + \omega ^4 ( 3 \alpha -1) \\&+ \omega ^2 r^* (3 {A_1^{(2)}}^2 (3 p + 12\alpha -5) + {A_1^{(1)}}^2 ( p + 20 \alpha -7))),\\ \gamma _{21}= & {} (\varepsilon (1 + 3 p - 6 \alpha )-8 b - 8r^* ), \\ \gamma _{22}= & {} 22r^*+6 b (8 r^* + \varepsilon (-1 + 3 \alpha )) +(34 \varepsilon \alpha -5 \varepsilon - \\&19 \varepsilon p ) {A_1^{(1)}}^2 +(38 \varepsilon \alpha - 7 \varepsilon - 17 \varepsilon p ){A_1^{(2)}}^2 +6 b^2 + 8 w^2 \\&- 4 \varepsilon ^2 \alpha - 2 \varepsilon ^2 p \alpha + 7 \varepsilon ^2 \alpha ^2, \\ \gamma _{23}= & {} 8 r^* (4 \omega ^2 + 3 r^{*2}) + 2 \varepsilon ^3 (-1 + \alpha ) \alpha ^2 + 3 b^2 \\&(8 r^* + \varepsilon (-1 + p + 2 \alpha )) + k (-r^* ({A_1^{(2)}}^2 (15 + \\&29 p - 74 \alpha ) + {A_1^{(1)}}^2 (7 + 37 p - 58 \alpha )) + 4 \omega ^2 \\&(-1 + 3 \alpha )) + 2 \varepsilon ^2 \alpha ({A_1^{(1)}}^2(-6 - 4 p + 11 \alpha ) + \\&{A_1^{(2)}}^2 (-10 - 4 p + 17\alpha )) + b (8 \omega ^2 + 88 r^2 - 4 \varepsilon \\&{A_1^{(1)}}^2 (5 + p - 16 \alpha ) + \varepsilon ^2 \alpha (-7 + p + 10\alpha ) + 4 \\&\varepsilon {A_1^{(2)}}^2 (-7 + p + 20 \alpha )), \\ \gamma _{24}= & {} (2 \omega ^2 + 22 r^{*2} + \varepsilon ^2 \alpha (-1 + p + \alpha ) + \varepsilon (5 {A_1^{(1)}}^2 \\&+ 7 {A_1^{(2)}}^2) (-1 + p + 2 \alpha )), \\ \gamma _{25}= & {} 16 r^* (\omega ^2 + 3 r^{*2}) + \varepsilon ^3 (-1 + \alpha ) \alpha ^2 + 2 \varepsilon (-r^* \\&({A_1^{(2)}}^2 (15 - 4 p - 41 \alpha ) + {A_1^{(2)}}^2 (7 + 4 p - 25\alpha )) +\\&\omega ^2 (-1 + 3 \alpha )) + \varepsilon ^2 \alpha (-{A_1^{(1)}}^2 (9 + p - 14 \alpha ) + \\&{A_1^{(2)}}^2 (-19 + 5 p + 26 \alpha )), \\ \gamma _{26}= & {} 3 \omega ^4 + 9 r^{*4} - 3 \varepsilon r^{*2} ({A_1^{(2)}}^2 (3 + 5 p - 14 \alpha ) + {A_1^{(1)}}^2 \\&(1 + 7 p - 10 \alpha )) + 2 \varepsilon ^3 ({A_1^{(1)}}^2 + 3 {A_1^{(2)}}^2) (-1 + \alpha )\\&\alpha ^2 + \varepsilon ^2 \alpha (-2 {A_1^{(1)}}^2 {A_1^{(2)}}^2 (14 + 8 p - 25 \alpha ) - 3 {A_1^{(2)}}^4 \\&(8 + 2 p - 13\alpha ) + {A_1^{(1)}}^4 (-8 - 6 p + 15 \alpha )) + \\&\omega ^2 (28 r^{*2} + \varepsilon ^2 \alpha (-2 + 3 \alpha ) - 2 k ({A_1^{(1)}}^2 (3 + p\\&- 10 \alpha ) - {A_1^{(2)}}^2 (-5 + p + 14 \alpha ))), \end{aligned}$$
$$\begin{aligned} \gamma _{31}= & {} (4 b + 8 r^* - \varepsilon (1 + p - 4 \alpha )), \\ \gamma _{32}= & {} -a (4 b^2 + 8\omega ^2 - 10 \varepsilon {A_1^{(1)}}^2 - 14 \varepsilon p {A_1^{(1)}}^2 22r^{*2} - 14 k {A_1^{(2)}}^2 \\&- 10 \varepsilon p {A_1^{(2)}}^2 + 48 b r^* - 5 \varepsilon ^2 \alpha - \varepsilon ^2 p \alpha + 44 \varepsilon {A_1^{(1)}}^2 \alpha + \\&52 \varepsilon {A_1^{(2)}}^2 \alpha + 8 \varepsilon ^2 \alpha ^2 + 2 b \varepsilon (-3 + p + 8 \alpha )), \\ \gamma _{33}= & {} 8 r^* + \varepsilon (p-1 + 2\alpha ), \\ \gamma _{34}= & {} (4 \omega ^2 + 44 r^{*2} + \varepsilon ^2 \alpha (-3 + p + 4 \alpha ) + 2 \varepsilon ({A_1^{(1)}}^2 (-5 \\&+ p + 14 \alpha ) + {A_1^{(2)}}^2 (3 p-7 + 18 \alpha ))), \\ \gamma _{35}= & {} (16 \omega ^2 - 22 \varepsilon {A_1^{(1)}}^2 - 22 \varepsilon p {A_1^{(1)}}^2 + 72 {A_1^{(1)}}^4 - 13 \varepsilon ^2 \alpha - \\&\varepsilon ^2 p \alpha + 88 \varepsilon {A_1^{(1)}}^2 \alpha + 20 \varepsilon ^2 \alpha ^2) {A_1^{(1)}}^2 + (16 \omega ^2 - 22 \varepsilon {A_1^{(1)}}^2\\&- 22 \varepsilon p {A_1^{(1)}}^2 + 72 {A_1^{(1)}}^4 - 13 \varepsilon ^2 \alpha - \varepsilon ^2 p \alpha + 88 \varepsilon {A_1^{(1)}}^2\alpha +\\&20 \varepsilon ^2 \alpha ^2) {A_1^{(2)}}^2 -2 \varepsilon \omega ^2 + 6 \varepsilon \omega ^2 \alpha - \varepsilon ^3 \alpha ^2 + \varepsilon ^3 \alpha ^3,\\ \gamma _{41}= & {} \varepsilon (3 + p - 10 \alpha )-8 b - 24 r^*, \\ \gamma _{42}= & {} 22r^{*2} +16br^*+(18\alpha - 3 p-5 )\varepsilon {A_1^{(1)}}^2 + (22\alpha -p \\&-7)\varepsilon {A_1^{(2)}}^2+6 a^2 + b^2 + 3 w^2 - 2 k^2 \alpha + 3 \varepsilon ^2 \alpha ^2 + \\&b \varepsilon (p-2 + 5 \alpha );~ r^*=(A_1^{(1)*}+A_2^{(1)*}). \end{aligned}$$

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Gowthaman, I., Sathiyadevi, K., Chandrasekar, V.K. et al. Symmetry breaking-induced state-dependent aging and chimera-like death state. Nonlinear Dyn 101, 53–64 (2020). https://doi.org/10.1007/s11071-020-05766-5

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11071-020-05766-5

Keywords

Search

Navigation