Proton–proton fusion in new pionless EFT power counting

Abstract

The zero-energy astrophysical S-factor for the fusion process $p+p\rightarrow d+e^{+}+\nu _{e}$ is calculated using pionless effective field theory ($~/\!\!\pi$EFT). In the present study, the order-by-order results are reproduced according to a new suggested power counting. The short range interactions in the S-wave pp scattering at leading order and the corrections in the next-to-leading order including the Coulomb interaction are introduced. In addition the Coulomb interaction between the incoming protons has been considered. The new nuclear $~/\!\!\pi$EFT amplitude is compatible with renormalization-group invariance.

Appendix A: The calculation of the LO amplitude

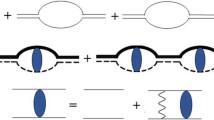
We present here a brief LO calculation of $^{1}S_{0}$ state of np scattering amplitude. The LO inverse amplitude by taking the two-dibaryon fields derives as below,

$$\begin{aligned} \begin{aligned} \left[ \frac{m_{N}}{4\pi }T(k;\varLambda )\right] ^{-1}&=\frac{4\pi }{m_{N}y_{s}^{2}} \\&\quad \times \frac{[\varDelta _{1}(\varLambda )+\frac{c_{1}(\varLambda )}{m_{N}}k^{2}][\varDelta _{2}(\varLambda )+\frac{c_{2}(\varLambda )}{m_{N}}k^{2}]}{\varDelta _{1}(\varLambda )+\varDelta _{2}(\varLambda ) +\frac{[c_{1}(\varLambda )+c_{2}(\varLambda )]}{m_{N}}k^2} \\&\quad +ik+\theta _{1}\varLambda +\theta _{-1}\frac{k^2}{\varLambda } +{\mathcal {O}}\left( \frac{k^{4}}{\varLambda ^{3}}\right) . \end{aligned} \end{aligned}$$

(A.1)

If here k is the smallest scale than the other scales, at large cutoff, Eq. (A.1) is equal to ERE

$$\begin{aligned} \left[ \frac{m_{N}}{4\pi }T(k;\varLambda )\right] ^{-1}=\frac{1}{a}+ik-\frac{r_{0}}{2}k^{2}-\frac{P_{0}}{4}k^{4}+\cdots , \end{aligned}$$

(A.2)

such that, for np scattering, The scattering length is $a \simeq$$-23.7$ fm $\simeq$ − (8 MeV)$^{-1}$ [74]. and the effective range is $r_{0}\simeq$ 2.7 fm $\simeq$(73 MeV)$^{-1}$ [64], Also, The shape parameter is $P_{0}\simeq$2.0 $\hbox {fm}^{3}$$\simeq$ (158 MeV)$^{-3}$ [65] and $k_{0} \simeq$340 MeV [54] is the scattering momentum. Because of the anomalously large value of $\mid a \mid$ that results a virtual bound state too close to threshold, its binding momentum corresponds to small scale $\aleph \sim$ 10 MeV. The suggestion of an enlarged range of validity of $~/\!\!\pi$EFT is introduced, with the low momentum scale $M_{lo}\sim m_{\pi }\sim Q$ and the EFT breakdown scale, $M_{hi} \le M_{QCD}\sim$1 GeV , so that $\aleph$ appears at NLO [66, 75]. Therefore phenomenological parameters of the theory rescaled as

$$\begin{aligned} \frac{1}{a}={\mathcal {O}}\left( \frac{M_{l0}^{2}}{M_{hi}}\right) ,\quad k_{0}\sim \frac{1}{r_{0}}\sim \frac{1}{P_{0}^{1/3}}\sim \cdots ={\mathcal {O}}(M_{lo}). \end{aligned}$$

(A.3)

the amplitude can be taken in the following form:

$$\begin{aligned} T(k;\varLambda )=T^{[0]}(k;\varLambda )+T^{[1]}(k;\varLambda )+\cdots , \end{aligned}$$

(A.4)

so that the high indexes (inside the bracket) show the order of the observables and,

$$\begin{aligned} T^{[0]}(k;\varLambda )&=V^{[0]}(k;\varLambda )\Bigg [1+\frac{m_{N}}{4\pi }V^{[0]}(k;\varLambda )\Bigg (ik \nonumber \\&\quad +\theta _{1}\varLambda +\frac{k^{2}}{ \varLambda } \times \sum _{n=0}^{\infty }\theta _{-1-2n}\frac{k^{2n}}{\varLambda ^{2n}}\Bigg )\Bigg ], \end{aligned}$$

(A.5)

$$\begin{aligned} \frac{m_{N}}{4\pi }V^{[0]}(k;\varLambda )&=\frac{m_{N}y_{s}^{2}}{4\pi }\sum _{j}\Bigg (\varDelta _{j}^{[0]}(\varLambda )+\frac{c_{j}^{[0]}(\varLambda )}{m_{N}}k^{2}\Bigg )^{-1},\qquad \qquad \end{aligned}$$

(A.6)

$$\begin{aligned} T^{[1]}(k;\varLambda )&=\Bigg (\frac{T^{[0]}(k;\varLambda )}{V^{[0]}(k;\varLambda )}\Bigg )^{2}V^{[1]}(k;\varLambda ),\qquad \qquad \qquad \qquad \end{aligned}$$

(A.7)

$$\begin{aligned} \frac{m_{N}}{4\pi }V^{[1]}(k;\varLambda )&=-\frac{m_{N}y_{s}^{2}}{4\pi }\sum _{j}\Bigg (\varDelta _{j}^{[0]}(\varLambda )+\frac{c_{j}^{[0]}(\varLambda )}{m_{N}}k^{2}\Bigg )^{-2} \nonumber \\&\quad \times \Bigg (\varDelta _{j}^{[1]}(\varLambda )+\frac{c_{j}^{[1]}(\varLambda )}{m_{N}}k^{2}\Bigg ). \end{aligned}$$

(A.8)

Since $1{/}\mid a \mid$ is very small, it imposes an expansion of the NN $^{1}S_{0}$ amplitude around the unitary limit [66, 75] and it takes as below:

$$\begin{aligned} \frac{1}{a^{[0]}}=0. \end{aligned}$$

(A.9)

According to Eq. (A.1), for reproducing the zero amplitude at LO requires a minimum of three non-vanishing bare parameters and scales the following form

$$\begin{aligned} \begin{aligned} {\bar{\varDelta }}_{1}^{[0]}&={\mathcal {O}}(M_{lo}) , \quad \frac{{\bar{c}}_{1}^{[0]}}{m_{N}}=0 ,\quad {\bar{\varDelta }}_{2}^{[0]}=0 , \\ \frac{{\bar{c}}_{2}^{[0]}}{m_{N}}&={\mathcal {O}}\left( \frac{1}{M_{lo}}\right) ,\qquad \qquad \qquad \qquad \qquad \qquad \qquad \qquad \qquad \end{aligned} \end{aligned}$$

(A.10)

for relating three non-vanishing LO bare parameters of the two dibaryon fields, ${\varDelta }_{1}^{[0]}(\varLambda )$, ${\varDelta }_{2}^{[0]}(\varLambda ),$ and ${c}_{2}^{[0]}(\varLambda )$, to found observables, we introduce

$$\begin{aligned} F(z;\varLambda )\equiv \mathrm {Re}\Bigg [\frac{m_{N}}{4\pi }T^{[0]}(\sqrt{z};\varLambda )\Bigg ]^{-1}, \end{aligned}$$

(A.11)

and impose three renormalization conditions on it the following form:

$$\begin{aligned} F(z;\varLambda )=0 , \quad \frac{\partial F(z;\varLambda )}{\partial z}\arrowvert _{z=0}=-\frac{r_{0}}{2} ,\quad F^{-1}(k_{0}^{2};\varLambda )=0, \end{aligned}$$

(A.12)

so that, we derive three parameters of two-dibaryon fields as below

$$\begin{aligned} \varDelta _{1}^{[0]}(\varLambda )= & {} \frac{m_{N}y_{s}^{2}}{4\pi }({\bar{\varDelta }}_{1}^{[0]}-\theta _{1}\varLambda +\cdots ), \end{aligned}$$

(A.13)

$$\begin{aligned} \varDelta _{2}^{[0]}(\varLambda )= & {} \frac{m_{N}y_{s}^{2}}{4\pi }\Bigg (\frac{2\theta _{1}}{r_{0}^{3}k_{0}^{2}}\Bigg [\theta _{1}(r_{0}\varLambda )^{2}-\left( \frac{r_{0}^{2}k_{0}^{2}}{2}+2\theta _{1}\theta _{-1}\right) r_{0}\varLambda \nonumber \\&+4\theta _{1}\theta _{-1}^{2}+\cdots \Bigg ]\Bigg ), \end{aligned}$$

(A.14)

$$\begin{aligned} \frac{c_{2}^{[0]}(\varLambda )}{m_{N}}= & {} \frac{m_{N}y_{s}^{2}}{4\pi }\Bigg (\frac{{\bar{c}}_{2}^{[0]}}{m_{N}}-\frac{2\theta _{1}}{r_{0}^{3}k_{0}^{4}}\Bigg [\theta _{1}(r_{0}\varLambda )^{2} \nonumber \\&-\left( \frac{r_{0}^{2}k_{0}^{2}}{2}+2\theta _{1}\theta _{-1}\right) r_{0}\varLambda +4\theta _{1}\theta _{-1}^{2}+\cdots \Bigg ]\Bigg ), \end{aligned}$$

(A.15)

where two renormalized parameters are

$$\begin{aligned} {\bar{\varDelta }}_{1}^{[0]}=\frac{r_{0}k_{0}^{2}}{2} , \quad \frac{{\bar{c}}_{2}^{[0]}}{m_{N}}=-\frac{r_{0}}{2}.\qquad \qquad \end{aligned}$$

(A.16)

Also, the prediction for shape parameter at this order is given as below [55]

$$\begin{aligned} P_{0}^{[0]}(\varLambda )=\frac{2r_{0}}{k_{0}^2}\Bigg [1+\frac{2\theta _{-1}}{r_{0}\varLambda }+{\mathcal {O}}\left( \frac{k_{0}^{2}}{r_{0}\varLambda ^{3}}\right) \Bigg ], \end{aligned}$$

(A.17)

where $P_{0}^{[0]}k_{0}^{2}/(2r_{0})=1.03\pm 0.3$ that it has about 30$\%$ error from a careful analysis in Ref. [65].