Skip to main content
SpringerLink
Log in

Multisoliton solutions of the Degasperis–Procesi equation and its shortwave limit: Darboux transformation approach

  • Published:
Theoretical and Mathematical Physics Aims and scope Submit manuscript

Abstract

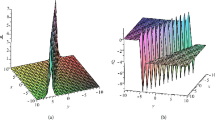
We propose a new approach for calculating multisoliton solutions of the Degasperis–Procesi equation and its shortwave limit by combining a reciprocal transformation with the Darboux transformation of the negative flow of the Kaup–Kupershmidt hierarchy. In particular, different specifications of the soliton parameters lead to two different types of soliton solutions of the Degasperis–Procesi equation.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Similar content being viewed by others

References

  1. A. Degasperis and M. Procesi, “Asymptotic integrability”, in: Symmetry and Perturbation Theory (Rome, Italy, 16-22 December 1998, A. Degasperis and G. Gaeta, eds.), World Scientific, Singapore (1999), pp. 23–37.

    Google Scholar 

  2. H. R. Dullin, G. A. Gottwald, and D. D. Holm, “Camassa-Holm, Korteweg-de Vries-5 and other asymptotically equivalent equations for shallow water waves”, Fluid Dynam. Res., 33, 73–95 (2003).

    Article  ADS  MathSciNet  Google Scholar 

  3. D. D. Holm and M. F. Staley, “Wave structure and nonlinear balances in a family of evolutionary PDEs”, SIAM J. Appl. Dyn. Syst., 2, 323–380 (2003).

    Article  ADS  MathSciNet  Google Scholar 

  4. A. Constantin and D. Lannes, “The hydrodynamical relevance of the Camassa-Holm and Degasperis-Procesi equations”, Arch. Ration. Mech. Anal., 192, 165–186 (2009).

    Article  MathSciNet  Google Scholar 

  5. A. Degasperis, D. D. Holm, and A. Hone, “A new integrable equation with peakon solutions”, Theor. Math. Phys., 133, 1463–1474 (2002).

    Article  MathSciNet  Google Scholar 

  6. R. Camassa and D. D. Holm, “An integrable shallow water equation with peaked solitons”, Phys. Rev. Lett., 71, 1661–1664 (1993); arXiv:patt-sol/9305002v1 (1993).

    Article  ADS  MathSciNet  Google Scholar 

  7. H. Lundmark and J. Szmigielski, “Multi-peakon solutions of the Degasperis-Procesi equation”, Inverse Problems, 19, 1241–1245 (2003); arXiv:nlin/0503033v1 (2005).

    Article  ADS  MathSciNet  Google Scholar 

  8. H. Lundmark and J. Szmigielski, “Degasperis-Procesi peakons and the discrete cubic string”, Int. Math. Res. Rap., 2005, 53–116 (2005).

    MathSciNet  MATH  Google Scholar 

  9. A. Constantin, R. I. Ivanov, and J. Lenells, “Inverse scattering transform for the Degasperis-Procesi equation”, Nonlinearity, 23, 2559–2575 (2010); arXiv:1205.4754v1 [nlin.SI] (2012).

    Article  ADS  MathSciNet  Google Scholar 

  10. A. Boutet de Monvel and D. Shepelsky, “A Riemann-Hilbert approach for the Degasperis-Procesi equation”, Nonlinearity, 26, 2081–2107 (2013).

    Article  ADS  MathSciNet  Google Scholar 

  11. A. Constantin and R. Ivanov, “Dressing method for the Degasperis-Procesi equation”, Stud. Appl. Math., 138, 205–226 (2017).

    Article  MathSciNet  Google Scholar 

  12. Y. Matsuno, “Multisolitons of the Degasperis-Procesi equation and their peakon limit”, Inverse Problems, 21, 1553–1570 (2005); arXiv:nlin/0511029v1 (2005).

    Article  ADS  MathSciNet  Google Scholar 

  13. Y. Matsuno, “The N-soliton solution of the Degasperis-Procesi equation”, Inverse Problems, 21, 2085–2101 (2005).

    Article  ADS  MathSciNet  Google Scholar 

  14. S. Stalin and M. Senthilvelan, “Multi-loop soliton solutions and their interaction in the Degasperis-Procesi equation”, Phys. Scr., 86, 015006 (2012); arXiv:1207.4634v1 [math-ph] (2012).

    Article  ADS  Google Scholar 

  15. J. K. Hunter and R. Saxton, “Dynamics of director fields”, SIAM J. Appl. Math., 51, 1498–1521 (1991).

    Article  MathSciNet  Google Scholar 

  16. J. K. Hunter and Y. Zheng, “On a completely integrable nonlinear hyperbolic variational equation”, Phys. D, 79, 361–386 (1994).

    Article  MathSciNet  Google Scholar 

  17. T. Schäfer and C. E. Wayne, “Propagation of ultra-short optical pulses in cubic nonlinear media”, Phys. D, 196, 90–105 (2004).

    Article  MathSciNet  Google Scholar 

  18. Y. Matsuno, “Smooth and singular multisoliton solutions of a modified Camassa-Holm equation with cubic nonlinearity and linear dispersion”, J. Phys. A: Math. Theor., 47, 125203 (2014); arXiv:1310.4011v2 [nlin.SI] (2013).

    Article  ADS  MathSciNet  Google Scholar 

  19. A. Sakovich and S. Sakovich, “The short pulse equation is integrable”, J. Phys. Soc. Japan, 74, 239–241 (2005); arXiv:nlin/0409034v1 (2004).

    Article  ADS  Google Scholar 

  20. J. C. Brunelli, “The bi-Hamiltonian structure of the short pulse equation”, Phys. Lett. A, 353, 475–478 (2006); arXiv:nlin/0601014v1 (2006).

    Article  ADS  MathSciNet  Google Scholar 

  21. S. Liu, L. Wang, W. Liu, D. Qiu, and J. He, “The determinant representation of an N-fold Darboux transformation for the short pulse equation”, J. Nonlinear Math. Phys., 24, 183–194 (2017).

    Article  MathSciNet  Google Scholar 

  22. V. A. Vakhnenko, “Solitons in a nonlinear model medium”, J. Phys. A: Math. Gen., 25, 4181–4187 (1992).

    Article  ADS  MathSciNet  Google Scholar 

  23. A. N. W. Hone and J. P. Wang, “Prolongation algebras and Hamiltonian operators for peakon equations”, Inverse Problems, 19, 129–145 (2003).

    Article  ADS  MathSciNet  Google Scholar 

  24. J. C. Brunelli and S. Sakovich, “Hamiltonian structures for the Ostrovsky-Vakhnenko equation”, Commun. Nonlinear Sci. Numer. Simul., 18, 56–62 (2013).

    Article  ADS  MathSciNet  Google Scholar 

  25. B.-F. Feng, K. Maruno, and Y. Ohta, “Integrable semi-discretizations of the reduced Ostrovsky equation”, J. Phys. A: Math. Theor., 48, 135203 (2015); arXiv:1502.03891v1 [nlin.SI] (2015).

    Article  ADS  MathSciNet  Google Scholar 

  26. V. O. Vakhnenko and E. J. Parkes, “The two loop soliton solution of the Vakhnenko equation”, Nonlinearity, 11, 1457–1464 (1998).

    Article  ADS  MathSciNet  Google Scholar 

  27. A. J. Morrison, E. J. Parkes, and V. O. Vakhnenko, “The N loop soliton solution of the Vakhnenko equation”, Nonlinearity, 12, 1427–1437 (1999).

    Article  ADS  MathSciNet  Google Scholar 

  28. V. O. Vakhnenko and E. J. Parkes, “The calculation of multi-soliton solutions of the Vakhnenko equation by the inverse scattering method”, Chaos, Solitons and Fractals, 13, 1819–1826 (2002).

    Article  ADS  MathSciNet  Google Scholar 

  29. Y. Matsuno, “Cusp and loop soliton solutions of short-wave models for the Camassa-Holm and Degasperis- Procesi equations”, Phys. Lett. A, 359, 451–457 (2006).

    Article  ADS  MathSciNet  Google Scholar 

  30. C. M. Bender and J. Feinberg, “Does the complex deformation for the Riemann equation exhibit shocks?’ J. Phys. A: Math. Theor., 41, 244004 (2008); arXiv:0709.2727v1 [hep-th] (2007).

    Article  ADS  MathSciNet  Google Scholar 

  31. Y. Li and J. E. Zhang, “The multiple-soliton solution of the Camassa-Holm equation”, Proc. Roy. Soc. London. Ser. A, 460, 2617–2627 (2004).

    Article  ADS  MathSciNet  Google Scholar 

  32. Y. Li, “Some water wave equations and integrability”, J. Nonlinear Math. Phys., 12, suppl. 1, 466–481 (2005).

    Article  ADS  MathSciNet  Google Scholar 

  33. B. Xia, R. Zhou, and Z. Qiao, “Darboux transformation and multi-soliton solutions of the Camassa-Holm equation and modified Camassa-Holm equation”, J. Math. Phys., 57, 103502 (2016); arXiv:1506.08639v2 [nlin.SI] (2015).

    Article  ADS  MathSciNet  Google Scholar 

  34. P. R. Gordoa and A. Pickering, “Nonisospectral scattering problems: A key to integrable hierarchies”, J. Math. Phys., 40, 5749–5786 (1999).

    Article  ADS  MathSciNet  Google Scholar 

  35. D. Levi and O. Ragnisco, “Non-isospectral deformations and Darboux transformations for the third-order spectral problem”, Inverse Problems, 4, 815–828 (1988).

    Article  ADS  MathSciNet  Google Scholar 

  36. S. B. Leble and N. V. Ustinov, “Third order spectral problems: Reductions and Darboux transformations”, Inverse Problems, 10, 617–633 (1994).

    Article  ADS  MathSciNet  Google Scholar 

  37. I. Loris, “On reduced CKP equations”, Inverse Problems, 15, 1099–1109 (1999).

    Article  ADS  MathSciNet  Google Scholar 

Download references

Acknowledgments. The authors are grateful to the referee for the helpful comments. They also thank Professor Q. P. Liu for the useful suggestions and discussions.

Author information

Authors and Affiliations

  1. School of Mathematical Sciences, Huaqiao University, Quanzhou, Fujian, China

    Nianhua Li

  2. Department of Mathematics, National Research University “Higher School of Economics,”, Moscow, Russia

    Nianhua Li

  3. Department of Mathematics, School of Science, China University of Mining and Technology, Beijing, China

    Gaihua Wang

  4. School of Science, Zhongyuan University of Technology, Zhengzhou, China

    Yonghui Kuang

Authors
  1. Nianhua Li
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Gaihua Wang
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Yonghui Kuang
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Nianhua Li.

Ethics declarations

Conflicts of interest. The authors declare no conflicts of interest.

Additional information

This research is supported in part by the National Natural Science Foundation of China (Grant Nos. 11505064, 11805071, and 11871471) and the Natural Science Foundation of Fujian Province, China (Grant No. 2016J05008).

Prepared from an English manuscript submitted by the authors; for the Russian version, see Teoreticheskaya i Matematicheskaya Fizika, Vol. 203, No. 2, pp. 205–219, May, 2020.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Li, N., Wang, G. & Kuang, Y. Multisoliton solutions of the Degasperis–Procesi equation and its shortwave limit: Darboux transformation approach. Theor Math Phys 203, 608–620 (2020). https://doi.org/10.1134/S0040577920050049

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1134/S0040577920050049

Keywords

Search

Navigation