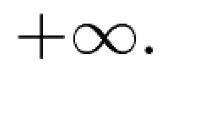Abstract
Let G be a connected and finite graph with the set of vertices V and the set of edges E. Let \(M_{G}\) be the Hardy–Littlewood maximal function defined on graph G and \(M_{\upalpha ,G}\) \((0\le \upalpha <1)\) be its fractional version. In this paper, the regularity problems related to \(M_{G}\) and \(M_{\upalpha ,G}\) will be studied. We show that \(M_{G}:\mathrm{BV}_p (G)\rightarrow \mathrm{BV}_p(G)\) is bounded and \(M_{\upalpha ,G}: \ell ^p(V)\rightarrow \mathrm{BV}_q(G)\) is bounded and continuous for all \(0<p,\,q\le \infty \). Here \(\mathrm{BV}_p(G)\) is the set of all functions of bounded p-variation on V. The operator norms of \(M_{G}\) and \(M_{\upalpha ,G}\) have also been investigated.
Similar content being viewed by others
References
Aldaz, J.M., Pérez Lázaro, J.: Functions of bounded variation, the derivative of the one dimensional maximal function, and applications to inequalities. Trans. Am. Math. Soc. 359(5), 2443–2461 (2007)
Beltran, D., Madrid, J.: Endpoint Sobolev continuity of the fractional maximal function in higher dimensions. Math. Res. Not. (to appear). arXiv:1906.00496 (2019)
Beltran, D., Madrid, J.: Regularity of the centered fractional maximal function on radial functions. arXiv:1911.00065.pdf (2019)
Carneiro, E., Mardid, J.: Derivative bounds for fractional maximal functions. Trans. Am. Math. Soc. 369(6), 4063–4092 (2017)
Carneiro, E., Mardid, J., Pierce, L.B.: Endpoint Sobolev and BV continuity for maximal operators. J. Funct. Anal. 273(10), 3262–3294 (2017)
Carneiro, E., Moreira, D.: On the regularity of maximal operators. Proc. Am. Math. Soc. 136(12), 4395–4404 (2008)
Cowling, M., Meda, S., Setti, A.G.: Estimates for functions of the Laplace operator on homogeneous trees. Trans. Am. Math. Soc. 352(9), 4271–4293 (2000)
Dungey, N.: A Littlewood–Paley–Stein estimate on graphs and groups. Stud. Math. 189(2), 113–129 (2008)
González-Riquelme, C.: Sobolev regularity of polar fractional maximal functions. arXiv:1910.05590 (2019)
Hajłasz, P., Malý, J.: On approximate differentiability of the maximal function. Proc. Am. Math. Soc. 138(1), 165–174 (2010)
Hajłasz, P., Onninen, J.: On boundedness of maximal functions in Sobolev spaces. Ann. Acad. Sci. Fenn. Math. 29, 167–176 (2004)
Kinnunen, J.: The Hardy–Littlewood maximal function of a Sobolev function. Isr. J. Math. 100, 117–124 (1997)
Kinnunen, J., Lindqvist, P.: The derivative of the maximal function. J. Reine Angew. Math. 503, 161–167 (1998)
Kinnunen, J., Saksman, E.: Regularity of the fractional maximal function. Bull. Lond. Math. Soc. 35(4), 529–535 (2003)
Kurka, O.: On the variation of the Hardy–Littlewood maximal function. Ann. Acad. Sci. Fenn. Math. 40, 109–133 (2015)
Korányi, A., Picardello, M.A.: Boundary behaviour of eigenfunctions of the Laplace operator on trees. Ann. Scuola Norm. Sup. Pisa Cl. Sci. (4) 13(3), 389–399 (1986)
Liu, F.: Continuity and approximate differentiability of multisublinear fractional maximal functions. Math. Inequal. Appl. 21(1), 25–40 (2018)
Liu, F., Chen, T., Wu, H.: A note on the endpoint regularity of the Hardy–Littlewood maximal functions. Bull. Austral. Math. Soc. 94(1), 121–130 (2016)
Liu, F., Mao, S.: On the regularity of the one-sided Hardy–Littlewood maximal functions. Czech. Math. J. 67(142), 219–234 (2017)
Liu, F., Wu, H.: On the regularity of the multisublinear maximal functions. Can. Math. Bull. 58(4), 808–817 (2015)
Liu, F., Wu, H.: Endpoint regularity of multisublinear fractional maximal functions. Can. Math. Bull. 60(3), 586–603 (2017)
Liu, F., Wu, H.: Regularity of discrete multisublinear fractional maximal functions. Sci. China Math. 60(8), 1461–1476 (2017)
Liu, F., Wu, H.: On the regularity of maximal operators supported by submanifolds. J. Math. Anal. Appl. 453, 144–158 (2017)
Luiro, H.: Continuity of the maixmal operator in Sobolev spaces. Proc. Am. Math. Soc. 135(1), 243–251 (2007)
Luiro, H.: On the regularity of the Hardy–Littlewood maximal operator on subdomains of \({\mathbb{R}}^d\). Proc. Edinb. Math. Soc. 53(1), 211–237 (2010)
Luiro, H.: The variation of the maximal function of a radial function. Ark. Mat. 56(1), 147–161 (2018)
Luiro, H., Madrid, J.: The variation of the fractional maximal function of a radial function. Int. Math. Res. Not. (to appear). arXiv:1710.07233
Madrid, J.: Endpoint Sobolev and BV continuity for maximal operators, II. Rev. Mater. Iberoam. (to appear). arXiv:1710.03546 (2017)
Russ, E.: Riesz transforms on graphs for \(1\le p\le 2\). Math. Scand. 87(1), 133–160 (2000)
Soria, J., Tradacete, P.: Best constants for the Hardy–Littlewood maximal operator on finite graphs. J. Math. Anal. Appl. 436(2), 661–682 (2016)
Tanaka, H.: A remark on the derivative of the one-dimensional Hardy–Littlewood maximal function. Bull. Aust. Math. Soc. 65(2), 253–258 (2002)
Acknowledgements
The authors would like to express their sincerely thanks to the referee for his or her valuable remarks and suggestions, which made this paper more readable. The authors also want to thank Professor Javier Soria for helpful comments during the preparation of this manuscript. The first author was supported partly by NSFC (Grant No. 11701333) and SP-OYSTTT-CMSS (Grant No. Sxy2016K01). The second author was supported partly by NSFC (Grant Nos. 11471041, 11671039, 11871101) and NSFC-DFG (Grant No. 11761131002).
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
About this article
Cite this article
Liu, F., Xue, Q. On the variation of the Hardy–Littlewood maximal functions on finite graphs. Collect. Math. 72, 333–349 (2021). https://doi.org/10.1007/s13348-020-00290-6
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s13348-020-00290-6