Introduction

Spheroidal graphite cast irons (SGCIs) are a family of materials that compete favorably with steels for application in the manufacture of structural engineering components. The material family is featured by a microstructure that can be described as constituted by a “high-silicon steel matrix surrounding spheroidal carbon sinks.” Carbon sinks and silicon allow for solid-state heat treatment transformations that are not possible with steel. For example, the entire wall thickness can be transformed into carbon saturated austenite. During the liquid–solid transformation, carbon and silicon allow a much lower (compared with steel) melting temperature, self-feeding properties, a carbide-free matrix and protection against surface oxidation. On the other hand, graphite spheroids influence the plastic deformation of SGCIs under load after the onset of necking and/or even prevent the onset of necking to be met.

Because of its material characteristics, SGCIs are often evaluated as an alternative to cast, forged and welded steels. As a consequence, linear elastic strength parameters (yield strength, hardness, fatigue strength) are likely to be directly compared between SGCIs and steels. Ductility and toughness or the influence of material plastic and fracture properties on components design have not been sufficiently considered in existing material standard. This paper analyses the material properties information required by the design procedures for the assessment of components under static loads, to establish the main metrics for a proper reclassification of SGCIs Grades, in view of design needs.

Plastic properties after yielding are also important for the designer, and they are often empirically taken in consideration as an “a priori” material selection.

Reclassification Framework

The map in Fig. 1 shows different available processes, using yield strength-YS and strength ratio SR (UTS/YS) as coordinates. The map refers to a given general foundry process with particular focus on the manganese content. It is assumed that Mn is ≤ to 0.35%. Furthermore, separately cast test bars Y25 mm with a relevant wall thickness t ≤ 30 mm were used in the construction of this map.

Figure 1
figure 1

Designation of SGCIs available processes

Full size image

Design procedures take into consideration the SR and not the UTS Rm directly because the strength ratio (SR) = Rm/Rp0.2 is an immediate rough but reliable indicator of the material strain hardening behavior.

In Fig. 1, the ordinate SR is intentionally not scaled, because values depend on the base chemistry, foundry process, casting wall thickness, etc. The graph only provides a comparative image of the different processes.

Comparison with traditional specifications based on minimum properties Rp0.2 and Rm taken separately can be done by drawing a vertical line starting from the point (Rp0.2 min; Rm min/Rp0.2 min) and then connecting this point with (Rm min; 1). All points on the right conform to the minimum properties taken separately. By dividing the abscissa Rp0.2 into arbitrary intervals, we can classify materials into “Grades” (different Rp0.2 ranges with the lower value designating the grade) within a “Process.”

A material specification may comprise several adjacent grades belonging to the same process, depending on the acceptable variation of allowable hardness, machinability, and mechanical properties that can be obtained on the castings.

The terms “perlitizers/pearlite promoters” refer to elements (generally Cu and Sn) able to promote the formation of as-cast pearlite. The term “Alloying” refers to elements (generally Cu, Ni, Mo) able to displace the TTT and/or CCT heat treatment curves to the right side, promoting quenchability by avoiding the crossing of the pearlite nose.

Material Quality Index

Tensile tests conform to the present reclassification if the calculated “material quality index”15

$$ {\text{MQI}} = R_{m}^{2} A_{5} /10000 $$
(1)

with Rm in [MPa] and A5 in [1/100], is not lower than a minimum value that should be indicated for each grade.

In Fig. 2, values corresponding to Rm,min and A5,min of commercially significant grades are reported, including interpolations with indication of the reference source.Footnote 111,12,16,17,21 The actual MQI should always be higher, if the minimum specified properties are to be achieved, accounting for an allowable hardness (strength) scatter.

Figure 2
figure 2

MQI of SGCIs available processes

Full size image

Description of Material Manufacturing Processes

Structures Obtained in the As-Cast State and/or Heat Treated by Annealing

Process F Fully Ferritic Ductile Iron, with no Addition of Pearlite Promoters or Alloying Elements, Strength Increases with Si Content

Grades 220F, 240F, 260F, 300F in Fig. 1 align well with the traditional ISO 1083: 201816 grades (Rp0.2 min − Rm min) 220–350, 240–400, 250–400, 310–450 with increasing the silicon content from slightly below 2% up to slightly more than 3% which is a range generally considered as “normal” foundry practice.

Grades 340F, 380F, 420F, 480F align with ISO 1083: 2018 Silicon Solid Solution Strengthened Ferritic (SSSSF) grades 350–450, 400–500, 470–600.

Silicon contents equal to 3.2%, 3.8%, 4.2%, respectively, are suggested by ISO 1083: 2018 for SSSSF grades. The SSSSF concept should not be confused with high-silicon ductile irons for use at high temperatures.

Process F grades may require annealing, either in correspondence of a too low silicon content to dissolve carbides or in the correspondence of a too high manganese content to dissolve pearlite.

The first commercial practice of a SSSSF grade originated with the intent to fully recycle low-yield ductile iron casting returns: the addition of FeSiMg as the spherodizer would imply a silicon content greater than the typical “normal.” At this new (higher) silicon content, the yield strength Rp0.2 was found to be acceptable, compared with the requirements of the traditional ferritic–pearlitic grades 320–500 and 370–600 [MPa], even if the ultimate tensile strength Rm was insufficient to meet the material standard requirements.

In some commercial current practices, ferritic–pearlitic ductile irons are characterized by a wide variation in hardness within the casting and between castings, a fact that negatively affects machinability. The possibility to replace these irons with fully ferritic materials, characterized by a high level of uniformity in hardness and then in machining, appeared attractive for high volume automotive components and greatly contributed to the commercial success of this SSSSF approach. Consequently, the idea was further developed and extended to higher silicon contents in international material standards.

In addition to the hardness uniformity, a high post-necking elongation at fracture A5 is an attractive property of SSSSF grades. However, the decrease in the strength ratio and the negative effect of silicon content on the low-temperature behavior must also be considered. The risk of chunky graphite and its negative effect on fatigue strength and fracture toughness should also be of concern.6

Non-alloyed ferritic grades offer the best opportunity for uniform properties within the casting, allowing for design with the maximum geometric freedom. Silicon content (below 2% up to 4.2%) and heat treatment are the drivers for obtaining the different grades.

Low silicon contents are used for optimizing the ductility at low temperatures (up to − 40 °C), in the as-cast or annealed state. Normal silicon contents are of common use in the industrial practice in the as-cast state. High silicon contents (between 3.2 and 4.2%), in the as-cast state, are viewed as an opportunity to replace ferritic–pearlitic grades, avoiding potential hardness inhomogeneity.

Low and normal silicon spheroidal graphite cast iron grades are the most suited for obtaining sound castings. High silicon grades are more prone to formation of porosity and/or chunky graphite.

Process FP Pearlite Promoters Added to Modify the F–P Content

Pearlite promoters are added to ferritic grades 220F, 240F, 260F, 300F, to promote pearlite in the as-cast state.

Grades 300FP, 340FP, 380FP, 420FP match traditional ISO 1083: 2018 grades 320–500, 350–550, 370–600, 420–700 [MPa].

FP grades are of interest in design because they allow for increasing strength, therefore maintaining a high strength ratio. These grades are sensitive to the casting wall thickness, i.e., to the cooling rate in the mold which affects hardness, ductility and machinability. This is the reason why conventional standards require low values for the minimum elongation at fracture A5 (consider ISO1083/JS/600-3, 700-2, 800-2).

In FP grades, the addition of pearlite promoters is necessary. Pearlite and ferrite form in the solid state when carbon saturated austenite transforms during slow cooling. The transformation time is measured in hours and increases with wall thickness. In addition, the distance between spheroids (formed during solidification) increases with wall thickness. The resultant effect is segregation of pearlite promoting elements. Segregation increases with decreasing the cooling rate (or increasing the wall thickness), resulting in a reduction in ductility as well as non-uniform hardness in different section sizes of the casting.

Since the 1970s, the Fiat 52215 material standard12 has deviated from the approach of international material standards, by promoting ferritic–pearlitic spheroidal graphite cast irons based on hardness control and uniform wall thickness (consider Gh 60.38.10, Rm min 590 MPa Rp0.2 min 370 MPa A5 min 10%). As an evolution of the Fiat approach, more recent commercial evidence14 indicates the possibility that pearlite promoters are also added to the lower grades of SSSSF irons. Together with SSSSF grades, the hardness-controlled FP grades offer a solution to design needs by increasing strength while also maintaining good ductility. SSSSF and FP grades differ between each other because of the strength ratio and consequent plastic properties.

Structures Obtained After Quenching Into a Salt Bath

Process IDI7—Proeutectoid Ferrite and Pearlite from Intercritical Austenitizing Non-alloyed Iron Followed by Quenching Into a Salt Bath

If a non-alloyed ferritic grade is austenitized in the intercritical range AC1–AC3, a fraction of the matrix transforms from ferrite to austenite, while the remaining matrix remains as proeutectoid ferrite. Subsequent quenching into a salt bath transforms the austenite into pearlite. In Fig. 3, the microstructure evolution with different cooling rates is shown.

Figure 3
figure 3

Possible microstructure evolution with different cooling rates

Full size image

Although the heat treatment process of IDI appears similar to austempering, relevant differences distinguish the so-called Perferritic Isothermed Ductile Iron (IDI) from the Intercritical Ausferritic Austempered Ductile Iron (IADI).

The salt bath in the IDI process only plays a role to accelerate the cooling rate to execute an austenite-to-pearlite transformation above the martensite start temperature.

Hence, the properties of IDI are comparable to those of FP grades and not to those of IADI.

The advantage of IDI, when compared to FP is in the perferritic, instead of pearlitic–ferritic structure, and the perferritic microstructure is imposed by the higher cooling rate. The low carbon mobility at high cooling rates results in an interconnected structure which offers higher strength with a significant amount of proeutectoid ferrite. This, in turn, results in significantly higher ductility, Charpy impact energy and expected toughness. The absence of pearlite promoters results in pronounced uniformity of the matrix in different wall thicknesses.

In IDI, Si content can vary in the same range as in the Si-based ferritic grades. The lowest Si grades exhibit the best Charpy impact energy results, even at low temperatures (however not so good as upper ausferritic ADIs). Low and “normal” silicon contents can avoid the chunky graphite formation. By increasing the silicon content, strength increases and ductility decreases.

Process ADI-Ausferritic Spheroidal Graphite Irons (Austempered Ductile Iron—ADI)

ADI requires alloying to avoid the formation of pearlite during the heat treatment. The formation of pearlite in the as-cast state is a resultant consequence of this. The alloying policy in the ADI process has some degree of freedom for its optimization. In fact, alloying elements effective in the ADI process are:

  • Carbon (increasing the austenitizing temperature)

  • Manganese (segregates at the grain boundary)

  • Copper (segregates to the spheroid/matrix interface)

  • Nickel (segregates to the spheroid/matrix interface, but not as severely as Cu)

  • Molybdenum (segregates to the grain boundary)

The cast wall thickness (and uniformity) along with the design requirements will dictate the alloy content for the component which, in turn, drives the selection of the correct heat treatment temperatures and times. The heat treatment equipment (quench severity and capacity) also plays an important role in the production of a successful ADI casting

Designers will consider two advantages of ADI, in addition to its high strength level:

  • The brittle–ductile transition temperature of ADI is low because of the ausferritic structure;

  • The partial transformation of austenite into martensite (PITRAM, SITRAM effect) allows, in many cases, to avoid surface hardening treatments such as nitriding, induction or case hardening.

On the other hand, the ausferritic matrix limits the use of ADI at high temperatures.

The “Germanite” Mühlberger invention19 in the 1970s established two fundamental statements for the development of ADI technology:

  1. 1.

    Where the Mn content is not greater than 0.3%, and the casting wall thickness is not larger than 100 mm, Mo is not a carbide stabilizer

  2. 2.

    It makes sense to distinguish the ADI process into two separate items:

    1. a.

      Austenitization above the critical range (GGG 100 B/A)

      1. i.

        Austempering above the ausferritic nose (Upper ADI, shortly UADI)

      2. ii.

        Austempering below the ausferritic nose (Lower ADI, shortly LADI)

    2. b.

      Austenitization inside the critical range (GGG 70 B/A) (Intercritical ADI, IADI)

The designation of the two items comes from the German use (GGG for spheroidal cast iron) and from the ancient metric kgf/mm2, meaning B/A “Bainitic/Austenitic” as it was common to interpret the austempered structures in those times.

The process GGG 100 B/A is implemented through a Cu–Ni–Mo alloying content driven by the largest casting wall thickness and through a heat treatment cycle driven by the grade to be obtained.

The statement (1), permitting Mo as alloying element, reduces the necessity to use a high austenitizing temperature to increase hardenability. In this way, a shorter austempering time is allowed for which promotes austenite stability and, consequently, improves machinability.

The ADI process displayed in Fig. 1 (grades from 540 to 1140 ADI) includes the original GGG 100B/A M-Type Germanite process in addition to other chemistries such as molybdenum free and manganese content greater than 0.30%.

The process GGG 70 B/A, implemented through a Cu–Ni–Mo low alloying content (also using a low intercritical austenitizing temperature), is suitable for limited casting wall thicknesses.

Grade 480 M-Typ in Fig. 1 matches the original GGG 70 B/A Mühlberger patent.

The grade GGG 70 B/A attracted the interest of the American automotive industry, promoting the definition of the material grade AD 750 into the material standard SAE J2477: 2004, which advises that “…this grade will exhibit a microstructure containing some pro-eutectoidic ferrite…”.

The US Patent9 “Machinable austempered cast iron article having improved machinability, fatigue performance and resistance to environmental cracking,” essentially recalls the “Germanite/Mühlberger GGG 70 B/A” invention,19 claiming a preferred chemistry which substantially avoids or limits Mo alloying. Consequently, this material, commercially known as MADI (Machinable ADI), can be described as similar to the “Germanite/Mühlberger GGG 70 B/A” where the Mo content is claimed as lower than 0.20%. The chemistry limitation reduces the casting wall thickness able to be austempered with this process, compared with GGG 70 B/A. Data reported in10 based on chemistry Si 2.64% Mn 0.25% Cu 0.71% Ni 0.96% Mo = 0% are represented by the grade 480 MADI.

The inventors of MADI reported data from tests on resistance to environmental cracking:9 proeutectoid ferrite increases the resistance to environmental cracking in intercritically austenitized materials.

They also reported data on machining forces10 with the intercritically austenitized ADI being the most machinable of all grades of ADI.

The main advantage expected by selecting the ADI grades 480 M-Type and/or 480 MADI is the improved machinability, a direct consequence of the lower hardness. At the same time, better fatigue properties, compared with pearlitic ductile irons, along with impact resistance similar to the ferritic grades, are expected.

Manganese increases the carbon solubility into the primary austenite (opposite effect as in steels), while it decreases its solubility into the metastable austenite. Successful production of low grade ADIs is facilitated by low manganese content with carefully controlled austenitizing temperature, controlled chemical composition—austenitizing and austempering times—salt bath quenching capacity.

Among design properties, it is worth consideration that ADIs are favorite materials when high strain rates and/or low temperatures are of interest.20

Materials Properties of Interest in Structural Design

Mechanical structural components are often designed considering static and cyclic loadings, being the maximum equivalent stress not larger than the material YS Rp0.2.

However, this design process begins after the material grade has been selected.

If the material selection would be based only on YS, very few steel casting would be used for mechanical structural components. Steel forgings and welded fabrications would be considered in alternative to SGCI castings only considering economic issues and expected defects.

If a SGCI casting solution was adopted, for a given YS only one grade (see Fig. 1) would be candidate for Rp0.2 ≤ 300 MPa and/or Rp0.2 > 600 MPa. Among 300 and 600 MPa, more than one option exists. On the base of information available from material standards, it could be expected that the material selection would be based also upon elongation at fracture A5, Brinell hardness range, machinability, supply chain configuration, casting cost.

SSSSF grades from 300F to 480F would appear the most attractive to the designer in this range, also considering that Charpy test is sometimes claimed to be no more representative for design needs.

If design is based only on YS, why the EF A5 is important?

The MQI concept (see Fig. 2) helps the designer to control A5, while Fig. 1 advises that also the SR Rm/Rp0.2 is important.

And also the plane strain static fracture toughness KIC is important.

However, material standards are based on minimum values of UTS Rm, YS Rp0.2, EF A5, with no requirement for the SR Rm/Rp0.2 and for KIC.

YS, SR, EF, KIC are the design parameters necessary for controlling, with a simple linear elastic FEM calculation, the maximum overload a component is able to sustain without undergoing to failure initiation.

If the designer is confident on these properties, she/he will be able to design more extensively SGCI castings instead of steel components and, inside the SGCIs family, will be able to select more efficiently the most appropriate grade.

Discussion on Analytical Design Rules Against Overloading

It is not necessary to read this chapter and it is possible to go immediately to the chapter “Conclusions.”

This chapter is dedicated to those readers that are willing to understand and/or to give further contributes on how the above-mentioned material properties are connected with design rules in case of overloading assessment.

Analytical Strength Assessment of Components Technically Without Fault

In Fig. 4, all the possible states of tensile stress are represented with reference to the principal triad, being

Figure 4
figure 4

Possible states of stress and strain in tension

Full size image
$$ \sigma_{1} \ge \sigma_{2} \ge \sigma_{3} \ge 0 $$
(2)

The red line on the left is drawn assuming the coincidence between the middle and the minimum principal tension, while the black line on the right is drawn assuming coincidence between the middle and the maximum tension.

For a constant maximum principal, the minimum principal stress varies from

$$ \sigma_{3} = 0\;({\text{plane}}\,{\text{stress}}) $$
(3)

to

$$ \sigma_{3} = \nu(\sigma_{1} + \sigma_{2} )\;({\text{plane strain}}\;{\varepsilon_{3}} = 0)\;{\text{or}}\;{\text{CF}}_{3} = \sigma_{3} /[\nu (\sigma_{1} + \sigma_{2} )] = 1 $$
(4)

where CF3 is called “Constraints Factor of the Minimum Principal Stress,” being ν = 0.27 the Poisson ratio.

The Triaxiality Factor TF is calculated by dividing the mean hydrostatic stress by the von Mises equivalent stress.

On the bottom line of the diagram, we find the whole range of plane stress conditions (CF3 = 0, TF = 0.33 ÷ 0.67), from the uniaxial up to the symmetric biaxial state of stress. On the bottom line, the Von Mises equivalent stress is given by:

$$ \frac{{\sigma_{\text{eq}} }}{{\sigma_{1} }} = \sqrt {1 + \left( {\frac{{\sigma_{2} }}{{\sigma_{1} }}} \right)^{2} - \left( {\frac{{\sigma_{2} }}{{\sigma_{1} }}} \right)} = 1 \div 0.866 \div 1 $$
(5)

On the upper line of the diagram, we find the whole range of plane strain conditions (CF3 = 1, TF = 0.92 ÷ 1.84), where

$$ \frac{{\sigma_{\text{eq}} }}{{\sigma_{1} }} \approx 1.18 + 0.22 {\text{TF}}^{2} - 0.80 {\text{TF}} = 0.63 \div 0.44 \div 0.46 $$
(6)

On the left side (red line), it is defined as:

$$ \frac{{\sigma_{\text{eq}} }}{{\sigma_{1} }} \approx 1 - 0.37 {\text{CF}}_{3} = 1 \div 0.63 $$
(7)

On the right side (black line), it is defined as:

$$ \frac{{\sigma_{\text{eq}} }}{{\sigma_{1} }} = 1 - 0.54\,{\text{CF}}_{3} = 1 \div 0.46 $$
(8)

As a rule of thumb, in plane stress σeq ~ σ1, while in plane strain σeq ~ ½ σ1. This helps in understanding how plane strain conditions contribute to stay far from yielding, promoting a linear elastic behavior.

In Fig. 4, the uniaxial test probe is associated with the state of stress TF = 0.33 and CF3 = 0, while three different blunt notched test probes having intermediate constraints factor are used for the measurement of the “strain at failure versus triaxiality factor.”

FKM Criterion for the Critical Strain

In FKM,13 we find the following criterion for the critical strain εertr:

$$ \varepsilon_{\text{ertr}} = \varepsilon_{\text{ref}} \quad {\text{for}}\,{\text{TF}} \le 1/3\;{\text{or}}\; \varepsilon_{\text{ref}} \le \varepsilon_{\text{th}} $$
(9)
$$ \varepsilon_{\text{ertr}} = \varepsilon_{\text{th}} + 0.3\left( {\frac{{\varepsilon_{\text{ref}} - \varepsilon_{\text{th}} }}{0.3}} \right)^{{3{\text{TF}}}} \quad {\text{for}}\;{\text{TF}} > 1/3\;{\text{and}}\;\varepsilon_{\text{ref}} > \varepsilon_{\text{th}} $$
(10)

where

$$ \varepsilon_{\text{ref}} = A_{5} \,{\text{for steels and}}\,0.4\,A_{5} \,{\text{for ductile irons}} $$
(11)
$$ \varepsilon_{\text{th}} = 5\% \,{\text{for steels and}}\,4\% \,{\text{for ductile irons when }}A_{5} \ge 6\% $$
(12)
$$ \varepsilon_{\text{th}} = R_{p0.2} /E\;{\text{when}}\,A_{5} < 6\% $$
(13)

Steels are defined as ductile materials, while SGCIs are defined as semi-ductile when A5 ≥ 6% and non-ductile when A5 < 6%. The attribute “ductile” for steel and “semi-ductile” for SGCIs depend on the post-necking behavior in the quasi-static uniaxial tensile test.

Common steels undergo pronounced restriction in area after the onset of necking, while ductile irons breaks before, or at, or after the onset of necking but, in any case, with a reduction in area not comparable with that characteristic of steels. This happens because of the spheroids.

How the different post-necking behavior contributes to different design properties is a complex issue that will not be considered in this discussion. Here, we simply observe how, for SGCIs, is possible to adopt two different approaches:

  1. 1.

    Based on εref = 0.4 A5, FKM guidelines, conservative, see Fig. 5;

    Figure 5
    figure 5

    Critical value of total strain for semi-ductile and brittle materials (FKM)

    Full size image
  2. 2.

    Based on εref = A5, non conservative, see Fig. 6.

    Figure 6
    figure 6

    Critical value of total strain for ductile materials (steels) (FKM)

    Full size image

In any case, the beneficial effect of a high elongation at fracture A5 is amplified at low TFs (plane stress), as it happens on the smooth or blunt notched casting surface in the absence of flaws and of locally applied loads.

In20 an experimental determination of the strain at failure versus triaxiality factor is considered for an ADI cast iron.

In this case, the plastic true strain component pf is calculated, following the expression

$$ p_{f } = \varepsilon_{\text{th}} \left( {\frac{{\varepsilon_{f} }}{{\varepsilon_{\text{th}} }}} \right)^{{\frac{1}{{R_{v} }}}} $$
(14)

where

$$ R_{v} = \frac{2}{3}\left( {1 + \nu } \right) + 3\left( {1 - 2\nu } \right){\text{TF}}^{2} $$
(15)

and

$$ \varepsilon_{\text{th}} = \, 4\% $$
(16)

(ν Poisson’s ratio, TF the triaxiality factor).

The true plastic strain in uniaxial tensile test was found to be εf = 14.45%, corresponding to the engineering value A5 = 16.34%.

The result is compliant with FKM criterion if approach (2) is adopted.

The FKM Double Criterion for the Critical Equivalent Stress

The critical equivalent stress at the reference point, otherwise stated as Component Static Strength, using local stress approach of FKM guidelines,13 in tension is given by

$$ \sigma_{\text{SK}} = R_{p0.2} n_{pl} $$
(17)

where npl is the double criterion section factor

$$ n_{pl} = { \hbox{min} }\left( {\sqrt {\frac{{E\varepsilon_{\text{ertr}} }}{{R_{p0.2} }}} ;K_{p} } \right) $$
(18)

The first term is the square root of the ratio between the total critical strain εertr and the elastic strain at yield Rp0.2/E.

The first criterion for σSK in tension

$$ \sigma_{\text{SK}} = \sqrt {{\text{ER}}_{p0.2} \varepsilon_{\text{ertr}} } \quad \left( {M_{1} , \, M_{2} , \, M_{3} \,{\text{when}}\,{\text{TF}} = 0.33,0.67,1.80} \right) $$
(19)

represents the maximum Neuber elastic peak applied to an ideally elastic perfectly plastic material model. Such a parameters can be assumed as “Ashby Material Index,”1 when evaluated at TF = 0.33, 0.67, 1.80, respectively.

The second term in (18) is the plastic notch factor, given by a material independent geometric coefficient, calculated using an elastic perfectly plastic material model (e.g., 1.5 for a rectangular section in bending) multiplied by

$$ 0.5 \, \left( {1 + R_{m} /R_{p0.2} } \right)\,{\text{only if}}\,R_{m} /R_{p0.2} \ge 1.33\,\left( {\text{Strain Hardening Contribution to the Effective Yield}} \right) $$
(20)

Multiplying by Rp0.2, we get the offset yield strength contributing to the net section yielding assessment

$$ R_{{p,{\text{ers}}}} = 0.5\left( {R_{p0.2} + R_{m} } \right)\,{\text{if}}\,R_{m} /R_{p0.2} \ge 1.33 ; R_{{p,{\text{ers}}}} = R_{p0.2} \,{\text{if}}\,R_{m} /R_{p0.2} < 1.33 \left( {M_{4} } \right) $$
(21)

Rp,ers is also assumed as “Ashby Material Index.”

The proposed reclassification can then support the FKM static assessment using local stress approach with the following material properties, associated with each grade:

$$ \begin{aligned} & {\text{Yield Strength}}\,R_{p0.2} \quad \left( {P_{1} } \right) \\ & {\text{Strength Ratio}}\,R_{m} /R_{p0.2} \quad \left( {P_{2} } \right) \\ \end{aligned} $$

and the following material indices:

  • M1, M2, M3 critical strain maximum allowable Neuber elastic peak in tension, applied to an ideally elastic perfectly plastic material model at TF = 0.33, 0.67, 1.80;

  • M4 offset yield strength in tension, contributing to the net section yielding assessment by strain hardening.

Note that also A5 is a material property and that also MQI is a material index; however, they have no direct use in design but are used inside calculation steps for M1, M2, M3, which can be directly used in design.

For this, A5 and MQI are intentionally not classified as “Ashby Material Property” and “Ashby Material Index.”

The provided design material properties and indices enable to predict the initiation of a failure, but they will not provide any information on its possible evolution, which is the field of the assessment under cyclic loads and/or fracture mechanics and/or elastic–plastic analysis and/or other assessment criteria.

Design Needs Coming from BS 7910:20058 and Equivalent SINTAP and R6 Procedures: Acceptability of Flaws

We consider the static fracture mechanics approach applied to a cracked component characterized by a plane strain state of stress beyond the crack tip. The intent is to measure the Plane Strain Linear Elastic Fracture Toughness KIC material property, independent from the test probe, then directly applicable in design.

$$ {\text{Plane Strain Linear Elastic Fracture Toughness}}\quad K_{\text{IC}} \quad \quad \left( {P3} \right) $$

Some SGCIs grades (e.g., pearlitic, high-strength ADI) permit the direct measurement of KIC, compliant to ASTM E399, using a reasonable size CT test probe (e.g., taken from a Y50 [mm] test sample). Other grades (e.g., low-silicon ferritic, low-strength ADI) do not. Grades containing a significant amount of pro-eutectoidic ferrite at low/normal silicon contents require a J testing approach.

EN 1563:2018 Annex H11 advises: “With regard to the initiation of instable crack propagation under linear elastic conditions, fracture toughness values KIC in accordance with” ISO 12135:2016 and/or ASTM E399:2012 “are considered as transferable to the component.”

“The only characteristic material values to be considered as transferable to the component with regard to the initiation of crack propagation under elastic–plastic conditions are physical crack initiation toughness values (e.g., Ji in accordance with ISO 12135) excluding any crack growth. The characteristic crack initiation toughness value JIc according to ASTM E 1820 contains considerable amounts of ductile tearing and does not fulfill the above condition unless additional validity requirements are met.”

Baer5 also advises: “As has been shown for Ductile Cast Irons, the value of elastic–plastic fracture mechanics crack initiation toughness may vary up to 60% even when the same set of data is analyzed simply using different definitions from different editions of several ASTM and ISO test standards.”

When a valid Ji (J integral at crack growth initiation) is available, it can be converted, under plane strain conditions, into

$$ K_{\text{I}}^{\text{J}} = \sqrt {\frac{{EJ_{i} }}{{1 - \upsilon^{2} }}} $$
(22)

to be used with the same meaning of KIC.

We suggest describing this possibility using the following sentences: “The actual test probe cannot behave under linear elastic conditions with the material under examination. If the test probe would change according to the linear elastic requirements, then a valid KIC ≈ KJI would be measured.”

EN 1563:2018 Annex H11 advises: “As far as performing fracture mechanical assessments on spheroidal graphite cast iron components is concerned, compilations of engineering rules and regulations such as the British Standard BS 7910 or the European FITNET procedure are available.” The aforementioned regulations are based on the Failure Assessment Diagram (FAD).

Consider a sharply notched generic component, loaded with a generic load pattern P, with the material behaving in a perfectly linear elastic way. Assume that the component is also cracked at the notch tip and that the crack opens in Mode I. The stress intensity factor (SIF) KI is proportional to the load P and tables or linear elastic FEM analysis gives the constant of proportionality. It is of common use to write the proportional relationship in the form

$$ K_{\text{I}} = \sigma_{\text{ref}} \sqrt {\pi \alpha^{2} a} $$
(23)

where KI (P) is the calculated SIF and σref (P) is an arbitrary chosen reference stress, also proportional to P.

The “equivalent crack length” is simply calculated from

$$ \alpha^{2} a = \frac{1}{\pi }\left( {\frac{{K_{\text{I}} }}{{\sigma_{\text{ref}} }}} \right)^{2} $$
(24)

and it is a constant characteristic of the component geometry, loading pattern, reference stress definition, but independent from the load P intensity.

From:22

“Whereas there is only a single KI solution, the limit load,” then σref, “depends on whether the specimen is in plane stress or plane strain” then, generally speaking, on the state of stress, “and on whether the von Mises or Tresca yield criterion is adopted” then, generally speaking, on the yield criterion.

We assume here that σref is always defined with reference to plane strain state of stress and von Mises yielding criterion.

If σref is defined (using available formulas for standard geometries and/or, generally, by linear elastic FEM analysis) following a criterion of yielding concurrent with the crack growth initiation, then the formulas (23) and/or (24) can be seen as a prediction of the crack growth initiation based on the simple calculation of the linear elastic SIF. For a given linear elastic proportional loading pattern α2a, the point (σref; KI) corresponds to the crack growth initiation on the failure assessment line.

For those loading patterns to which LEFM applies, the Failure Assessment Line (initiation of crack propagation) becomes

$$ K_{\text{IC}} = \sigma_{\text{ref}}^{i} \sqrt {\pi \alpha^{2} a} $$
(25)

where \( \sigma_{\text{ref}}^{i} \) is the reference stress at the crack growth initiation.

In static assessment, LEFM applies only if “the plastic zone size ahead of the crack tip is ‘small’ compared to the crack size, say for example four times smaller.4 Under this hypothesis, the plastic zone size Rp can be estimated from Irwin’s linear elastic equations and is given by:

$$ R_{p} = \frac{1}{\pi y}\left( {\frac{{K_{\text{I}} }}{{\sigma_{s} }}} \right)^{2} $$
(26)

where y equals 1 for plane stress and 3 for plane strain conditions and σs is the material yield stress.”4

Assuming plane strain conditions and σs = Rp0.2, LEFM Failure Assessment Line applies when

$$ \alpha^{2} a > a_{{0{\text{EP}}}} = \frac{4}{3\pi }\left( {\frac{{K_{\text{IC}} }}{{R_{p0.2} }}} \right)^{2} $$
(27)

At lower equivalent crack lengths, EPFM applies. This means that, instead of (25), the following equation appliesFootnote 2:

$$ K_{\text{IC}} \left( {\frac{{\alpha^{2} a}}{{a_{{0{\text{EP}}}} }}} \right)^{{\frac{N - 1}{{2\left( {N + 1} \right)}}}} = \sigma_{\text{ref}}^{i} \sqrt {\pi \alpha^{2} a} $$
(28)

where N = 1/n is the power law exponent in the Ramberg–Osgood constitutive law

$$ \varepsilon = \frac{\sigma }{E} + \left( {\frac{\sigma }{K}} \right)^{N} $$
(29)

being n the strain hardening exponent in the Hollomon equation

$$ \sigma = {\text{\rm K}}\varepsilon_{pl}^{n} $$
(30)

The strain hardening exponent n is assumed as given by an empirical lower bound value

$$ n = 0.3 \left( {1 - \frac{{R_{p0.2} }}{{R_{m} }}} \right) $$
(31)

as suggested in the literature2,8,22

The meaning of Eq. (28) is that, when the plastic zone beyond the crack tip is large (EPFM), the linear elastically calculated SIF (right side of the equation) at crack growth initiation is lower than KIC. The reduction factor (multiplier of KIC on the left side) depends on:

  • The strain hardening exponent n and/or the Ramberg–Osgood power law exponent N = 1/n;

  • The \( \left( {\frac{{K_{\text{IC}} }}{{R_{p0.2} }}} \right)^{2} \) material characteristic length;

  • The geometric linear elastic crack equivalent length α2a.

The amount of the reduction is predictable, and then it is sufficient for a linear elastic analysis for calculating the load at crack growth initiation under linear elastic plane strain state of stress, even when the component behaves in EPFM mode beyond the crack tip.

Both LEFM and EPFM crack growth initiation lines can be regarded as

$$ J_{i} = \frac{{K_{\text{IC }}^{2} \left( {1 - \nu^{2} } \right)}}{E} = {\text{const}} $$
(32)

condition.

The failure assessment line is represented in Fig. 7.

Figure 7
figure 7

Static Kitagawa diagram

Full size image

For α2a ≥ a0EP, LEFM is dominant and the failure assessment line is given by (25).

For α2a < a0EP, EPFM is dominant and the failure assessment line is given by (28) until the reference stress is smaller than the offset yield strength.

As the load linear elastically increases the computation of the reference stress on the CDE line, we have in D a SIF = KI < KIC in correspondence of which the crack growth initiates. This happens because the elasto-plastic energy in the plastic process zone reaches the material critical value Ji.

Rewriting (28)

$$ K_{r} = \frac{{K^{i} }}{{K_{\text{IC}} }} = \frac{{\sigma_{\text{ref}}^{i} \sqrt {\pi \alpha^{2} a} }}{{K_{\text{IC}} }} = \left( {\frac{{\alpha^{2} a}}{{a_{{ 0 {\text{EP}}}} }}} \right)^{{\frac{N - 1}{{2\left( {N + 1} \right)}}}} $$

Rearranging (27)

$$ \frac{{\alpha^{2} a}}{{a_{{ 0 {\text{EP}}}} }} = \frac{3}{4}\left( {\frac{{K^{i} }}{{\sigma_{\text{ref}}^{i} }}} \right)^{2} \left( {\frac{{R_{p0.2} }}{{K_{\text{IC}} }}} \right)^{2 } = \frac{3}{4}\left( {\frac{{K^{i} }}{{K_{\text{IC}} }}} \right)^{2} \left( {\frac{{R_{p0.2} }}{{\sigma_{\text{ref}}^{i} }}} \right)^{2 } = \frac{3}{4}\left( {\frac{{K_{r} }}{{L_{r} }}} \right)^{2} $$

then

$$ K_{r} = \left[ {\frac{3}{4}\left( {\frac{{K_{r} }}{{L_{r} }}} \right)^{2} } \right]^{{\frac{N - 1}{{2\left( {N + 1} \right)}}}} $$

and finallyFootnote 3

$$ K_{r} = \left( {\frac{3}{4}} \right)^{{\frac{N - 1}{4}}} L_{r}^{{ - \frac{N - 1}{2}}} \quad {\text{in plane strain}} $$
(33)

We get an equivalent form of the EPFM line, linking the linear elastic relative SIF at the crack growth initiation

$$ K_{r} = \frac{{K^{i} }}{{K_{\text{IC}} }} $$
(34)

to the linear elastic relative reference stress at the crack growth initiation

$$ L_{r} = \frac{{\sigma_{\text{ref}}^{i} }}{{R_{p0.2} }} $$
(35)

The change of coordinates is graphically represented in Fig. 8.

Figure 8
figure 8

Static Kitagawa diagram—coordinates change for FAD

Full size image

Figure 9 shows FADs at crack growth initiation compliant with BS 7910:20058 Level 2B (and equivalent SINTAP and R6 procedures), where the relationship between Kr and Lr in plane strain is given by

$$ K_{r} = \left( {\frac{{E\varepsilon_{\text{ref}} }}{{L_{r} R_{p0.2} }} + \frac{{L_{r}^{3} R_{p0.2} }}{{2E\varepsilon_{\text{ref}} }}} \right)^{ - 0.5} $$
(36)

of possible evaluation when the material properties yield strength (P1) and

$$ {\text{Young's modulus}}\;E\quad \quad \left( {P_{4} } \right) $$

and Material Index

$$ N = 1/n\quad \left( {M_{5} } \right) $$
(37)

enable the Ramberg–Osgood constitutive curve to be drawn.

Figure 9
figure 9

FAD as in BS 7910: 2005 Level 2B

Full size image

Two potential collapse modes are identified (BS 7910:20058 Annex P):

  • Collapse of the remaining ligament adjacent to the flaw being assessed—local collapse;

  • Collapse of the structural section containing the flaw—net section collapse.

Collapse of the structure by gross straining—gross section collapse, when collapse takes place away from the flawed section or is unaffected by the presence of the flaw, is not considered by FAD.8

The “reference stress” σref is the stress used for plastic collapse considerations.8

Annex P (normative) of BS 7910:20058 provides formulae for the calculation of σref for local and/or net section collapse for various structural configurations, as a function of the applied load.

Lr, the ratio of the applied load to the yield load, is a measure of the amount of plasticity at the crack tip and shall be limited to a maximum value called “flow stress” or “effective yield after strain hardening”:8

$$ L_{r\,\hbox{max} } = \frac{1}{2}\left( {1 + \frac{{R_{m} }}{{ R_{p0.2} }}} \right)\quad \left( {M_{6} } \right) $$
(38)

M6 is an “Ashby Material Index” distinct from M4, being the latter subjected to a threshold value for the strength ratio.

For most applications, it is acceptable to use engineering stress–strain data, but it is important to concentrate calculation points around Rp0.2:

Lr = 0.7–0.9–0.98–1.0–1.02–1.1–1.2 and intervals of 0.1 up to the “effective yield.”8

Spheroidal graphite materials grades show yield ratios varying in a range as

$$ \frac{{R_{p0.2} }}{{R_{m} }} = 0.58 \div 0.83 $$
(39)

and/or

$$ \frac{{R_{m} }}{{R_{p0.2} }} = 1.71 \div 1.20 $$
(40)

hence

$$ \frac{1}{N} = n = 0.125 \div 0.050 $$
(41)
$$ N = 8 \div 20 $$
(42)

In Fig. 9, the two extreme constitutive curves (42), component independent, are drawn.

The loading pattern of a cracked component, subjected to an applied primary (contributing to yielding) load, is represented by a point (Lr, Kr) positioned on a straight line originated in (0, 0) featured by slope:

$$ \frac{{K_{r} }}{{L_{r} }} = \frac{{K_{\text{I}} }}{{K_{\text{IC}} }}\frac{{R_{p0.2} }}{{\sigma_{\text{ref}} }} = \left( {\frac{{K_{\text{I}} }}{{\sigma_{\text{ref}} }}} \right)\left( {\frac{{R_{p0.2} }}{{K_{\text{IC}} }}} \right) $$
(43)

The slope is given by the contribution of two terms:

  • KI/σref, linear elastically representing geometry, crack, load, assuming plane strain state of stress and von Mises criterion, material independent, and corresponding to the effective crack length α2a in the Kitagawa diagram;

  • Rp0.2/KIC, material contribution corresponding in the Kitagawa diagram to a0EP, the transition crack length from EPFM and LEFM.

Rearranging (43), the following relation can be obtained:

$$ \left( {\frac{{K_{r} }}{{L_{r} }}} \right)^{2} = \left( {\frac{{K_{\text{I}} }}{{\sigma_{\text{ref}} }}} \right)^{2} \left( {\frac{{R_{p0.2} }}{{K_{\text{IC}} }}} \right)^{2} = \pi \left( {\alpha^{2} a} \right)\left( {\frac{{R_{p0.2} }}{{K_{\text{IC}} }}} \right)^{2} = \frac{\pi }{\text{SF}} $$
(44)

We define as “Slope Factor” SF the ratio between the “Material Characteristic Length” (material index)

$$ \left( {\frac{{K_{\text{IC}} }}{{R_{p0.2} }}} \right)^{2} \quad \left( {M_{7} } \right) $$
(45)

and the “Effective Crack Length” α2a.

Let us compare two materials, representing the whole range of SGCIs grades, having any Rp0.2 and any KIC, but different strain hardening exponents n, and consequently different Ramberg–Osgood power law exponents

$$ N = 8\,{\text{the first and}}\,N = 20\;{\text{the second}}, $$

with

$$ R_{m} /R_{p0.2} = 1.71\;{\text{and}}\;1.20\;{\text{respectively}}, $$

and with

$$ L_{r\,\hbox{max} } = 1.36\;{\text{and}}\;1.10\;{\text{respectively}} $$

In Fig. 9, the black and red thick continuous lines are drawn by (36), while the thin ones are drawn considering only the first term (see23).

In Fig. 9, the loading pattern SF = 0.35 ÷ 0.51 (values depending only on W/a) corresponds to the compliance condition 2.5 (KIC/Rp0.2)2 for the minimum ligament W–a in ASTM E399 of a CT specimen.

This result is obtained from the rearranged SINTAP22 equations for an ASTM E399 CT specimen

$$ K_{I} = \frac{P\sqrt a }{{\sqrt {BB_{N} } W}}\left[ {29.6 - 185.5\frac{a}{W} + 655.7\frac{{a^{2} }}{{W^{2} }} - 1017\frac{{a^{3} }}{{W^{3} }} + 638.9\frac{{a^{4} }}{{W^{4} }}} \right] $$
(46)
$$ \sigma_{\text{ref}} = \frac{P}{{\sqrt {B B_{N} } W}}\frac{\sqrt 3 }{{2 \left\{ {\left[ {2.702 + 4.599\left( {\frac{a}{W}} \right)^{2} } \right]^{{\frac{1}{2}}} - 1 - 1.702\left( {\frac{a}{W}} \right)} \right\}}} {\text{Plane Strain, Von Mises}} $$
(47)

Equation (46) gives approximately the same results as ASTM formulas3

$$ K_{I} = \frac{P}{{\sqrt {B B_{N} } W^{1/2} }}f\left( {\frac{a}{W} } \right) $$
(48)
$$ f\left( {\frac{a}{W}} \right) = \frac{{\left( {2 + \frac{a}{W}} \right)}}{{\left( {1 - \frac{a}{W}} \right)^{{\frac{3}{2}}} }}\left( {0.886 + 4.64\frac{a}{W} - 13.32\frac{{a^{2} }}{{W^{2} }} + 14.72\frac{{a^{3} }}{{W^{3} }} - 5.6\frac{{a^{4} }}{{W^{4} }}} \right) $$
(49)

when a/W is in the range 0.4 ÷ 0.7. ASTM does not indicate any expression for the reference stress.

The constitutive ratio KI/σref, in plane strain conditions and using the von Mises yielding criterion, depends only on the crack depth a and ligament ratio a/W and thus derives by a bi-dimensional model.

The loading pattern SF = 2.4 (a constant for all materials) corresponds to the transition between LEFM and EPFM, following the schematic Kitagawa diagram4.

The EPFM regime lasts until the load reaches the value σref = Rp0.2, at a SF depending on the material index Rp0.2/E.

$$ \frac{{R_{p0.2} }}{E}\quad \left( {M_{8} } \right) $$
(50)

This material index is not of interest when selecting a material within the SGCIs family, but it is of interest when the selection is done between SGCIs grades and other material families, such as steels and/or aluminum alloys.

SGCIs considered in this paper can be loaded at σref = Rp0.2 (plane strain crack growth initiation) at SF ranging from a value of 9 for low silicon ferritic DI and 5 for high strength ADI.

At lower equivalent crack lengths, the load could be larger than yield before the plane strain crack growth initiation (PYFM, Post Yield Fracture Mechanics), up to the maximum cutoff value Lr maxRp0.2.

The maximum value is reached when the loading pattern SF crosses the FAD curve at Lr max, SF value depending on M8 and M5:

$$ {\text{SF at}}\; L_{r\hbox{max} } = A \frac{E}{{R_{p0.2} }} + B $$
(51)
$$ A = 0.488 N^{ - 0.815} ; B = 0.0356 N^{2} - 1.1592 N + 14.349 $$

Charpy Test and Plane Strain Fracture Toughness

For a number of years, SGCIs standardization WGs have debated the adoption of the Charpy test inside the material standards. Casting manufactures are concerned that the comparison of Charpy energy values between SGCIs and steels will discourage potential SGCIs casting applications. On the other side, designers that are interested to change material are afraid of unexpected material behaviors, even considering they are not using Charpy values in their calculation codes.

It has been proposed18 that the difference in Charpy energy between the two material families consists in the “shear lips advantage” of steels, undergoing a plane stress state of stress at the external surfaces of the test probe.

From the concept,18 it can be indicated that the main difference between SGCIs and steels is in the post-necking behavior, when common steels show pronounced restriction in area, while SGCIs do not because of spheroids. The material behavior in the post-necking regime is a complicated issue. Its description requires complicated and non-standard approaches based on damage models. This field of investigation is not comprised in the scope neither of this paper nor of the here considered design guidelines; both consider the material behavior up to the damage initiation not exceeding the offset yield strength, where the uniform nonlinear deformation of the quasi-static tensile test constitutive curve applies.

The Charpy V-notch energy value, at a given temperature, is determined by the concurrent contribution of the following material properties:

  • Shear lips advantage/post-necking behavior;

  • Strain rate sensitivity;

  • Fracture toughness.

For steels, correlations are proposed between Charpy V-notched energy values and fracture toughness.

“Where Charpy results are available at the temperature at which Kmat is required, the following equation can be used to estimate fracture toughness:8

$$ K_{\text{mat}} = {\left( {12\sqrt {C_{V} } - 20} \right)\left( {\frac{25}{B}} \right)}^{0.25} + 20 $$
(52)

where Kmat is the estimate of the fracture toughness in MPa√m, B is the thickness of the material for which an estimate of Kmat is required (in mm), CV is the lower bound Charpy V-notch impact energy at the service temperature (in joules).”

Martinez et al.18 proposed also for ADIs a linear correlation between the Material Characteristic Crack Length (KIC/Rp0.2)2 in [mm] and (CV/Rp0.2) or (CU/Rp0.2) in [J/MPa], where CV stays for V-notched Charpy and CU for Un-notched Charpy.Footnote 4

$$ \left( {\frac{{K_{\text{IC}} }}{{R_{p0.2} }}} \right)^{2} = 63.1 \left( {\frac{{C_{U} }}{{R_{p0.2} }}} \right) + 0.66 $$
(53)
$$ \left( {\frac{{K_{\text{IC}} }}{{R_{p0.2} }}} \right)^{2} = 1430.4 \left( {\frac{{C_{V} }}{{R_{p0.2} }}} \right) - 0.589 $$
(54)

Equation (53) is in good agreement with the data of ISO 17804, using for Rp0.2 the minimum value specified for the grade (see Table 1).

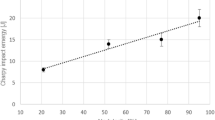
Figure 10 shows the trend lines of V-notched Charpy energy values of SGCIs tested at Zanardi Fonderie laboratory, specimens taken from Y50 [mm] test samples, convenient for fracture toughness testing. Thick lines are related to RT, dotted lines to − 20 °C, and pointed lines to − 40 °C. For FP (red lines), − 20 and − 40 °C lines are coincident. IDILT (low silicon, low temperature) and IDIRT (normal silicon, room temperature) are considered at one average Rp0.2 value. The highest dot is at RT, the lowest dot is at − 40 °C.

Figure 10
figure 10

Trend lines for V-notched Charpy energy values

Full size image

Figure 11 converts the CV data of Fig. 10 into Fracture Toughness data KJIC, using the trend line of the Wallin equation for SGCIs24

$$ K_{\text{JIC }} \approx 180 \left[ {{\text{MPa}} \sqrt m } \right]\left( {\frac{{C_{V } \left[ {\text{J}} \right]}}{{100 \left[ {\text{J}} \right]}}} \right) ^{0.4 } \pm 0.2 K_{\text{JIC}} \left( {2\sigma } \right) $$
(55)
Figure 11
figure 11

Fracture toughness estimated by conversion of V-notched Charpy energy

Full size image

Values from actual or obsolete material standards are also reported in Fig. 11.

Wallin fundamental indications are:

  • SGCIs should have the same correlation between Charpy V and fracture toughness in both the transition and the upper shelf region;

  • It is possible to estimate an equivalent impact energy that gives the same fracture toughness for steel as for SGCIs.

Defining Wallin Steel Equivalent Charpy V-notch WSECV, the impact energy of a steel having the same fracture toughness of a SGCI having a given Charpy V-notch impact energy CV, we get from Table 1 in24 the following best fit correlation

Table 1 Comparison between ISO 17804 KIC data and Eq. 53
Full size table
$$ {\text{WSEC}}_{V} = 4.6661 C_{V}^{0.6944} $$
(56)

Note that (55) matches (52), where CV is substituted by WSECV assuming B ≈ 7 mm which is closed to the 10 × 10 mm size of the standard Charpy specimen.

Two new material properties have to be defined

$$ \begin{aligned} & {\text{Un - notched Charpy Energy Value}}\quad C_{U} \quad \quad \left( {P5} \right) \\ & V{\text{ - notched Charpy Energy Value}}\quad C_{V} \quad \quad \left( {P6} \right) \\ \end{aligned} $$

together with a new material index

$$ {\text{Wallin Steel Equivalent Charpy}}\,V{\text{ - notched Energy Value}}\quad {\text{WSEC}}_{V} \quad \left( {M9} \right) $$

Real Cracked Components

The acceptability of flaws using the FAD has been described considering a reference stress calculated in plane strain conditions and also using a plain strain toughness material property KIC.

The designers (and the component design standards) are also interested to know the grade of safety offered by different materials, in case the essentially linear elastic design and plane strain based calculations are violated on the field. In simple words, the materials’ “forgiveness ability” is of interest.

These kinds of evaluation cannot be referred to the material only but have to consider also the component geometry and loading pattern.

Conclusions

A cube {ΔYS; ΔSR; ΔMQI} of appropriate size on the three dimensions is proposed as identifier of one SGCI grade at the minimum granularity. ΔYS granularity level is defined as in Fig. 1, for ΔSR and ΔMQI it depends on the foundry process capability.

Measured material properties and calculated material indexes, to be used in components design, should be associated with a cube at the minimum granularity, and the position identified in this cube can be used to synthetically identify the mechanical properties of a specific SGCI.

The following material properties and material indexes are of immediate use in design, after a linear elastic FEM has been performed:

Material Properties

P 1 :

Yield strength: Rp0.2

P 2 :

Strength ratio: Rm/Rp0.2

P 3 :

Young’s modulus: E

P 4 :

Plane strain linear elastic fracture toughness: KIC or KjI

P 5 :

Un-notched Charpy energy value: CU (estimate of P4 at different temperatures)

P 6 :

V-notched Charpy energy value: CV (estimate of P4 at different temperatures)

P 7 :

Fatigue strength on a smooth specimen (stress and/or strain controlled)

P 8 :

Fatigue strength on sharply notched/cracked specimen (stress controlled)

Material Indexes

M 1 :

Critical strain maximum allowable Neuber elastic peak in tension, applied to an ideally elastic perfectly plastic material model at TF = 0.33: σSK 1st criterion

M 2 :

Same at TF = 0.67

M 3 :

Same at TF = 1.80

M 4 :

Offset yield strength in tension with threshold P2 ≥ 1.33: Rp,ers

M 5 :

Power law exponent in Ramberg–Osgood constitutive equation: N

M 6 :

Relative offset yield strength in tension without threshold: Lr max

M 7 :

Material characteristic crack length: (KIC/Rp0.2)2

M 8 :

Yield-to-stiffness ratio: Rp0.2/E

M 9 :

Wallin steel equivalent Charpy V-notched energy value: WSECV

Materials properties available from the quasi-static uniaxial tensile test are essentially the Young’s modulus E, the yield strength Rp0.2, the ultimate tensile strength Rm and the elongation at Fracture A5.

Plane strain linear elastic fracture toughness KIC should be measured whenever possible following ASTM E399 requirements, using a test probe taken from a representative test sample (e.g., Y50 [mm]).

When the material ductility is too high for the ASTM E399 requirements, a substitute KjI should be converted from a valid physical crack initiation toughness value (e.g., Ji in accordance with ISO 12135) excluding any crack growth.

For material selection purposes only, the adoption of a substitute Wallin Steel Equivalent Charpy V-notched Energy Value WSECV is proposed.

For material standard normative purposes, the elongation at fracture A5 is considered not directly but as a component of the material quality index MQI, in this way avoiding indeterminateness due to the inverse statistical relationship between Rm and A5.15

The Ultimate Tensile Strength Rm is considered not directly but as a component of the strength ratio (SR), in this way characterizing the manufacturing process and consequently the material properties for design.

In design, the calculated

$$ A_{5} = 10^{4} \frac{\text{MQI}}{{{\text{SR}}^{2} R_{p0.2}^{2} }} $$
(55)

does not enter directly in calculations, being a component of the “strain at failure versus triaxiality factor,” calculated using a standard threshold value at high triaxiality.

Contractual Specifications

Regarding contractual design specifications the following procedure should be recommended:

1. Specify, at given locations on the castings, the minimum and maximum hardness HBWmin and HBWmax;

2. For the actual DI material process and for different thicknesses Y25 Y50 Y75 [mm] calculate the relationships between Rm and Rp0.2 versus HBW (see EN 1564:2011 Annex E, ISO 17804:2005 Annex D);

3. Calculate Rm min, Rm max, Rp0.2 min, Rp0.2 max using the correlations established at point 2:

 a. \( R_{m} = A*{\text{HBW}} + B \) (Eq. 56)

 b. \( R_{p0.2} = \, C*{\text{HBW}} + D \) (Eq. 57)

4. Specify the separately cast test sample Y25 Y50 Y75 [mm] and/or the casting best sounda location where to cut the tensile test specimen. The Brinell hardness measured on the tensile test specimen should be found inside the range HBWmin ÷ HBWmax;

5. Specify the minimum MQI to be obtained from the tensile test.

  1. aSee ISO 1083:2018 paragraph 7.1, ISO 17804:2005 paragraph 7.1, EN 1563:2018 paragraph 7.2.2, EN 1564:2011 paragraph 7.3

The strength ratio (SR) = Rm/Rp0.2 versus HBW is monotonous, then

$$ SR_{\hbox{min} } = \hbox{min} \left( {R_{m\,\hbox{min} } /R_{p0.2\,\hbox{min} } ;R_{m\,\hbox{max} } /R_{p0.2\,\hbox{max} } } \right) $$
(58)

SRmin makes the designer confident versus the FKM offset yield strength Rp, ers (21) and versus the FAD maximum ratio of the applied load to the yield load Lr max (38).

The first criterion for σSK (19) is controlled by

$$ \left( {R_{p0.2} A_{5} } \right)_{\hbox{min} } = {\text{ MQI}}_{\hbox{min} } /\left( {R_{m}^{2} /R_{p0.2} } \right)_{\hbox{max} } $$

The ratio (R2m /Rp0.2) versus HBW is growing monotonous, then

$$ \left( {R_{p0.2} A_{5} } \right)_{\hbox{min} } = {\text{MQI}}_{\hbox{min} } /\left( {R_{m\,\hbox{max} }^{2} /R_{p0.2\,\hbox{max} } } \right) $$
(59)

Equation (59) makes the designer confident versus the FKM critical strain criterion.

Figures 12 and 13 show an example of design confidence based on contractual specification.

Figure 12
figure 12

Relationship between hardness and strength for traditional F FP SGCIs

Full size image
Figure 13
figure 13

FKM component static strength critical strain control using MQI specification

Full size image

Traditional ferritic (excluding high silicon) and ferritic pearlitic grades are considered.

Specifying the HBW range, the designer is confident on the Rm and Rp0.2 ranges and also on the strength ratio (SR) that we can see is not significantly changing in the whole interval being even more uniform in the specified HBW range.

For the required material quality index (MQI), the minimum elongation A5 at the right end of the interval is 64% of the maximum elongation at the left end. But the minimum value controlling the Component Strength limited by the Critical Strain is 89% of the maximum.

One can observe that, from a design point of view, it would be more convenient to directly specify a (Rp0.2A5)min requirement. But this is not the case, because the statistical output of a given foundry process is best described by a relationship (Rem A5) = const and not by (Rfp0.2 A5) = const. It can also be observed that Rm and A5 are close events, while Rp0.2 and A5 are nearly independent events in the uniaxial tensile test flow curve.