Skip to main content
SpringerLink
Log in

N-body Dynamics on an Infinite Cylinder: the Topological Signature in the Dynamics

  • Published:
Regular and Chaotic Dynamics Aims and scope Submit manuscript

Abstract

The formulation of the dynamics of N-bodies on the surface of an infinite cylinder is considered. We have chosen such a surface to be able to study the impact of the surface’s topology in the particle’s dynamics. For this purpose we need to make a choice of how to generalize the notion of gravitational potential on a general manifold. Following Boatto, Dritschel and Schaefer [5], we define a gravitational potential as an attractive central force which obeys Maxwell’s like formulas.

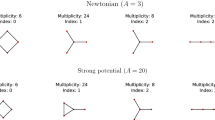
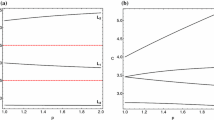
As a result of our theoretical differential Galois theory and numerical study — Poincaré sections, we prove that the two-body dynamics is not integrable. Moreover, for very low energies, when the bodies are restricted to a small region, the topological signature of the cylinder is still present in the dynamics. A perturbative expansion is derived for the force between the two bodies. Such a force can be viewed as the planar limit plus the topological perturbation. Finally, a polygonal configuration of identical masses (identical charges or identical vortices) is proved to be an unstable relative equilibrium for all N > 2.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Arnol’d, V. I., Mathematical Methods of Classical Mechanics, 2nd ed., Grad. Texts in Math., vol. 60, New York: Springer, 1989.

    Book  Google Scholar 

  2. Baird, Ch. S., Helmholtz Decomposition of Vector Fields, http://faculty.uml.edu/cbaird/95.657(2012)/Helmholtz-Decomposition.pdf (2012).

  3. Boatto, S., Curvature Perturbations and Stability of a Ring of Vortices, Discrete Contin. Dyn. Syst. Ser. B, 2008, vol. 10, nos. 2–3, pp. 349–375.

    Article  MathSciNet  Google Scholar 

  4. Boatto, S. and Simó, C., Thomson’s Heptagon: A Case of Bifurcation at Infinity, Phys. D, 2008, vol. 237, nos. 14–17, pp. 2051–2055.

    Article  MathSciNet  Google Scholar 

  5. Boatto, S., Dritschel, D. G., and Schaefer, R. G., N-Body Dynamics on Closed Surfaces: The Axioms of Mechanics, Proc. A, 2016, vol. 472, no. 2192, 20160020, 20 pp.

    MathSciNet  MATH  Google Scholar 

  6. Boatto, S. and Koiller, J., Vortices on Closed Surfaces, in Geometry, Mechanics, and Dynamics, D. E. Chang, D. D. Holm, G. Patrick, T. Ratiu (Eds.), Fields Inst. Commun., vol. 73, New York: Springer, 2015, pp. 185–237.

    Chapter  Google Scholar 

  7. Boatto, S. and Simó, C., A Vortex Ring on a Sphere: The Case of Total Vorticity Equal to Zero, Philos. Trans. R. Soc. Lond. Ser. A Math. Phys. Eng. Sci., 2019, vol. 377, no. 2158, 20190019, 22 pp.

    Article  MathSciNet  Google Scholar 

  8. Borisov, A. V., García-Naranjo, L. C., Mamaev, I. S., and Montaldi, M., Reduction and Relative Equilibria for the Two-Body Problem on Spaces of Constant Curvature, Celestial Mech. Dynam. Astronom., 2018, vol. 130, no. 6, Art. 43, 36 pp.

    Google Scholar 

  9. Borisov, A. V. and Mamaev, I. S., On the Problem of Motion Vortex Sources on a Plane, Regul. Chaotic Dyn., 2006, vol. 11, no. 4, pp. 455–466.

    Article  MathSciNet  Google Scholar 

  10. Borisov, A. V. and Mamaev, I. S., The Restricted Two-Body Problem in Constant Curvature Spaces, Celestial Mech. Dynam. Astronom., 2006, vol. 96, no. 1, pp. 1–17.

    Article  MathSciNet  Google Scholar 

  11. Chorin, A. J. and Marsden, J. E., A Mathematical Introduction to Fluid Mechanics, 3rd ed., Texts Appl. Math., vol. 4, New York: Springer, 1993.

    Book  Google Scholar 

  12. Dritschel, D. G. and Boatto, S., The Motion of Point Vortices on Closed Surfaces, Proc. A, 2015, vol. 471, no. 2176, 20140890, 25 pp.

    MathSciNet  MATH  Google Scholar 

  13. Duarte, G., A dinâmica de 2 corpos sobre a superfície de un cilindro infinito, Master’s Thesis, Rio de Janeiro: Federal University of Rio de Janeiro, 2016.

    Google Scholar 

  14. Falconer, I., Vortices and Atoms in the Maxwellian Era, Philos. Trans. R. Soc. Lond. Ser. A Math. Phys. Eng. Sci., 2019, vol. 377, no. 2158, 20180451, 15 pp.

    Article  MathSciNet  Google Scholar 

  15. Flucher, M. and Gustafsson, B., Vortex Motion in Two-Dimensional Hydrodynamics, Energy Renormalization and Stability of Vortex Pairs, Preprint, https://people.kth.se/gbjorn/vortex.pdf (1997).

  16. Hairer, E., Nørsett, S. P., and Wanner, G., Solving Ordinary Differential Equations: Vol. 1. Nonstiff Problems, 2nd ed., Springer Ser. Comput. Math., vol. 8, New York: Springer, 1993.

    MATH  Google Scholar 

  17. Hansen, E. R., A Table of Series and Products, Upper Saddle River, N.J.: Prentice Hall, 1975.

    MATH  Google Scholar 

  18. Jackson, J. D., Classical Electrodynamics, New York: Wiley, 1998.

    MATH  Google Scholar 

  19. Kirchhoff, G., Vorlesungen über mathematische Physik: Vol. 1. Mechanik, Leipzig: Teubner, 1876.

    MATH  Google Scholar 

  20. Kozlov, V. V. and Harin, A. O., Kepler’s Problem in Constant Curvature Spaces, Celestial Mech. Dynam. Astronom., 1992, vol. 54, no. 4, pp. 393–399.

    Article  MathSciNet  Google Scholar 

  21. Lamb, H., Hydrodynamics, 6th ed., New York: Dover, 1945.

    MATH  Google Scholar 

  22. Landau, L. D. and Lifshitz, E. M., Course of Theoretical Physics: In 10 Vols.: Vol. 6. Fluid Mechanics, 2nd ed., Oxford: Butterworth-Heinemann, 2003.

    Google Scholar 

  23. Loiola, R., Vortex and Mass Dynamics over Hyperbolic Surfaces, Master’s Thesis, Rio de Janeiro: Federal University of Rio de Janeiro, 2016.

    Google Scholar 

  24. Maxwell, J. C., A Treatise on Electricity and Magnetism: Vol. 1, 3rd ed., New York: Dover, 1954.

    MATH  Google Scholar 

  25. Montaldi, J., Soulière, A., and Tokieda, T., Vortex Dynamics on a Cylinder, SIAM J. Appl. Dyn. Syst., 2003, vol. 2, no. 3, pp. 417–430.

    Article  MathSciNet  Google Scholar 

  26. Morales-Ruiz, J. J. and Ramis, J.-P., Galoisian Obstructions to Integrability of Hamiltonian Systems: Statements and Examples, in Hamiltonian Systems with Three or More Degrees of Freedom (S’Agaro, 1995), C. Simó (Ed.), NATO Adv. Sci. Inst. Ser. C Math. Phys. Sci., vol. 533, Dordrecht: Kluwer, 1999, pp. 509–513.

    Chapter  Google Scholar 

  27. Morales-Ruiz, J. J., Ramis, J.-P., and Simó, C., Integrability of Hamiltonian Systems and Differential Galois Groups of Higher Variational Equations, Ann. Sci. École Norm. Sup. (4), 2007, vol. 40, no. 6, pp. 845–884.

    Article  MathSciNet  Google Scholar 

  28. Schaefer, R., A dinâmica de N corpos sobre superfícies: um exemplo para 2 corpos, Master’s Thesis, Rio de Janeiro: Federal University of Rio de Janeiro, 2014.

    Google Scholar 

  29. Shchepetilov, A. V., Calculus and Mechanics on Two-Point Homogeneous Riemannian Spaces, Lecture Notes in Phys., vol. 707, Berlin: Springer, 2006.

    MATH  Google Scholar 

  30. Warner, F. W., Foundations of Differentiable Manifolds and Lie Groups, Grad. Texts in Math., vol. 94, New York: Springer, 1983.

    Book  Google Scholar 

  31. Ziglin, S. L., Branching of Solutions and Nonexistence of First Integrals in Hamiltonian Mechanics: 1, Funct. Anal. Appl., 1982, vol. 16, no. 3, pp. 181–189; see also: Funktsional. Anal. i Prilozhen., 1982, vol. 16, no. 3, pp. 30–41.

    Article  MathSciNet  Google Scholar 

Download references

Acknowledgments

The authors would like to thank Isobel Falconer for very useful historical insights and references. Special thanks to Carles Simó, Rodrigo Schaefer, Umberto Hryniewicz, Sergio Joras and Sonia P. de Carvalho for suggestions and encouragements.

Funding

Jaime Andrade was partially supported by CONICYT (Chile) through FONDECYT project 11180776. Stefanella Boatto was partially supported by the Luís Santaló Visiting Professor fellowship through CRM (Catalonia, Spain). Gladston Duarte was partially supported by a scholarship from the Coordenação de Aperfeiçoamento de Pessoal de Ensino Superior (CAPES, Brazil), through the Graduate Program (Programa de Pos-graduação) of the Mathematical Institute of the Federal University of Rio de Janeiro, and by the María de Maeztu Unit of Excellence in Research Program (MTM-2014-0445) through the Barcelona Graduate School of Mathematics (BGSMath).

Author information

Authors and Affiliations

  1. Departamento de Matemática, Facutad de Ciencias, Universidad del Bii o-Bii o, Casilla 5-C, Concepción, VIII-región, Chile

    Jaime Andrade

  2. Departamento de Matemática Aplicada, Instituto de Matemática, Universidade Federal de Rio de Janeiro, 68530, Rio de Janeiro, RJ, Brazil

    Stefanella Boatto & Gladston Duarte

  3. Barcelona Graduate School of Mathematics & Departament de Matemàtiques i Informática, Universitat de Barcelona, Gran Via de les Corts Catalanes, 585, 08007, Barcelona, Spain

    Stefanella Boatto & Gladston Duarte

  4. Institut de Mathématiques de Bourgogne, Université de Bourgogne, 21078, Dijon, France

    Thierry Combot

  5. Departamento de Fiisica-Matemática, Instituto de Fisica, Universidade Federal de Rio de Janeiro, Rio de Janeiro, RJ, Brazil

    Teresinha J. Stuchi

Authors
  1. Jaime Andrade
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Stefanella Boatto
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Thierry Combot
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Gladston Duarte
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. Teresinha J. Stuchi
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding authors

Correspondence to Jaime Andrade, Stefanella Boatto, Thierry Combot, Gladston Duarte or Teresinha J. Stuchi.

Additional information

Conflict of Interest

The authors declare that they have no conflicts of interest.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Andrade, J., Boatto, S., Combot, T. et al. N-body Dynamics on an Infinite Cylinder: the Topological Signature in the Dynamics. Regul. Chaot. Dyn. 25, 78–110 (2020). https://doi.org/10.1134/S1560354720010086

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1134/S1560354720010086

Keywords

MSC2010 numbers

Search

Navigation