A fractional nonlocal approach to nonlinear blood flow in small-lumen arterial vessels

Abstract

The behavior of human blood flowing in arteries is still an open topic for its multi-phase nature and heterogeneity. In large arterial vessels the well-known Hagen–Poisueille law, which main assumption is that the blood is Newtonian, is considered acceptable. In small arterial vessels, instead, this law does not reproduce experimental results that show non-parabolic profiles of velocity across the vessel diameter. For capillary vessels the Casson model of fluids that is nonlinear is used in place of the Newton law, resulting in nonlinear governing equations and difficulties in mathematical manipulation. For these reasons an alternative approach is proposed in this paper. Starting from the micro-mechanics of blood, the Hagen–Poisueille model is enriched with long-range interactions that simulate the interactions of non-adjacent fluid volume elements due to the presence of red blood cells and other dispersed cells in the plasma. These nonlocal forces are defined as linearly dependent on the product of the volumes of the considered elements and on their relative velocity. Moreover, as the distance between two volume elements increases, the nonlocal forces are scaled through an attenuation function; if this function is chosen as a power law of real order of the distance between the volume elements, an operator related to the fractional derivative of relative velocity appears in the resulting governing equation. It is shown that the fractional Hagen–Poisueille law is able to reproduce experimentally measured profiles of velocity with a great accuracy, moreover as the dimension of the vessel increases, nonlocal forces become negligible and the proposed model reverts to the classical Hagen–Poisueille model.

Appendix: Fractional calculus

In this section, a brief introduction to the fundamentals of fractional calculus will be given.

Consider the function f(x), $x\in {\mathbb {R}}$, the left and the right Riemann–Liouville (RL) fractional integral are defined as [25]:

$$\begin{aligned} \left( I_+^\alpha f\right) (x)= & {} \frac{1}{\varGamma (\alpha )}\int _{-\infty }^x \frac{f(\xi )}{(x-\xi )^{1-\alpha }}d\xi \end{aligned}$$

(34a)

$$\begin{aligned} \left( I_-^\alpha f\right) (x)= & {} \frac{1}{\varGamma (\alpha )}\int _x^\infty \frac{f(\xi )}{(\xi -x)^{1-\alpha }}d\xi \end{aligned}$$

(34b)

while the RL fractional derivative are defined as:

$$\begin{aligned} \left( D_+^\alpha f\right) (x)= & {} \frac{1}{\varGamma (1-\alpha )}\frac{d}{dx}\int _{-\infty }^x \frac{f(\xi )}{(x-\xi )^{\alpha }}d\xi \end{aligned}$$

(35a)

$$\begin{aligned} \left( D_-^\alpha f\right) (x)= & {} -\frac{1}{\varGamma (1-\alpha )}\frac{d}{dx}\int _x^\infty \frac{f(\xi )}{(\xi -x)^{\alpha }}d\xi \end{aligned}$$

(35b)

where $\alpha \in {\mathbb {R}}$, $0\le \alpha \le 1$ and $\varGamma (\cdot )$ is the Euler gamma function. If f(x) is a continuous function with continuous first derivative, the left and right RL fractional derivatives are coincident with the Marchaud fractional derivatives, that may be written as follows:

$$\begin{aligned} \left( \mathbf{D}_+^\alpha f\right) (x)= & {} \frac{\alpha }{\varGamma (1-\alpha )}\int _{-\infty }^x \frac{f(x)-f(\xi )}{(x-\xi )^{\alpha }}d\xi \end{aligned}$$

(36a)

$$\begin{aligned} \left( \mathbf{D}_-^\alpha f\right) (x)= & {} \frac{\alpha }{\varGamma (1-\alpha )}\int _x^\infty \frac{f(x)-f(\xi )}{(\xi -x)^{\alpha }}d\xi \end{aligned}$$

(36b)

The Marchaud fractional derivatives may be defined also for a bounded domain $0\le x\le L$ as:

$$\begin{aligned} \left( \mathbf{D}_{0^+}^\alpha f\right) (x)= & {} \frac{\alpha }{\varGamma (1-\alpha )}\int _0^x \frac{f(x)-f(\xi )}{(x-\xi )^{1+\alpha }}d\xi +\frac{f(x)}{\varGamma (1-\alpha )x^{1+\alpha }} \end{aligned}$$

(37a)

$$\begin{aligned} \left( \mathbf{D}_{L^-}^\alpha f\right) (x)= & {} \frac{\alpha }{\varGamma (1-\alpha )}\int _x^L \frac{f(x)-f(\xi )}{(\xi -x)^{1+\alpha }}d\xi +\frac{f(x)}{\varGamma (1-\alpha )(L-x)^{1+\alpha }} \end{aligned}$$

(37b)

The definitions of Marchaud fractional derivatives related to a single-variable scalar function may be extended to a multi-variable scalar function. The extension is more readable if referred to the Riesz fractional operators. Then it is necessary to introduce Riesz fractional integral $\left( {\bar{I}}^\alpha f\right) (x)$ and derivative $\left( {\bar{D}}^\alpha f\right) (x)$:

$$\begin{aligned} \left( {{\bar{I}}}^\alpha f\right) (x)= & {} \nu (\alpha )\int _{-\infty }^\infty \frac{f(\xi )}{|x-\xi |^{1-\alpha }}d\xi =\nu (\alpha )\left[ \left( I_+^\alpha f\right) (x)+\left( I_-^\alpha f\right) (x)\right] \end{aligned}$$

(38a)

$$\begin{aligned} \left( {{\bar{D}}} ^\alpha f\right) (x) & = \nu (-\alpha )\int _{-\infty }^\infty \frac{f(x-\xi )-f(x)}{|\xi |^{1+\alpha }}d\xi \nonumber \\ & = \varGamma (1-\alpha )\nu (-\alpha )\left[ \left( \mathbf{D}_+^\alpha f\right) (x)+\left( \mathbf{D}_-^\alpha f\right) (x)\right] \end{aligned}$$

(38b)

where $\nu (\pm \alpha )=[2 \cos (\alpha \pi /2)\varGamma (\pm \alpha )]^{-1}$. The Riesz fractional operator may be generalized to multivariate scalar function $f(\mathbf{x})$, with $\mathbf{x}\in {\mathbb {R}}^n$:

$$\begin{aligned} \left( {{\bar{D}}} ^\alpha f\right) (\mathbf{x})=\frac{1}{d_{n,\bar{l}}({{\bar{\alpha }}})}\int _{{\mathbb {R}}^{n}} \frac{f({{\varvec{\xi }}})-f(\mathbf{x})}{||{{\varvec{\xi }}}-\mathbf{x}||^{n+\alpha }}d{{\varvec{\xi }}}= \frac{\chi ({{\bar{\alpha }}})}{d_{n,{{\bar{l}}}}({{\bar{\alpha }}})}\left[ \left( \mathbf{D}_+^\alpha f\right) (\mathbf{x})+\left( \mathbf{D}_-^\alpha f\right) ({{\varvec{x}}})\right] \end{aligned}$$

(39)

where

$$\begin{aligned} d_{n,l}(\alpha )= & {} \beta _n(\alpha )\frac{A_l(\alpha )}{\sin (\alpha \pi /2)} \end{aligned}$$

(40a)

$$\begin{aligned} \beta _n(\alpha )= & {} \frac{\pi ^{1+n/2}}{2^\alpha \varGamma (1+\alpha /2)\varGamma (n+\alpha /2)} \end{aligned}$$

(40b)

$$\begin{aligned} A_l(\alpha )= & {} \sum _{k=0}^{l}(-1)^{k-1}{l\atopwithdelims ()k}k^\alpha \end{aligned}$$

(40c)

and $\chi _l(\alpha )=-A_l(\alpha )\varGamma (\alpha )$, ${{\bar{\alpha }}}=n-1+\alpha$, ${{\bar{l}}}=n-1+l$, $l=\{\alpha \}+1$ and $\{\alpha \}$ is the integer part of $\alpha$. The complete demonstration of Eq. (39) is omitted here for the sake of brevity; more information can be found in [26].

Finally, we briefly introduce the n-dimensional Central Marchaud Fractional Derivative (CMFD) as:

$$\begin{aligned} \left( \mathbf{D}_-^\alpha f\right) ({{\varvec{x}}})=\frac{\alpha }{\varGamma (1-\alpha )}\int _{{\mathbb {R}}^{n}} \frac{f({{\varvec{x}}})-f({{\varvec{\xi }}})}{({{\varvec{\xi }}}-{{\varvec{x}}})^{n+\alpha }}{{\varvec{J}}}_{kj}d{{\varvec{\xi }}} \end{aligned}$$

(41)

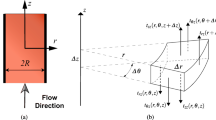
where ${{\varvec{J}}}_{kj}={{\varvec{i}}}_{k}{{\varvec{i}}}_{j}$ is a Jacoby directional tensor, being ${{\varvec{i}}}_{k}$ the unit vector associated with the direction ${{\varvec{x}}}-{{\varvec{\xi }}}$. In the specific problem treated in this paper (the Poiseuille flow), the governing equation written in polar coordinates and in axisymmetric conditions is basically a scalar governing equation, then the Jacoby tensor reduce to unity. This means that the power law attenuation function, responsible for the appearance of fractional operator, reduces in this case to a scalar function. As a consequence, in the governing equation in Eq. (29), the integral term may be recognized as the integral part of the Marchaud fractional derivative defined in bounded domain and reported in Eq. (37). More details can be found in [31].