Skip to main content
SpringerLink
Log in

Generalized Lagrange’s equations for systems with general constraints and distributed parameters

  • Published:
Multibody System Dynamics Aims and scope Submit manuscript

Abstract

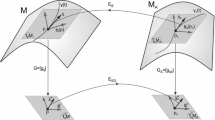
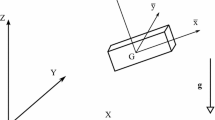
This paper presents a general explicit differential form of Lagrange’s equations for systems with hybrid coordinates and general holonomic and nonholonomic constraints. The appropriate constraint conditions are imposed on d’Alembert–Lagrange principle via Lagrange multipliers to find the correct equations of state of nonholonomic systems. The developed equations take the differential form in terms of the Lagrangian, accounting for the masses and springs that may exist at the boundary. The Lagrangian of hybrid-coordinate systems consists of three parts: the first is due to the rigid-body motion, the second is due to the boundary elements, and the third is the Lagrangian density due to the elastic elements. The developed Lagrange’s equations produce the equations of state and the boundary conditions without the need to carry out the integration by parts generally one needs to do using Hamilton’s principle. Illustrative examples are introduced to show the effectiveness of the developed equations.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4

Similar content being viewed by others

References

  1. Low, K.H., Vidyasagar, M.: A Lagrangian formulation of the dynamic model for flexible manipulator systems. ASME J. Dyn. Syst. Meas. Control 110, 175–181 (1988)

    Article  Google Scholar 

  2. Meirovitch, L.: Hybrid state equations of motion for flexible bodies in terms of quasi-coordinates. J. Guid. Control Dyn. 14(5), 1008–1013 (1991)

    Article  Google Scholar 

  3. Lee, S., Junkins, J.L.: Explicit generalization of Lagrange’s equations for hybrid coordinate dynamical systems. J. Guid. Control Dyn. 15(6), 1443–1452 (1992)

    Article  MathSciNet  Google Scholar 

  4. Estes, A., Majji, M.: Generalization of Lagrange’s equations for constrained hybrid-coordinate systems. J. Guid. Control Dyn. 40(3), 709–712 (2017)

    Article  Google Scholar 

  5. Shabana, A.: An absolute nodal coordinate formulation for the large rotation and deformation analysis of flexible bodies. Technical Report #MBS96-1-UIC, Department of Mechanical Engineering, University of Illinois at Chicago (1996)

  6. Shabana, A.A.: Definition of the slopes and the finite element absolute nodal coordinate formulation. Multibody Syst. Dyn. 1, 339–348 (1997)

    Article  Google Scholar 

  7. Gerstmayr, J., Sugiyama, H., Mikkola, A.: Review on the absolute nodal coordinate formulation for large deformation analysis of multibody systems. J. Comput. Nonlinear Dyn. 8, 031016 (2013)

    Article  Google Scholar 

  8. Karavaev, Yu.L., Kilin, A.A.: The dynamics and control of a spherical robot with an internal omniwheel platform. Regul. Chaotic Dyn. 20(2), 134–152 (2015).

    Article  MathSciNet  Google Scholar 

  9. Hubbard, M.: Lateral dynamics and stability of the skateboard. J. Appl. Mech. 46(4), 931–936 (1979)

    Article  MathSciNet  Google Scholar 

  10. Ostrowski, J., Lewis, A., Murray, R., Burdick, J.: Nonholonomic mechanics and locomotion: the snakeboard example. In: Proc. of the IEEE Internat. Conf. on Robotics and Automation, San Diego, CA, May 1994, pp. 2391–2397 (1994)

    Google Scholar 

  11. Bloch, A.M., Krishnaprasad, P.S., Marsden, J.E., Murray, R.M.: Nonholonomic mechanical systems with symmetry. Arch. Ration. Mech. Anal. 136(1), 21–99 (1996)

    Article  MathSciNet  Google Scholar 

  12. Fedonyuk, V., Tallapragada, P.: The dynamics of a Chaplygin sleigh with an elastic internal rotor. Regul. Chaotic Dyn. 24(1), 114–126 (2019)

    Article  MathSciNet  Google Scholar 

  13. Greenwood, D.T.: Advanced Dynamics. Cambridge University Press, Cambridge (2003)

    Book  Google Scholar 

  14. Flannery, M.R.: The enigma of nonholonomic constraints. Am. J. Phys. 73, 265–272 (2005)

    Article  MathSciNet  Google Scholar 

  15. Pars, L.A.: A Treatise on Analytical Dynamics. Wiley, New York (1965). Reprinted by Oxbow, Woodbridge, CT (1979)

    MATH  Google Scholar 

  16. Lanczos, C.: The Variational Principles of Mechanics, 4th edn. Dover, New York (1970)

    MATH  Google Scholar 

  17. Flannery, M.R.: D’Alembert-Lagrange analytical dynamics for nonholonomic systems. J. Math. Phys. 52, 032705 (2011)

    Article  MathSciNet  Google Scholar 

  18. Desloge, E.A.: The Gibbs–Appell equations of motion. Am. J. Phys. 56, 841–846 (1988)

    Article  MathSciNet  Google Scholar 

  19. Ray, J.R.: Nonholonomic constraints and Gauss’s principle of least constraint. Am. J. Phys. 40, 179–183 (1972)

    Article  Google Scholar 

  20. Flannery, M.R.: The elusive d’Alembert–Lagrange dynamics of nonholonomic systems. Am. J. Phys. 79, 932 (2011)

    Article  Google Scholar 

  21. Jourdain, P.E.B.: Q. J. Pure Appl. Math. 40, 153 (1909)

    Google Scholar 

  22. Papastavridis, J.: On Jourdain’s principle. Int. J. Eng. Sci. 30, 135–140 (1992)

    Article  MathSciNet  Google Scholar 

  23. Abraham, R., Marsden, J.E.: Foundations of Mechanics, 2nd edn. Addison-Wesley, Reading (1994)

    Google Scholar 

  24. Benenti, S.: Geometrical aspects of the dynamics of nonholonomic systems. Rend. Semin. Mat. (Torino) 54, 203–212 (1996)

    MathSciNet  MATH  Google Scholar 

  25. Lewis, A.: The geometry of the Gibbs–Appell equations and Gauss’ principle of least constraint. Rep. Math. Phys. 38, 11–28 (1996)

    Article  MathSciNet  Google Scholar 

  26. Chhabra, R., Emami, M.R.: Nonholonomic dynamical reduction of open-chain multi-body systems: a geometric approach. Mech. Mach. Theory 82, 231–255 (2014)

    Article  Google Scholar 

  27. Chhabra, R., Emami, M.R., Karshon, Y.: Reduction of Hamiltonian mechanical systems with affine constraints: a geometric unification. J. Comput. Nonlinear Dyn. 12(2), 021007 (2017)

    Article  Google Scholar 

  28. Terze, Z., Naudet, J.: Geometric properties of projective constraint violation stabilization method for generally constrained multibody systems on manifolds. Multibody Syst. Dyn. 20, 85–106 (2008)

    Article  Google Scholar 

  29. Terze, Z., Naudet, J.: Structure of optimized generalized coordinates partitioned vectors for holonomic and non-holonomic systems. Multibody Syst. Dyn. 24, 203–218 (2010)

    Article  MathSciNet  Google Scholar 

  30. Lee, T., Leok, M., McClamroch, N.H.: Global Formulations of Lagrangian and Hamiltonian Dynamics on Manifolds: A Geometric Approach to Modeling and Analysis. Springer, Cham (2018). https://doi.org/10.1007/978-3-319-56953-6

    Book  MATH  Google Scholar 

  31. Bloch, A.M., Marsden, J.E., Zenkov, D.: Nonholonomic dynamics. Not. Am. Math. Soc. 52, 324–333 (2005)

    MathSciNet  MATH  Google Scholar 

  32. de León, M.: A historical review on nonholonomic mechanics. Rev. R. Acad. Cienc. Exactas Fís. Nat., Ser. A Mat. 106, 191–224 (2012)

    Article  Google Scholar 

  33. Hertz, H.: The principles of mechanics. In: Jones, D.E., Walley, J.T. (eds.) Introduction by Robert S. Cohen. Dover, New York (1956), xlii+274, preface by H. von Helmholtz; translation by D.E. Jones and J.T. Walley

    Google Scholar 

  34. Chaplygin, S.A.: Selected Works on Mechanics and Mathematics. State Publ. House, Moscow (1954) Technical–Theoretical Literature

    MATH  Google Scholar 

  35. Chaplygin, S.: On the theory of motion of nonholonomic systems. The reducing-multiplier theorem. Regul. Chaotic Dyn. 13(4), 369–376 (2008). English translation of Mat. Sb. 28(1) (1911)

    Article  MathSciNet  Google Scholar 

  36. Neimark, Y., Fufaev, N.A.: Dynamics of Nonholonomic Systems. American Mathematical Society Translations, vol. 33 (1972)

    MATH  Google Scholar 

  37. Bloch, A.M.: Nonholonomic Mechanics and Control. Springer, New York (2015)

    Book  Google Scholar 

  38. Ray, J.R.: Nonholonomic constraints. Am. J. Phys. 34, 406–408 (1966)

    Article  MathSciNet  Google Scholar 

  39. Ray, J.R.: Erratum: nonholonomic constraints. Am. J. Phys. 34, 1202–1203 (1966)

    Article  Google Scholar 

  40. Saletan, E.J., Cromer, A.H.: A variational principle for nonholonomic systems. Am. J. Phys. 38, 892–897 (1970)

    Article  MathSciNet  Google Scholar 

  41. Harvey, P.S.: Rolling Isolation Systems: Modeling, Analysis, and Assessment. PhD Dissertation, Duke University (2013)

  42. Meirovitch, L.: Methods of Analytical Dynamics. McGraw-Hill, New York (1970)

    MATH  Google Scholar 

  43. Timoshenko, S., Gere, J.: Theory of Elastic Stability. McGraw-Hill, New York (1961)

    Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mechanical Engineering, American University of Sharjah, Sharjah, 26666, United Arab Emirates

    Samir A. Emam

  2. Faculty of Engineering, Zagazig University, Zagazig, 44519, Egypt

    Samir A. Emam

Authors
  1. Samir A. Emam
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Samir A. Emam.

Additional information

Publisher’s Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Emam, S.A. Generalized Lagrange’s equations for systems with general constraints and distributed parameters. Multibody Syst Dyn 49, 95–117 (2020). https://doi.org/10.1007/s11044-019-09706-z

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11044-019-09706-z

Keywords

Search

Navigation