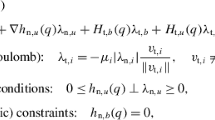Abstract
In this article we analyze the following problem: given a mechanical system subject to (possibly redundant) bilateral and unilateral constraints with set-valued Coulomb’s friction, provide conditions such that the state, which consists of all contacts sticking in both tangential and normal directions, is solvable. The analysis uses complementarity problems, variational inequalities, and linear algebra, hence it provides criteria which are, in principle, numerically tractable. An algorithm and several illustrating examples are proposed.






Similar content being viewed by others
Notes
This is sometimes called rolling friction, or friction without sliding. The expression for the velocities comes from the principle of virtual work.
This kind of transition is often assumed to exist in biped robots control, for instance, when one foot detaches from the ground at the end of a step where both feet are in persistent all-sticking mode.
Vector product is calculated adding 0 as the third component of vectors.
References
Abadie, M.: Dynamic simulation of rigid bodies: modelling of frictional contacts. In: Brogliato, B. (ed.) Impacts in Mechanical Systems. Analysis and Modelling. Lecture Notes in Physics, vol. 551, pp. 61–144. Springer, Berlin (2000)
Acary, V., Brogliato, B.: Numerical Methods for Nonsmooth Dynamical Systems. Lecture Notes in Applied and Computational Mechanics, vol. 35. Springer, Berlin (2008)
Addi, K., Brogliato, B., Goeleven, D.: A qualitative mathematical analysis of a class of linear variational inequalities via semi-complementarity problems. Applications in electronics. Math. Program., Ser. A 126(1), 31–67 (2011)
Adly, S., Nacry, F., Thibault, L.: Preservation of prox-regularity of sets and application to constrained optimization. SIAM J. Optim. 26(1), 448–473 (2016)
Akhadkar, N., Acary, V., Brogliato, B.: Multibody systems with 3D revolute joints with clearances: an industrial case study with an experimental validation. Multibody Syst. Dyn. 42(3), 249–282 (2018)
Anitescu, M., Cremer, J.F., Potra, F.A.: Formulating dynamic multi-rigid-body contact problems with friction as solvable linear complementarity problems. Nonlinear Dyn. 24, 405–437 (1997)
Anitescu, M., Cremer, J.F., Potra, F.A.: On the existence of solutions to complementarity formulations of contact problems with friction. In: Ferris, M.C., Pang, J.S. (eds.) Complementarity and Variational Problems. State of the Art, pp. 12–21. SIAM, Philadelphia (1997)
Aubin, J.P.: Applied Functional Analysis. Wiley, New York (1979)
Audren, H., Kheddar, A.: 3-D robust stability polyhedron in multicontact. IEEE Trans. Robot. 34(2), 388–403 (2018)
Balkcom, D., Trinkle, J.: Computing wrench cones for planar rigid body contact tasks. Int. J. Robot. Res. 21(2), 1053–1066 (2002)
Baraff, D.: Issues in computing contact forces for non-penetrating rigid bodies. Algorithmica 10(2–4), 292–352 (1993)
Bernstein, D.S.: Matrix Mathematics. Theory, Facts, and Formulas with Application to Linear Systems Theory. Princeton University Press, Princeton (2005)
Bertsekas, D.P.: Convex Optimization Theory. Athena Scientific, Belmont (2009)
Bicchi, A.: Hands for dexterous manipulation and robust grasping: a difficulty road toward simplicity. IEEE Trans. Robot. Autom. 16(6), 652–662 (2000)
Blumentals, A., Brogliato, B., Bertails-Descoubes, F.: The contact problem in Lagrangian systems subject to bilateral and unilateral constraints, with or without sliding Coulomb’s friction: a tutorial. Multibody Syst. Dyn. 38, 43–76 (2016)
Bretl, T., Lall, S.: Testing static equilibrium for legged robots. IEEE Trans. Robot. 24(4), 794–807 (2008)
Brogliato, B.: Inertial couplings between unilateral and bilateral holonomic constraints in frictionless Lagrangian systems. Multibody Syst. Dyn. 29, 289–325 (2013)
Brogliato, B.: Kinetic quasi-velocities in unilaterally constrained Lagrangian mechanics with impacts and friction. Multibody Syst. Dyn. 32, 175–216 (2014)
Brogliato, B.: Nonsmooth Mechanics. Models, Dynamics and Control, 3rd edn. Communications and Control Engineering. Springer International Publishing Switzerland, Cham (2016). Erratum/addendum at https://hal.inria.fr/hal-01331565v2
Brogliato, B., Goeleven, D.: Singular mass matrix and redundant constraints in unilaterally constrained Lagrangian and Hamiltonian systems. Multibody Syst. Dyn. 35, 39–61 (2015)
Brogliato, B., Thibault, L.: Existence and uniqueness of solutions for non-autonomous complementarity dynamical systems. J. Convex Anal. 17(3–4), 961–990 (2010)
Caron, S., Pham, Q.C., Nakamura, Y.: ZMP support areas for multicontact mobility under frictional constraints. IEEE Trans. Robot. 33(1), 67–80 (2017)
Chen, X., Xiang, S.: Perturbation bounds of P-matrix linear complementarity problems. SIAM J. Optim. 18(4), 1250–1265 (2007)
Choudhury, D., Horn, R., Pierce, S.: Quasi-positive definite operators and matrices. Linear Algebra Appl. 99, 161–176 (1988)
Cottle, R., Pang, J., Stone, R.: The Linear Complementarity Problem. Computer Science and Scientific Computing. Academic Press, San Diego (1992)
Facchinei, F., Pang, J.S.: Finite-Dimensional Variational Inequalities and Complementarity Problems, vol. I. Operations Research. Springer, New York (2003)
Fraczek, J., Wojtyra, M.: On the unique solvability of a direct dynamics problem for mechanisms with redundant constraints and Coulomb friction. Mech. Mach. Theory 46, 312–334 (2011)
Gholami, F., Nasri, M., Kovecses, J., Teichmann, M.: A linear complementarity formulation for contact problems with regularized friction. Mech. Mach. Theory 105, 568–582 (2016)
Glocker, C.: Set-Valued Force Laws: Dynamics of Non-Smooth Systems. Springer, Berlin (2001)
Glocker, C., Pfeiffer, F.: Dynamical systems with unilateral contacts. Nonlinear Dyn. 3(4), 245–259 (1992)
Glocker, C., Pfeiffer, F.: Complementarity problems in multibody systems with planar friction. Arch. Appl. Mech. 63(7), 452–463 (1993)
Goeleven, D.: Complementarity and Variational Inequalities in Electronics. Mathematical Analysis and Its Applications. Academic Press, San Diego (2017)
Han, L., Trinkle, J., Li, Z.X.: Grasp analysis as linear matrix inequality problems. IEEE Trans. Robot. Autom. 16(6), 663–674 (2000)
Higashimori, M., Kimura, M., Ishii, I., Kaneko, M.: Dynamic capturing strategy for a 2-D stick-shaped object based on friction independent collision. IEEE Trans. Robot. 23(3), 541–552 (2007)
Hiriart-Urruty, J.B., Lemaréchal, C.: Fundamentals of Convex Analysis. Grundlehren Text Editions. Springer, Berlin (2001)
de Jalón, J.G., Gutteriez-Lopez, M.D.: Multibody dynamics with redundant constraints and singular mass matrix: existence, uniqueness, and determination of solutions for accelerations and constraint forces. Multibody Syst. Dyn. 30(3), 311–341 (2013)
de Jalón, J.G., Unda, J., Avello, A.: Natural coordinates for the computer analysis of multibody systems. Comput. Methods Appl. Mech. Eng. 56(3), 309–327 (1986)
Klepp, H.J.: Existence and uniqueness of solutions for accelerations for multibody systems with friction. Z. Angew. Math. Mech. 75(1), 679–689 (1995)
Laulusa, A., Bauchau, O.A.: Review of classical approaches for constraint enforcement in multibody systems. J. Comput. Nonlinear Dyn. 3(1), 011004 (2008)
Leine, R.I., Glocker, C.: A set-valued force law for spatial Coulomb–Contensou friction. Eur. J. Mech. A, Solids 22(2), 193–216 (2003)
Leine, R.I., van de Wouw, N.: Stability and Convergence of Mechanical Systems with Unilateral Constraints. Lecture Notes in Applied and Computational Mechanics, vol. 36. Springer, Berlin (2008)
Lötstedt, P.: Coulomb friction in two-dimensional rigid body systems. Z. Angew. Math. Mech. 61, 605–615 (1981)
Lötstedt, P.: Mechanical systems of rigid bodies subject to unilateral constraints. SIAM J. Appl. Math. 42(2), 281–296 (1982)
Ma, S., Wang, T.: Planar multiple-contact problems subject to unilateral and bilateral kinetic constraints with static Coulomb friction. Nonlinear Dyn. 94, 99–121 (2018)
Moreau, J.J.: Application of convex analysis to some problems of dry friction. In: Zorski, H. (ed.) Trends in Applications of Pure Mathematics to Mechanics, vol. 2, pp. 263–280. Pitman, London (1979)
Negrut, D., Serban, R., Tasora, A.: Posing multibody dynamics with friction and contact as a differential complementarity problem. J. Comput. Nonlinear Dyn. 13, 014503 (2018)
Nikolic, M., Borovac, B., Rakovic, M.: Dynamic balance preservation and prevention of sliding for humanoid robots in the presence of multiple spatial contacts. Multibody Syst. Dyn. 42, 197–218 (2018)
Or, Y., Rimon, E.: Analytic characterization of a class of three-contact frictional equilibrium postures in three-dimensional gravitational environments. Int. J. Robot. Res. 29(1), 3–22 (2010)
Or, Y., Rimon, E.: Characterization of frictional multi-legged equilibrium postures on uneven terrains. Int. J. Robot. Res. 36(1), 105–128 (2017)
Panagiotopoulos, P.D.: Inequality Problems in Mechanics and Applications. Convex and Nonconvex Energy Functions. Birkhäuser, Basel (1985)
Panagiotopoulos, P.D., Al-Fahed, A.M.: Robot hand grasping and related problems: optimal control and identification. Int. J. Robot. Res. 13(2), 127–136 (1994)
Pang, J., Trinkle, J.: Stability characterization of rigid body contact problems with Coulomb friction. Z. Angew. Math. Mech. 80(10), 643–663 (2000)
Pang, J.S., Trinkle, J.C.: Complementarity formulation and existence of solutions of dynamic rigid-body contact problems with Coulomb friction. Math. Program. 73(2), 199–226 (1996)
Pang, J.S., Trinkle, J.C., Lo, G.: A complementarity approach to a quasistatic multi-rigid-body contact problem. J. Comput. Optim. Appl. 5(2), 139–154 (1996)
Pekal, M., Fraczek, J.: Comparison of selected formulations for multibody systems dynamics with redundant constraints. Arch. Mech. Eng. LXII(1), 93–112 (2016)
Pfeiffer, F.: Non-smooth engineering dynamics. Meccanica 51(12), 3167–3184 (2016)
Pfeiffer, F., Glocker, C.: Multibody Dynamics with Unilateral Contacts. Nonlinear Science. Wiley, New York (1996)
Rockafellar, R.: Convex Analysis. Princeton University Press, Princeton (1970)
Rohde, C.A.: Generalized inverses of partitioned matrices. J. Soc. Ind. Appl. Math. 13(4), 1033–1035 (1965)
Seifried, R.: Dynamics of Underactuated Multibody Systems. Solid Mechanics and Its Applications. Springer International Publishing Switzerland, Cham (2014)
Seon, J.A., Dahmouche, R., Gauthier, M.: Enhance in-hand dexterous micromanipulation by exploiting adhesion forces. IEEE Trans. Robot. 34(1), 113–125 (2018)
Shi, J., Woodruff, J., Umbanhowar, P., Lynch, K.: Dynamic in-hand sliding manipulation. IEEE Trans. Robot. 33(4), 778–795 (2017)
Simeon, B.: Computational Flexible Multibody Dynamics. A Differential-Algebraic Approach. Differential-Algebraic Equations Forum. Springer, Berlin (2013)
Studer, C., Glocker, C.: Representation of normal cone inclusion problems in dynamics via non-linear equations. Arch. Appl. Mech. 76(5), 327–348 (2006)
Tasora, A., Anitescu, M.: A matrix-free cone complementarity approach for solving large-scale, nonsmooth, rigid body dynamics. Comput. Methods Appl. Mech. Eng. 200(5–8), 439–453 (2011)
Tasora, A., Anitescu, M.: A complementarity-based rolling friction model for rigid contacts. Meccanica 48(7), 1643–1659 (2013)
Tian, Y., Takane, Y.: Schur complements and Banachiewicz--Schur forms. Electron. J. Linear Algebra 13, 405–418 (2005)
Trinkle, J.C.: On the stability and instantaneous velocity of grasped frictionless objects. IEEE Trans. Robot. Autom. 8(5), 560–572 (1992)
Trinkle, J.C., Pang, J.S., Sudarsky, S., Lo, G.: On dynamic multi-rigid-body contact problems with Coulomb friction. J. Appl. Math. Mech./Z. Angew. Math. Mech. 77(4), 267–279 (1997)
Trinkle, J.C., Tzitzouris, J.A., Pang, J.S.: Dynamic multi-rigid-body systems with concurrent distributed contacts: theory and examples. Philos. Trans., Math. Phys. Eng. Sci. 359(1789), 2575–2593 (2001)
Vanderbei, R., Carpenter, T.: Symmetric indefinite systems for interior point methods. Math. Program. 58(1–3), 1–32 (1993)
Varkonyi, P.L., Or, Y.: Lyapunov stability of a rigid body with two frictional contacts. Nonlinear Dyn. 88, 363–393 (2017)
Wojtyra, M.: Joint reactions in rigid body mechanisms with dependent constraints. Mech. Mach. Theory 44, 2265–2278 (2009)
Wojtyra, M.: Modeling of static friction in closed-loop kinetic chains–uniqueness and parametric sensitivity. Multibody Syst. Dyn. 39, 337–361 (2017)
Wojtyra, M.: The Moore–Penrose inverse approach to modeling of multibody systems with redundant constraints. In: Uhl, T. (ed.) Advances in Mechanisms and Machine Science, Mechanisms and Machine Science, vol. 73, pp. 3087–3096. Springer Nature Switzerland AG, Cham (2019)
Wojtyra, M., Fraczek, J.: Comparison of selected methods of handling redundant constraints in multibody systems simulations. J. Comput. Nonlinear Dyn. 8, 021,007 (2013)
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher’s Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Appendices
Appendix A: Useful results from linear algebra
The first part of this lemma is taken from [59], the second part is [12, Fact 6.4.29], see also [67, Eq. (1.5)].
Lemma 3
Letbe symmetric and positive semidefinite. Assume that\(Q=B-C^{\top }A^{\dagger }C\)is nonsingular. Then the Moore–Penrose generalized inverse of\(M\)is given by
When\(B=0\)one obtains with\(E=A+CC^{\top }\)and\(D=C^{\top }E^{ \dagger }C\):
Proposition 11
([12, Proposition 6.1.7])
Let\(A \in \mathbb{R}^{n \times m}\)and\(b \in \mathbb{R}^{n}\). Then the two statements are equivalent:
- (i)
There exists a vector\(x \in \mathbb{R}^{m}\)satisfying\(Ax=b\).
- (ii)
\(AA^{\dagger }b=b\).
If (i) or (ii) is satisfied, then for all\(y \in \mathbb{R}^{m}\), \(x=A^{\dagger }b+(I-A^{\dagger }A)y\)satisfies\(Ax=b\), and\(y=0\)minimizes\(x^{\top }x\).
Appendix B: Well-posedness of variational inequalities
The next results use the notions of recession functions and cones, which we briefly introduce now (see [20, 32] for illustrating examples). Let \(f: \mathbb{R}^{n} \rightarrow \mathbb{R} \cup \{+\infty \}\) be a proper convex and lower semicontinuous function, we denote by \(\mathrm{dom}(f) \stackrel{\Delta }{=} \{ x \in \mathbb{R}^{n} \mid f(x) < +\infty \}\) the domain of the function \(f(\cdot )\). The epigraph of \(f(\cdot )\) is the set \(\mathrm{epi}(f) \stackrel{ \Delta }{=} \{ (x,\alpha ) \in \mathbb{R}^{n} \times \mathbb{R}\mid \alpha \geq f(x) \}\). The Fenchel transform \(f^{\star }(\cdot )\) of \(f(\cdot )\) is the proper, convex, and lower semicontinuous function defined by
The subdifferential \(\partial f(x)\) of \(f(\cdot )\) at \(x \in \mathbb{R}^{n}\) is defined by
We denote by \(\mathrm{Dom}(\partial f) \stackrel{\Delta }{=} \{ x \in \mathbb{R}^{n} \mid \partial f(x) \neq \emptyset \}\) the domain of the subdifferential operator \(\partial f: \mathbb{R}^{n} \rightarrow \mathbb{R}^{n}\). Recall that (see, e.g., Theorem 2, Chap. 10, Sect. 3 in [8]): \(\mathrm{Dom}(\partial f) \subset {\mathrm{dom}}(f)\).
Let \(x_{0}\) be any element in the domain \(\mathrm{dom}(f)\) of \(f(\cdot )\), the recession function \(f_{\infty }(\cdot )\) of \(f(\cdot )\) is defined by
The function \(f_{\infty }: \mathbb{R}^{n} \rightarrow \mathbb{R} \cup \{ +\infty \}\) is a proper convex and lower semicontinuous function which describes the asymptotic behavior of \(f(\cdot )\).
Let \(K \subset \mathbb{R}^{n}\) be a nonempty closed convex set. Let \(x_{0}\) be any element in \(K\). The recession cone of \(K\) is defined by
The set \(K_{\infty }\) is a nonempty closed convex cone that is described in terms of the directions which recede from \(K\). The indicator function of a set \(K \subseteq \mathbb{R}^{n}\) is \(\psi _{K}(x)=0\) if \(x \in K\), \(\psi _{K}(x)=+\infty \) if \(x \notin K\). If \(K\) is closed, nonempty and convex, we have \(\partial \psi _{K}(x)=\mathcal{N}_{K}(x)\), the so-called normal cone to \(K\) at \(x\), defined as \(\mathcal{N}_{K}(x)=\{v \in \mathbb{R}^{n} \mid v^{\top }(s-x) \leq 0\mbox{ for all} {s \in K}\}\). When \(K\) is finitely represented, i.e., \(K=\{x \in \mathbb{R}^{n} \mid k_{i}(x) \geq 0, 1\leq i \leq m\}\), and if the functions \(k_{i}(\cdot )\) satisfy some constraint qualification (like, independency, or extensions like the MFCQ, see Appendix C), then \(\mathcal{N}_{K}(x)\) is generated by the outwards normals at the active constraints \(k_{i}(x)=0\), i.e., \(\mathcal{N} _{K}(x)=\{v \in \mathbb{R}^{n} \mid v=-\lambda _{i} \nabla k_{i}(x), k _{i}(x)=0, \lambda _{i} \geq 0\}\).
Let us here recall some important properties of the recession function and recession cone (see, e.g., [13, Proposition 1.4.8]:
Proposition 12
The following statements hold:
- (a)
Let\(f_{1}: \mathbb{R}^{n} \rightarrow \mathbb{R}\cup \{ +\infty \}\)and\(f_{2}: \mathbb{R}^{n} \rightarrow \mathbb{R}\cup \{ +\infty \}\)be two proper, convex, and lower semicontinuous functions. Suppose that\(f_{1}+f_{2}\)is proper. Then for all\(x \in \mathbb{R}^{n}\): \((f_{1}+ f_{2})_{\infty }(x) = (f_{1})_{\infty }(x)+ (f_{2})_{\infty }(x)\).
- (b)
Let\(f: \mathbb{R}^{n} \rightarrow \mathbb{R}\cup \{ +\infty \}\)be a proper, convex, and lower semicontinuous function and let\(K\)be a nonempty closed convex set, such that\(f+\varPsi _{K}\)is proper (equivalently\(\mathrm{dom}(f) \cap K\)is nonempty). Then for all\(x \in \mathbb{R}^{n}\): \((f+\varPsi _{K})_{\infty }(x) = f_{\infty }(x) + (\varPsi _{K})_{\infty }(x)\).
- (c)
Let\(K \subset \mathbb{R}^{n}\)be a nonempty, closed, and convex set. Then for all\(x \in \mathbb{R}^{n}\): \((\varPsi _{K})_{\infty }(x) = \varPsi _{K_{\infty }}(x)\). Moreover, for all\(x \in K\)and\(e \in K_{ \infty }\), \(x+e \in K\).
- (d)
If\(K \subset \mathbb{R}^{n}\)is a nonempty, closed, and convex cone, then\(K_{\infty }= K\).
- (e)
Let\(K=P(a,b)\stackrel{\Delta }{=}\{x \in \mathbb{R}^{n} \mid Ax \geq b\}\)for\(A \in \mathbb{R}^{m \times n}\)and\(b \in \mathbb{R}^{m}\). If\(K \neq \emptyset \)then\(K_{\infty }=P(A,0)=\{x \in \mathbb{R}^{n} \mid Ax \geq 0\}\).
- (f)
\(K \subset \mathbb{R}^{n}\)is a nonempty, closed, convex, and bounded set if and only if\(K_{\infty }=\{0_{n}\}\).
Let us now concatenate [3, Theorem 3, Corollaries 3 and 4]. They concern variational inequalities of the form: Find \(u \in \mathbb{R}^{n}\) such that
where \(\mathbf{M} \in \mathbb{R}^{n \times n}\) is a real matrix, \(\mathbf{q} \in \mathbb{R}^{n}\) a vector and \(\varphi : \mathbb{R} ^{n} \rightarrow \mathbb{R}\cup \{+\infty \}\) a proper convex and lower semicontinuous function. The analogy with the generalized equation in (36) is clear taking \(\varphi (\cdot )= \varPsi _{\tilde{K}}( \cdot )\), thus restricting the variation of \(v\) to \(\tilde{K}\), \(\mathbf{q}=F(q,\dot{q},t)\) and \(\mathbf{M}=M(q)\).
The problem in (69) is denoted as \(VI(\mathbf{M},\mathbf{q}, \varphi )\) in the next proposition. We also set
Note that \((\mathrm{dom}(\varphi _{\infty }))^{\star }\) is the dual cone of the domain of the recession function \(\varphi _{\infty }\) while \((\mathrm{dom}(\varphi ))_{\infty }\) is the recession cone of \(\mathrm{dom}(\varphi )\).
Proposition 13
([3])
Let\(\varphi : \mathbb{R}^{n} \rightarrow \mathbb{R}\cup \{ +\infty \}\)be a proper, convex, and lower semicontinuous function with closed domain, and suppose that\(\mathbf{M} \in \mathbb{R}^{n \times n}\)is positive semidefinite (not necessarily symmetric).
- (a):
If\((\mathrm{dom}(\varphi ))_{\infty }\cap {\mathrm{ker}}\{ \mathbf{M}+\mathbf{M}^{\top } \} \cap {\mathcal{K}}(\mathbf{M},\varphi ) = \{ 0 \}\)then for each\(\mathbf{q} \in \mathbb{R}^{n}\), problem\(VI(\mathbf{M},\mathbf{q},\varphi )\)has at least one solution.
- (b):
Suppose that\((\mathrm{dom}(\varphi ))_{\infty }\cap {\mathrm{ker}} \{ \mathbf{M}+\mathbf{M}^{\top }\} \cap {\mathcal{K}}(\mathbf{M}, \varphi ) \neq \{ 0 \}\). If there exists\(x_{0} \in {\mathrm{dom}}( \varphi )\)such that
$$ \bigl\langle \mathbf{q}-\mathbf{M}^{\top }x_{0},v \bigr\rangle + \varphi _{ \infty }(v) > 0, \ \forall v \in \mathrm{dom}( \varphi )_{\infty } \cap {\mathrm{ker}} \bigl\{ \mathbf{M}+ \mathbf{M}^{\top } \bigr\} \cap {\mathcal{K}}( \mathbf{M},\varphi ), \ v \neq 0, $$(71)then problem\(VI(\mathbf{M},\mathbf{q},\varphi )\)has at least one solution.
- (b′):
If\(\mathbf{M}=\mathbf{M}^{\top }\)then one can take\(x_{0}=0\)in (b).
- (c):
If\(u_{1}\)and\(u_{2}\)denote two solutions of problem\(VI( \mathbf{M},\mathbf{q},\varphi )\)then\(u_{1} - u_{2} \in \mathrm{ker}\{ \mathbf{M}+\mathbf{M}^{\top } \}\).
- (d):
If\(\mathbf{M}=\mathbf{M}^{\top }\)and\(u_{1}\)and\(u_{2}\)denote two solutions of problem\(VI(\mathbf{M},\mathbf{q},\varphi )\), then\(\langle \mathbf{q},u_{1}-u_{2} \rangle = \varphi (u_{2})-\varphi (u _{1})\).
- (e):
If\(\mathbf{M}=\mathbf{M}^{\top }\)and\(\varphi (x+z) = \varphi (x)\)for all\(x \in {\mathrm{dom}}(\varphi )\)and\(z \in \mathrm{ker}\{ \mathbf{M}\}\)and\(\langle \mathbf{q},e \rangle \neq 0\)for all\(e \in \mathrm{ker}\{ \mathbf{M} \}\), \(e \neq 0\), then problem\(VI(\mathbf{M},\mathbf{q},\varphi )\)has at most one solution.
Notice that the function \(\varphi (\cdot )\) will never be strictly convex in our case (it is an indicator function) so that the strict convexity argument of [3, Theorem 5] which applies when \(\mathbf{M}\) is a \(P_{0}\)-matrix never holds.
Appendix C: Some convex analysis and complementarity theory tools
Theorem 1
([25, Theorem 3.8.6])
Let\(M \in \mathbb{R}^{n \times n}\)be copositive and let\(q \in \mathbb{R}^{n}\)be given. If the implication\(0 \leq v \perp Mv \geq 0 \Rightarrow v^{\top }q \geq 0\)is valid, then the\(\textit{LCP}(M,q)\)is solvable.
Let \(\mathcal{Q}_{M}\) denote the solution set of the homogeneous LCP. This theorem can be restated equivalently as: \(v \in {\mathcal{Q}} _{M} \Rightarrow q \in {\mathcal{Q}}_{M}^{\star }\).
The next corollary is proved in [18], and is a consequence of results in [23].
Corollary 4
Let\(D=P+N\), where\(D\), \(P\)and\(N\)are\(n \times n\)real matrices, and\(P \succ 0\), not necessarily symmetric. If
then\(D \succ 0\).
If \(K \subset \mathbb{R}^{n}\) is a set, then \(K^{\star }=\{z \in \mathbb{R}^{n} \mid \langle z,x \rangle \geq 0\mbox{ for all }x \in K\}\) is its dual cone. Let \(K\) be a closed convex cone, then
Let \(M=M^{\top } \succ 0\), \(x\) and \(y\) two vectors, then
We note that this is a particular case of (69), so that Proposition 13 can be considered as the characterization of a generalized projection operator \(VI(\mathbf{M}, \mathbf{q},\varphi )\).
In this paper we deal with nonconvex sets, for which it is needed to define suitable notions of normal and tangent cones. The so-called Mangasarian–Fromovitz constraint qualification (MFCQ) [26, pp. 17, 252] is also used.
Definition 1
(The MFCQ)
Let \(K\) be a finitely represented set, i.e., \(K=\{x \in \mathbb{R}^{n} \mid h_{i}(x) \geq 0, 1 \leq i \leq m \}\), and let \(\mathcal{J}(x)=\{ i \in \{1,m\} \mid h_{i}(x)=0\}\) be the set of active constraints indices. The continuously differentiable functions \(h_{i}: \mathbb{R}^{n} \rightarrow \mathbb{R}\), satisfy the MFCQ at \(x\) if there exists a vector \(v \in \mathbb{R}^{n}\) such that \(\nabla h_{i}(x)^{\top }v >0\) for all \(i \in {\mathcal{J}}(x)\).
Under the MFCQ, Clarke’s normal cone of prox-regular sets which are finitely represented by inequalities can be expressed in the so-called linearized form, using the normals to the constraint at the active points, as follows. Let \(K=\{x \in \mathbb{R}^{n} \mid h(x) \geq 0\}\). Suppose that the functions \(h_{i}: \mathbb{R}^{n} \rightarrow \mathbb{R}\) are continuously differentiable and satisfy the MFCQ. Then Clarke’s normal cone to \(K\) at \(x\) is equal to \(\mathcal{N}_{K}=\{w \in \mathbb{R}^{n} \mid w=-\sum_{i \in {\mathcal{J}}(x)}\lambda _{i} \nabla h_{i}(x),\ \lambda _{i} \geq 0 \}=- (\mathcal{T}_{K}(x) ) ^{\star }\), with Clarke’s tangent cone equal to \(\mathcal{T}_{K}=\{z \in \mathbb{R}^{n} \mid z^{T}\nabla h_{i}(x) \geq 0,\mbox{ for all }i \in {\mathcal{J}}(x) \}\). In the case of the set \(S_{\mathrm{n},u}^{st}\) in Sect. 3.2, \(\mathcal{N}_{S _{\mathrm{n},u}^{st}}(q)\) is the cone generated by \(-\nabla h_{ \mathrm{n},u,i}^{st}(q)\) for active constraints \(h_{\mathrm{n},u,i} ^{st}(q)=0\). If \(K\) is closed convex this coincides with the definitions of convex analysis.
Appendix D: Dynamics of the RB + pendulum system
The kinetic energy is given by
where \(I_{p}\) is the pendulum moment of inertia at its gravity center (supposed to be at the middle of the bar), \(\bar{m}\) is its mass. From the Lagrange equations (the potential energy is supposed to be null, hence the Lagrangian function is the kinetic energy), the term \(\frac{d}{dt} (\frac{\partial T}{\partial \dot{q}} )\) yields the inertia matrix
The Coriolis and centripetal forces \(C(q,\dot{q})\dot{q}\) can be computed from the remaining terms in \(\frac{d}{dt} (\frac{\partial T}{\partial \dot{q}} )\) and in \(-\frac{\partial T}{\partial q}\) such that \(C(q,\dot{q})+C^{\top }(q,\dot{q})=\frac{d}{dt} M(q)= (\frac{ \partial m_{ij}(q)}{\partial q}\dot{q} )_{ij}\) if the Christoffel’s symbols are used. One has:
We therefore deduce the vector of inertial plus external generalized forces:
Rights and permissions
About this article
Cite this article
Brogliato, B., Kovecses, J. & Acary, V. The contact problem in Lagrangian systems with redundant frictional bilateral and unilateral constraints and singular mass matrix. The all-sticking contacts problem. Multibody Syst Dyn 48, 151–192 (2020). https://doi.org/10.1007/s11044-019-09712-1
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11044-019-09712-1