Abstract
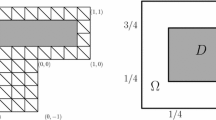
In solving elliptic problems by the finite element method in a bounded domain which has a re-entrant corner, the rate of convergence can be improved by adding a singular function to the usual interpolating basis. When the domain is enclosed by line segments which form a corner of π/2 or 3π/2, we have obtained an explicit a prioriH 10 error estimation ofO(h) and anL 2 error estimation ofO(h 2) for such a finite element solution of the Poisson equation. Particularly, we emphasize that all constants in our error estimates are numerically determined, which plays an essential role in the numerical verification of solutions to non-linear elliptic problems.
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
References
R.A. Adams, Sobolev Spaces. Academic Press, New York, 1975.
I. Babuska, R.B. Kellog and J. Pitkaranta, Direct and inverse error estimates for finite elements with mesh refinement. Numer. Math.,33 (1979), 447–471.
H. Blum and M. Dobrowolski, On finite element methods for elliptic equations on domains with corners. Computing,28 (1982), 53–63.
S.C. Brenner, Multigrid methods for the computation of singular solutions and stress intensity factors, I: Corner singularities. Math. Comp.,68 (1999), 559–583.
Z. Cai and S. Kim, A finite element method using singular function for the Poisson equation: corner singularities. SIAM J. Numer. Anal.,39 (2001), 286–299.
P.G. Ciarlet, The Finite Element Method for Elliptic Problems. North-Holland, Amsterdam, 1978.
M. Dauge, S. Nicaise, M. Bourland and M.S. Lubuma, Coefficients of the singularities for elliptic boundary value problems on domains with conical points, III: Finite element methods on polygonal domains. SIAM J. Numer. Anal.,29 (1992), 136–155.
G. Fix, S. Gulati and G.I. Wakoff, On the use of singular functions with the finite element method. J. Comp. Phys.,13 (1973), 209–228.
P. Grisvard, Elliptic Problems in Nonsmooth Domains. Pitman Publishing, Boston, 1985.
P. Grisvard, Singularities in Boundary Value Problems. RMA,22, Masson, Paris, 1992.
O.A. Ladyzhenskaya, The Mathematical Theory of Viscous Incompressible Flows. Gordon and Breach, 1969.
M.T. Nakao, A numerical verification method for the existence of weak solutions for non-linear boundary value problems. J. Math. Anal. Appl.,164 (1992), 489–507.
M. Schultz, Spline Analysis. Prentice-Hall, 1973.
M. Tabata and M. Yamaguti, Approximate solution of the second order elliptic differential equation in a domain with piecewise smooth boundary by the finite-element method using singularity functions. Theor. and Appl. Mech.,22 (1974), 165–173.
H. Takahasi and M. Mori, Double exponential formulas for numerical integration. Publ. Res. Inst. Math. Soc.,9 (1974), 721–741.
N. Yamamoto and M.T. Nakao, Numerical verifications of solutions for elliptic equations in nonconvex polygonal domains. Numer. Math.,65 (1993), 503–521.
Y. Watanabe and M.T. Nakao, Numerical verifications of solutions for nonlinear elliptic equations. Japan J. Indust. Appl. Math.,10 (1993), 165–178.
Author information
Authors and Affiliations
Corresponding author
Additional information
This research is partly supported by the 21st Century COE program of Faculty of Mathematics, Kyushu University and Grant-in-Aid for Young Scientists (B), 19740052 by the Ministry of Education, Science, Sports and Culture.
About this article
Cite this article
Kobayashi, K. A constructive a priori error estimation for finite element discretizations in a non-convex domain using singular functions. Japan J. Indust. Appl. Math. 26, 493–516 (2009). https://doi.org/10.1007/BF03186546
Received:
Revised:
Issue Date:
DOI: https://doi.org/10.1007/BF03186546