Skip to main content
SpringerLink
Log in

Bifurcation Analysis for a Delayed Diffusive Logistic Population Model in the Advective Heterogeneous Environment

  • Published:
Journal of Dynamics and Differential Equations Aims and scope Submit manuscript

Abstract

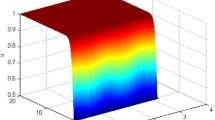
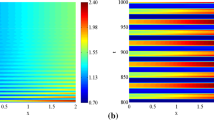
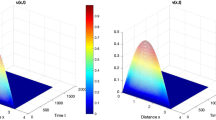
In this paper, we investigate a delayed reaction–diffusion–advection equation, which models the population dynamics in the advective heterogeneous environment. The existence of the nonconstant positive steady state and associated Hopf bifurcation are obtained. A weighted inner product associated with the advection rate is introduced to compute the normal forms, which is the main difference between Hopf bifurcation for delayed reaction–diffusion–advection model and that for delayed reaction–diffusion model. Moreover, we find that the spatial scale and advection can affect Hopf bifurcation in the heterogenous environment.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4

Similar content being viewed by others

References

  1. Belgacem, F., Cosner, C.: The effects of dispersal along environmental gradients on the dynamics of populations in heterogeneous environment. Can. Appl. Math. Q. 3(4), 379–397 (1995)

    MathSciNet  MATH  Google Scholar 

  2. Britton, N.F.: Spatial structures and periodic travelling waves in an integro-differential reaction–diffusion population model. SIAM J. Appl. Math. 50(6), 1663–1688 (1990)

    MathSciNet  MATH  Google Scholar 

  3. Busenberg, S., Huang, W.: Stability and Hopf bifurcation for a population delay model with diffusion effects. J. Differ. Equ. 124(1), 80–107 (1996)

    MathSciNet  MATH  Google Scholar 

  4. Cantrell, R.S., Cosner, C.: Spatial Ecology via Reaction–Diffusion Equations. Wiley, Chichester (2003)

    MATH  Google Scholar 

  5. Cantrell, R.S., Cosner, C., Hutson, V.: Ecological models, permanence, and spatial heterogeneity. Rocky Mt. J. Math. 26(1), 1–35 (1996)

    MathSciNet  MATH  Google Scholar 

  6. Cantrell, R.S., Cosner, C., Lou, Y.: Movement towards better environments and the evolution of rapid diffusion. Math. Biosci. 204, 199–214 (2006)

    MathSciNet  MATH  Google Scholar 

  7. Chen, S., Lou, Y., Wei, J.: Hopf bifurcation in a delayed reaction–diffusion–advection population model. J. Differ. Equ. 264(8), 5333–5359 (2018)

    MathSciNet  MATH  Google Scholar 

  8. Chen, S., Shi, J.: Stability and Hopf bifurcation in a diffusive logistic population model with nonlocal delay effect. J. Differ. Equ. 253(12), 3440–3470 (2012)

    MathSciNet  MATH  Google Scholar 

  9. Chen, S., Yu, J.: Stability and bifurcations in a nonlocal delayed reaction–diffusion population model. J. Differ. Equ. 260, 218–240 (2016)

    MathSciNet  MATH  Google Scholar 

  10. Chen, X., Hambrock, R., Lou, Y.: Evolution of conditional dispersal: a reaction–diffusion–advection model. J. Math. Biol. 57, 361–386 (2008)

    MathSciNet  MATH  Google Scholar 

  11. Cosner, C., Lou, Y.: Does movement toward better environments always benefit a population? J. Math. Appl. Anal. 277, 489–503 (2003)

    MathSciNet  MATH  Google Scholar 

  12. Crandall, M.G., Rabinowitz, P.H.: Bifurcation from simple eigenvalues. J. Funct. Anal. 8, 321–340 (1971)

    MathSciNet  MATH  Google Scholar 

  13. Du, Y., Hsu, S.-B.: On a nonlocal reaction-diffusion problem arising from the modeling of phytoplankton growth. SIAM J. Math. Anal. 42(3), 1305–1333 (2010)

    MathSciNet  MATH  Google Scholar 

  14. Faria, T.: Normal forms and Hopf bifurcation for partial differential equations with delays. Trans. Am. Math. Soc. 352(5), 2217–2238 (2000)

    MathSciNet  MATH  Google Scholar 

  15. Faria, T.: Normal forms for semilinear functional differential equations in Banach spaces and applications. II. Discrete Contin. Dyn. Syst. 7(1), 155–176 (2001)

    MathSciNet  MATH  Google Scholar 

  16. Faria, T.: Stability and bifurcation for a delayed predator-prey model and the effect of diffusion. J. Math. Anal. Appl. 254(2), 433–463 (2001)

    MathSciNet  MATH  Google Scholar 

  17. Faria, T., Huang, W.: Stability of periodic solutions arising from Hopf bifurcation for a reaction–diffusion equation with time delay. In: Differential Equations and Dynamical Systems (Lisbon, 2000), volume 31 of Fields Inst. Commun., pp. 125–141. Amer. Math. Soc. (2002)

  18. Faria, T., Huang, W., Wu, J.: Smoothness of center manifolds for maps and formal adjoints for semilinear FDEs in general Banach spaces. SIAM J. Math. Anal. 34(1), 173–203 (2002)

    MathSciNet  MATH  Google Scholar 

  19. Furter, J., Grinfeld, M.: Local vs. non-local interactions in population dynamics. J. Math. Biol. 27(1), 65–80 (1989)

    MathSciNet  MATH  Google Scholar 

  20. Gourley, S.A., So, J.W.-H., Wu, J.: Nonlocality of reaction–diffusion equations induced by delay: biological modeling and nonlinear dynamics. J. Math. Sci. 124(4), 5119–5153 (2004)

    MathSciNet  MATH  Google Scholar 

  21. Guo, S.: Stability and bifurcation in a reaction–diffusion model with nonlocal delay effect. J. Differ. Equ. 259(4), 1409–1448 (2015)

    MathSciNet  MATH  Google Scholar 

  22. Guo, S.: Spatio-temporal patterns in a diffusive model with non-local delay effect. IMA J. Appl. Math. 82(4), 864–908 (2017)

    MathSciNet  MATH  Google Scholar 

  23. Guo, S., Yan, S.: Hopf bifurcation in a diffusive Lotka–Volterra type system with nonlocal delay effect. J. Differ. Equ. 260(1), 781–817 (2016)

    MathSciNet  MATH  Google Scholar 

  24. Hadeler, K.P., Ruan, S.: Interaction of diffusion and delay. Discrete Contin. Dyn. Syst. Ser. B 8(1), 95–105 (2007)

    MathSciNet  MATH  Google Scholar 

  25. Hale, J.: Theory of Functional Differential Equations, 2nd edn. Springer-Verlag, New York (1977)

    MATH  Google Scholar 

  26. Hassard, B.D., Kazarinoff, N.D., Wan, Y.: Theory and Applications of Hopf Bifurcation. Cambridge University Press, Cambridge (1981)

    MATH  Google Scholar 

  27. Hu, G.-P., Li, W.-T.: Hopf bifurcation analysis for a delayed predator–prey system with diffusion effects. Nonlinear Anal. Real World Appl. 11(2), 819–826 (2010)

    MathSciNet  MATH  Google Scholar 

  28. Hu, R., Yuan, Y.: Spatially nonhomogeneous equilibrium in a reaction–diffusion system with distributed delay. J. Differ. Equ. 250(6), 2779–2806 (2011)

    MathSciNet  MATH  Google Scholar 

  29. Huisman, J., van Oostveen, P., Weissing, F.J.: Species dynamics in phytoplankton blooms: incomplete mixing and competition for light. Am. Nat. 154, 46–67 (1999)

    Google Scholar 

  30. Jin, Y., Hilker, F.M., Steffler, P.M., Lewis, M.A.: Seasonal invasion dynamics in a spatially heterogeneous river with fluctuating flows. Bull. Math. Biol. 76(7), 1522–1565 (2014)

    MathSciNet  MATH  Google Scholar 

  31. Lam, K.-Y.: Concentration phenomena of a semilinear elliptic equation with large advection in an ecological model. J. Differ. Equ. 250, 161–181 (2011)

    MathSciNet  MATH  Google Scholar 

  32. Lee, S.S., Gaffney, E.A., Monk, N.A.M.: The influence of gene expression time delays on Gierer–Meinhardt pattern formation systems. Bull. Math. Biol. 72(8), 2139–2160 (2010)

    MathSciNet  MATH  Google Scholar 

  33. Lou, Y., Lutscher, F.: Evolution of dispersal in open advective environments. J. Math. Biol. 69, 1319–1342 (2014)

    MathSciNet  MATH  Google Scholar 

  34. Lou, Y., Xiao, D., Zhou, P.: Qualitative analysis for a Lotka–Volterra competition system in advective homogeneous environment. Discrete Contin. Dyn. Syst. A 36, 953–969 (2016)

    MathSciNet  MATH  Google Scholar 

  35. Lou, Y., Zhao, X.-Q., Zhou, P.: Global dynamics of a Lotka–Volterra competition–diffusion–advection system in heterogeneous environments. J. Math. Pures Appl. 121, 47–82 (2019)

    MathSciNet  MATH  Google Scholar 

  36. Lou, Y., Zhou, P.: Evolution of dispersal in advective homogeneous environment: the effect of boundary conditions. J. Differ. Equ. 259, 141–171 (2015)

    MathSciNet  MATH  Google Scholar 

  37. Lutscher, F., Lewis, M.A., McCauley, E.: Effects of heterogeneity on spread and persistence in rivers. Bull. Math. Biol. 68, 2129–2160 (2006)

    MathSciNet  MATH  Google Scholar 

  38. Lutscher, F., McCauley, E., Lewis, M.A.: Spatial patterns and coexistence mechanisms in systems with unidirectional flow. Theor. Popul. Biol. 71, 267–277 (2007)

    MATH  Google Scholar 

  39. Mckenzie, H.W., Jin, Y., Jacobsen, J., Lewis, M.A.: \(R_0\) analysis of a spatiotemporal model for a stream population. SIAM J. Appl. Dyn. Syst. 11(2), 567–596 (2012)

    MathSciNet  MATH  Google Scholar 

  40. Sen, S., Ghosh, P., Riaz, S.S., Ray, D.S.: Time-delay-induced instabilities in reaction–diffusion systems. Phys. Rev. E 80(4), 046212 (2008)

    Google Scholar 

  41. Shi, Q., Shi, J., Song, Y.: Hopf bifurcation and pattern formation in a diffusive delayed logistic model with spatial heterogeneity. To appear in Discrete Contin. Dyn. Syst. B. (2018)

  42. Speirs, D.C., Gurney, W.S.C.: Population persistence in rivers and estuaries. Ecology 82(5), 1219–1237 (2001)

    Google Scholar 

  43. Su, Y., Wei, J., Shi, J.: Hopf bifurcations in a reaction–diffusion population model with delay effect. J. Differ. Equ. 247(4), 1156–1184 (2009)

    MathSciNet  MATH  Google Scholar 

  44. Su, Y., Wei, J., Shi, J.: Hopf bifurcation in a diffusive logistic equation with mixed delayed and instantaneous density dependence. J. Dyn. Differ. Equ. 24(4), 897–925 (2012)

    MathSciNet  MATH  Google Scholar 

  45. Vasilyeva, O., Lutscher, F.: Population dynamics in rivers: analysis of steady states. Can. Appl. Math. Q. 18, 439–469 (2011)

    MathSciNet  MATH  Google Scholar 

  46. Wu, J.: Theory and Applications of Partial Functional Differential Equations. Springer-Verlag, New York (1996)

    MATH  Google Scholar 

  47. Yan, X.-P., Li, W.-T.: Stability of bifurcating periodic solutions in a delayed reaction–diffusion population model. Nonlinearity 23(6), 1413–1431 (2010)

    MathSciNet  MATH  Google Scholar 

  48. Yan, X.-P., Li, W.-T.: Stability and Hopf bifurcations for a delayed diffusion system in population dynamics. Discrete Contin. Dyn. Syst. Ser. B 17(1), 367–399 (2012)

    MathSciNet  MATH  Google Scholar 

  49. Zhao, X.-Q., Zhou, P.: On a Lotka–Volterra competition model: the effects of advection and spatial variation. Calc. Var. 55, 73 (2016)

    MathSciNet  MATH  Google Scholar 

  50. Zhou, P.: On a Lotka–Volterra competition system: diffusion vs. advection. Calc. Var. 55, 137 (2016)

    MathSciNet  MATH  Google Scholar 

  51. Zhou, P., Xiao, D.: Global dynamics of a classical Lotka–Volterra competition–diffusion–advection system. J. Funct. Anal. 275(2), 356–380 (2018)

    MathSciNet  MATH  Google Scholar 

  52. Zhou, P., Zhao, X.-Q.: Evolution of passive movement in advective environments: general boundary condition. J. Differ. Equ. 264(6), 4176–4198 (2018)

    MathSciNet  MATH  Google Scholar 

Download references

Acknowledgements

The authors are grateful to the anonymous referees for their helpful comments and valuable suggestions which have improved the presentation of the paper.

Author information

Authors and Affiliations

  1. Department of Mathematics, Harbin Institute of Technology, Weihai, 264209, Shandong, People’s Republic of China

    Shanshan Chen, Junjie Wei & Xue Zhang

  2. School of Mathematics and Data, Foshan University, Foshan, 528000, Guangdong, People’s Republic of China

    Junjie Wei

Authors
  1. Shanshan Chen
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Junjie Wei
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Xue Zhang
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Junjie Wei.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

This research is supported by the National Natural Science Foundation of China (No 11771109).

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Chen, S., Wei, J. & Zhang, X. Bifurcation Analysis for a Delayed Diffusive Logistic Population Model in the Advective Heterogeneous Environment. J Dyn Diff Equat 32, 823–847 (2020). https://doi.org/10.1007/s10884-019-09739-0

Download citation

  • Received:

  • Revised:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10884-019-09739-0

Keywords

Search

Navigation