Rank orders and signed interactions in evolutionary biology
Abstract
Rank orders have been studied in evolutionary biology for almost a hundred years. Constraints on the order in which mutations accumulate are known from cancer drug treatment, and order constraints for species invasions are important in ecology. However, current theory on rank orders in biology is somewhat fragmented. Here, we show how our previous work on inferring genetic interactions from comparative fitness data (Crona et al., 2017) is related to an influential approach to rank orders based on sign epistasis. Our approach depends on order perturbations that indicate interactions. We apply our results to malaria parasites and find that order perturbations beyond sign epistasis are prevalent in the antimalarial drug-resistance landscape. This finding agrees with the observation that reversed evolution back to the ancestral type is difficult. Another application concerns the adaptation of bacteria to a methanol environment.
Introduction
Rank orders of genotypes with respect to fitness are central in evolutionary biology. Key concepts, such as peaks and mutational trajectories, refer to an underlying rank order. The impact of suboptimal peaks has been discussed ever since fitness landscapes were introduced (Wright, 1932). A major advantage with rank orders, as compared to other statistics for fitness landscape, is that order data is robust for variation in experimental methods and measurements. In fact, one does not necessarily need to measure fitness at all. If a rank order can be established by a competition experiment or by any type of rank order preserving fitness proxy, then one can apply theory on rank orders. Results from different empirical studies can therefore be compared; fitness or log-fitness, radius or area, change per minute or generation, makes no difference if rank orders are considered.
The concept of sign epistasis introduced by Weinreich et al. (2005), has been important for recent work on rank orders in evolutionary biology (Kaznatcheev, 2019; Crona et al., 2017; Wu et al., 2016; de Visser and Krug, 2014; Crona et al., 2013; Poelwijk et al., 2011). A system has sign epistasis if the sign of the effect of a mutation, whether positive or negative, depends on genetic background. The graphs in Figure 1 illustrate the absence of sign epistasis (A), sign epistasis (B), and reciprocal sign epistasis (C) for two-locus systems. Each arrow points toward the genotype of higher fitness. The importance of rank order data for two-locus subsystems is that they carry information about global aspects of fitness landscapes, and therefore about long-term evolution. For instance, all multi-peaked fitness landscapes have reciprocal sign epistasis (Poelwijk et al., 2011). Conversely, if subsystems of type C exist, but no systems of type B, then the landscape is multi-peaked (Crona et al., 2013).
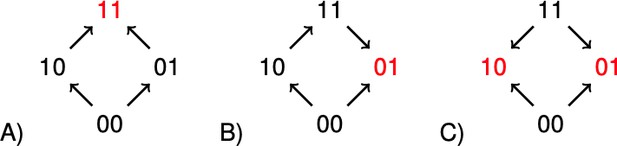
The graphs illustrate (A) no sign epistasis, (B) sign epistasis, and (C) reciprocal sign epistasis.
The peaks are marked red. Under the assumption that 00 has minimal fitness, and that the genotypes are positioned as in the figure, the three types can be characterized as graphs with no arrows down, one arrow down, or two arrows down.
The squares representing a two-locus subsystem (see the orange square in Figure 2) are clearly informative. However larger rectangles can improve the precision of the analysis. Each arrow in Figure 2 points toward the genotype of higher fitness. The undirected edges connect mutational neighbors, that is genotypes that differ at one locus only. The blue rectangles in Figure 2 concern replacements of the type for the second and third loci. If the sign of the effect of such a replacement depends on background, then the long arrows have different directions (Figure 2B and D). Such a perturbation is similar to sign epistasis, except that two loci are replaced.
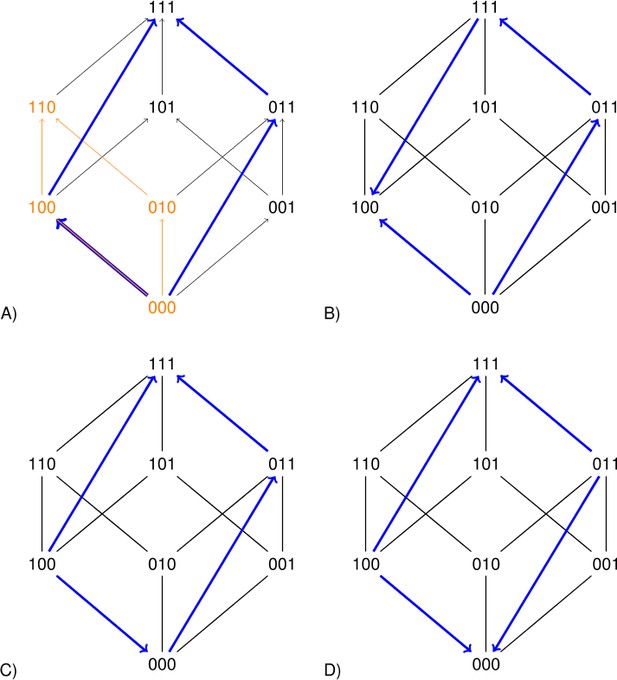
Each arrow points toward the genotype of higher fitness.
The undirected edges connect mutational neighbors and carry no information about fitness differences. The graph 2A is compatible with additive fitness. The other graphs are not compatible with additive fitness because at least one pair of parallel arrows point in different directions. The lower graphs indicate sign epistasis, as is clear from the short arrows. The right graphs indicate size two perturbations, as is clear from the long arrows.
We propose a broader perspective on perturbations, that takes advantage of all rectangles in the cube (or hypercube). Differently expressed, in addition to single mutations it is sometimes useful to consider the effects of double mutations and any higher order mutations, as well as replacements and for any selected subset of loci. If the sign of the effect of such a replacement depends on background, then the system has a rectangular perturbation. As proof of principle, we demonstrate that the large rectangles give new insights for two empirical studies (as compared to an analysis of sign epistasis). The first concerns antimalarial drug resistance (Ogbunugafor and Hartl, 2016), whereas the second example involves bacteria adapting to a methanol environment (Chou et al., 2011).
As in Crona et al. (2017), fitness is additive if the fitness effects of individual mutations sum, otherwise the system has epistasis (or gene interactions). By a rank order induced, or signed, interaction, we mean that the rank order reveals epistasis. Questions about how different approaches to signed interactions relate and how it is possible to determine whether a rank order is compatible with additive fitness were briefly discussed by the authors. As eLife reviewers pointed out, the signed interactions in Crona et al. (2017) are not generalizations of sign epistasis but there is clearly some connection. The remaining open problems were the starting point for this work. The main idea for resolving the problems was to consider rectangles such as those in Figure 2 (no knowledge of Walsh-coefficient, polytopes, or similarly is assumed here).
Results
We consider biallelic -locus systems. The fitness of a genotype is denoted . For simplicity, we assume that there exists a total order of the genotypes with respect to fitness, here referred to as a rank order (no two genotypes have equal fitness).
For two-locus systems, the expression measures epistasis. If it easy to verify that if fitness is additive. By definition, the system has an interaction if , and a signed interaction if the rank order implies that or . For instance the order implies that .
For a systematic treatment of order perturbations, it is necessary to write the information in compact form. Notice that a rank order where and implies sign epistasis, as the effect of a mutation at the first locus can increase or decrease fitness. Reciprocal sign epistasis for a two-locus system can be defined as a system with two peaks (Figure 1C). In particular, parallel arrows point in different directions.
It is straightforward to a check that a two-locus system has sign epistasis exactly if at least one of the following expressions is negative, and reciprocal sign epistasis if both of them are negative (Figure 1).
A negative sign corresponds to that a pair of parallel arrows that point in different directions. Large systems can be analyzed similarly.
A system has a rectangular perturbation if the sign of the effect of replacing a subset of loci, according to the rule and , depends on background. The size of the perturbation refers to the number of loci replaced.
In particular, a rectangular perturbation of size 1 is a case of sign epistasis. The expression was used to analyze gene interactions in the two-locus case, and a similar approach works in general. We define as the set of all expressions of the type
for genotypes such that if fitness is additive. For instance, for n = 3, the expressions
associated with two of the rectangles in Figure 2 (i.e., the leftmost side and the blue rectangle) are included in . Note that each expression in can be obtained by going around a rectangle in the cube and assigning coefficients with alternating signs along the way (each -coefficient is +1 or –1).
We call the elements in circuits, with reference to theory presented in Beerenwinkel et al. (2007b) (see also 'Materials and methods'; no knowledge of circuits is assumed). By a rank-order-induced circuit, or signed circuit, we mean that the rank order implies that the circuit is positive or negative (exactly as in the two-locus case).
For , consider the circuit and the related expressions (the four genotypes from the circuit can be combined in two different ways):
If the rank order implies that expression (3) is negative, then the system has sign epistasis, and if expression (4) is negative, the system has an order perturbation of size 2. The short blue arrows in Figure 2 point in different directions in the first case, and the long blue arrows do so in the latter. Similarly, any rectangular perturbation corresponds to two parallel arrows (along opposite sides of a rectangle) that point in different directions.
Remark 1. The relation between signed circuits and sign epistasis can be summarized as follows for .
A rank order of the genotypes 00, 10, 01 and 11 with respect to fitness implies that or exactly if the system has sign epistasis.
From the information that the rank order implies (or ) alone, one cannot determine whether there are one or two order perturbations, that is, whether or not the system has reciprocal sign epistasis.
The system has reciprocal sign epistasis if both expressions (1) and (2) are negative, and sign epistasis if at least one of them is negative.
Remark 2. A similar observation holds for signed circuits and any .
Each case of sign epistasis is associated with a signed interaction for a circuit in , that is the rank order of genotypes with respect to fitness implies that the form is positive or negative.
More generally, each rectangular perturbation is associated with a signed circuit interaction for a circuit in .
Each circuit in corresponds exactly to two potential rectangular perturbations.
In particular, in order to check a three-locus system for perturbations it is sufficient to check the signs of 24 expressions, including expression (3) and (4), associated with 12 circuits (see 'Materials and methods').
Applications
The first application concerns a study of the malaria-causing parasite Plasmodium vivax (Ogbunugafor and Hartl, 2016). The original study investigates a four-locus system exposed to different concentrations of the anti-malarial drug pyrimethamine (PYR). The quadruple mutant denoted 1111 has the highest degree of drug resistance, whereas the genotype 0000 has the highest fitness among all genotypes in the drug-free environment. Several concentrations of the drug were tested in the original study. We compared the highest concentration of the drug and the drug-free environment. Sign epistasis was equally frequent in both fitness landscapes. However, the drug-free environment had about twice as many perturbations of size 2 and 3, as the drug-exposed environment. The summary statistic indicates that (Table 1) adaptation is more difficult in the drug-free environment, because few replacements of pieces of code (regardless of size) are universally beneficial.
Rectangular perturbations for drug-exposed and drug-free malaria fitness landscapes.
The third line shows the total number of expressions checked. The prevalence of sign epistasis is similar for the landscapes, whereas the remaining perturbations differ by a factor of two.
| Perturbation size | 1 | 2 | 3 | Size (1-3) |
|---|---|---|---|---|
| Drug-exposed | 55 | 21 | 5 | 81 |
| Drug-free | 54 | 39 | 9 | 102 |
| Expressions checked | 112 | 72 | 16 | 200 |
This finding agrees well with the authors’ observation that resistance development is a relatively straightforward process, whereas reversed evolution from the mutant 1111 back to the ancestral type is difficult. Interestingly, the complete statistics of order perturbations was better able to distinguish the landscapes than sign epistasis alone.
The second application concerns a study of the bacterium Methylobacterium extorquens, which adapts to a methanol environment (Chou et al., 2011). For the sake of the argument, we assume that the published measurements are exact (a discussion about the impact of measurement errors can be found in 'Materials and methods'). The fitness landscape does not have sign epistasis. However, the system has size 2 perturbations (Table 2). In particular and , so that the sign of the effect of the change at the first pair of loci depends on background. The perturbations show that the rank order is incompatible with additive fitness.
Order perturbations of size 1 and 2 for Methylobacterium extorquens.
The landscape has no sign epistasis. However, perturbations of size 2 reveal that the landscape is not additive.
| Perturbation of size | 1 | 2 |
|---|---|---|
| Number of perturbations | 0 | 3 |
| Expressions checked | 112 | 72 |
A complete analysis of perturbations for an -locus system requires an investigation of expressions for (Theorem 1, 'Materials and methods'). Statistics on order perturbations should always be considered estimates because of measurement errors. However, that is true for all rank-order-based concepts, and a peak count is sensitive to noise. One could naively believe that a broader perspective makes the problem worse. By contrast, rectangular perturbations can sometimes be helpful in separating signal and noise.
For instance, assume that fitness is additive and that all mutations decrease fitness (Figure 3). The graphs show the proportion of unexpected observations, that is increased fitness, for replacements of the type , and for two error distributions (errors follow a normal distribution in the upper graph and the Student’s t-distribution with df = 3 in the lower, see 'Materials and methods' for more details). In both cases, the proportion of unexpected results decreases by the number of loci replaced. The reason is that the noise level is similar in all cases, whereas the effect size is not.

The fitness landscapes are additive and each mutation decreases fitness.
The graphs compare replacements and . Because of measurement errors, some replacements appear to increase fitness. The graphs show the proportion of such cases.
This observation can be useful. For instance, it is difficult to exclude additive fitness for a collection of detrimental mutations by observing sign epistasis alone (apparent sign epistasis can result from noise). However, an investigation of the three types of replacements described could be conclusive. In particular, similar proportions of unexpected results would constitute a strong argument against additive fitness.
Rank orders and perturbations are important for problems beyond natural selection. Constraints on the orders in which mutation occur are known for development of cancer drug resistance (Hosseini et al., 2019; Beerenwinkel et al., 2007a). In brief, a typical case would be that some mutation is not selected for unless a mutation has occurred, even though has high fitness. If the constraint holds universally, it can be described as: , where * is an arbitrary sequence. For an -locus system, such a constraint implies order perturbations if fitness is additive for the remaining loci.
Additivity and rank orders
The problem of determining if rank orders are compatible with additive fitness is already interesting for .
For the fitness landscape
the rank order is The change at any locus increases fitness regardless of background, so there is no sign epistasis. However, the sign of the effect of the change at the first pair of loci depends on background, as and . In particular, the rank order is not compatible with additive fitness.
As remarked in Crona et al. (2017), exactly 384 orders are compatible with the absence of sign epistasis for . By applying order perturbations (and by inspection), we verified that exactly 96 out of the 384 orders are compatible with additive fitness, or 0.24 percent of all 40,320 rank orders for three-locus systems. After relabeling (see 'Materials and methods' for details), only the following two orders are compatible with additive fitness:
Theory on rank orders and additivity has been developed independent of biological application and is still an active research area (Searles and Slinko, 2015; Maclagan, 1998). In principle, one can determine whether a specific rank order is compatible with additive fitness by solving a system of linear inequalities obtained from the order. It is straightforward to verify that a rank order is compatible with additive fitness unless it has rectangular perturbations for =4. However, counterexamples to the analogous statement for exist (Kraft et al., 1959) (see Materials and methods for more details).
Discussion
A rank order of genotypes, from highest to lowest fitness, is informative about gene interactions (Crona et al., 2017). We introduced rectangular perturbations to resolve problems on how different approaches to rank order induced (or signed) interactions relate. Sign epistasis concerns the effects of single mutations, and the new perturbations also concern the effects of multiple mutations.
We have established that sign epistasis implies that a system has signed circuit interactions, but the converse is not true. Strictly speaking, signed circuits are not generalizations of sign epistasis, neither are signed circuits refinements of sign epistasis. However, sign epistasis can be seen as rectangular perturbations of minimal size.
A rank order is compatible with additive fitness unless there is sign epistasis for a two-locus system. We have provided a counterexample for the analogous claim for three loci. For four-locus systems, a rank order is compatible with additive fitness unless there are rectangular perturbations. However, counterexamples exist for five loci (Maclagan, 1998; Kraft et al., 1959). In general, only a very small proportion of all rank orders are compatible with additive fitness, and the theoretical understanding for the property is limited.
As a proof of principle, we applied rectangular perturbations to empirical studies on antimalarial drug resistance (Ogbunugafor and Hartl, 2016) and on bacteria adapting to a methanol environment (Chou et al., 2011). Rectangular perturbations have the capacity to detect epistasis when conventional rank-order-based methods cannot. The perturbations capture evolutionary important properties beyond local aspects. A complete analysis of rectangular perturbations requires an investigation of a large number of expressions (of the order ). Often, it is meaningful to consider selected perturbations, depending on the context.
In general, rank orders are quite informative about gene interactions, and also regarding evolutionary potential (in a qualitative sense) provided one assumes the Strong Selection Weak Mutation regime (Gillespie, 1984). If the available information from an empirical study is a rank order, for instance from a competition experiment, then it is obviously useful to have methods for interpreting rank orders (further motivations are discussed in Crona et al., 2017). However, rank-orders methods have obvious limitations. Rank orders are insufficient for determining the effect of genetic recombination (de Visser et al., 2009; Otto and Lenormand, 2002). Evolutionary predictability is sensitive for population parameters, and the importance of accessible mutational trajectories is not universal (de Visser and Krug, 2014; Krug, 2019).
It would be interesting to determine the extent to which rectangular perturbations are helpful for relating local and global properties of fitness landscapes, similar to results on sign epistasis (Weinreich et al., 2005; Poelwijk et al., 2011; Crona et al., 2013), and also in analyzing statistical aspects further. Algorithms and exact formulas for potential perturbations are provided in the 'Materials and methods'.
Materials and methods
General circuits
Request a detailed protocolFor a biallelic -locus system, we have discussed a set defined as all expressions of the form
for genotypes , such that if fitness is additive. In order to connect to general theory, we need some concepts. Starting with the two-locus case, for the genotypes , one can form vectors in by adding an extra coordinate one for each genotype
The four vectors are linearly dependent since
The dependence relation corresponds exactly to the linear form
Similarly, one can form vectors in from the vertices of the -cube by adding a coordinate 1. Circuits are defined as the minimal dependence relations, in the sense that each proper subset of the vectors (with non-zero coefficients) are linearly independent.
There are in total 20 circuits for , including , but also for instance the circuit
In this terminology, one can describe as the set of circuits that have exactly four non-zero coefficients for the variables . Note that all circuits are zero if fitness is additive. In that sense, circuits measure epistasis. An analysis of all circuits provides very complete information on gene interactions (Beerenwinkel et al., 2007b).
Counting rectangular perturbation
Request a detailed protocolTo count rectangular perturbations, one needs to find all rectangles with vertices in an -cube. We provide an explicit formula with proof for the reader’s convenience, even though the result is elementary. The proof depends on Stirling numbers of the second kind. The Stirling number is defined as the number of ways to partition a set of objects into non-empty subsets. We refer to Grimaldi (2006) for more background.
Theorem 1. The total number of (potential) rectangular perturbations for an -locus system is
Moreover, the number of rectangular perturbations of size (exactly loci are replaced) equals
Corollary. A complete investigation of sign epistasis for an -locus system requires that one checks the signs of expressions.
Lemma 1. For Stirling numbers of the second kind , the following identities hold.
Proof. The first formula holds since there are non-empty proper subsets of elements, and each partition corresponds to exactly two subsets. Similarly, the second formula can be derived from the observation that one can construct three labeled subsets of elements in ways. After reducing for all cases with empty sets, the number of alternatives is
Each partition corresponds to six alternatives, which completes the argument.
Lemma 2. Let . There are
rectangles with vertices in an -cube.
Proof. For each vertex in an -cube, one can construct a rectangle with vertices on the -cube as follows. Distribute the set of loci into three subsets and , where the intersection of each pair of sets is empty, and where and are non-empty.
From the vertex , one constructs the remaining vertices by replacing sets of loci, according to the rule , and . Specifically, one vertex is obtained by replacing all elements in , one by replacing all elements in , and the last by replacing all elements in . If is empty, one can construct rectangles. If all three sets are non-empty, then one can choose in three ways, and consequently construct rectangles. In total, one obtains rectangles starting from a particular vertex. There are vertices in the -cube and each rectangle has four vertices. By the previous lemma, the number of rectangles is
which completes the proof.
We can now prove the main result.
Proof of Theorem 1. The total number of (potential) rectangular perturbations for an -locus system is
A rectangle with vertices in the -cube corresponds to exactly two rectangular perturbations, one for each pair of parallel edges. Consequently, the result follows from Lemma 2.
The second part of the theorem states that the number of rectangular perturbations where exactly loci are replaced is equal to
The positions of the loci that change can be chosen in different ways. There are words of length , and therefore pairs consisting of a word and its replacement (for instance, the replacement of 110 is 001). Finally, there are different backgrounds, so that a pair of backgrounds can be chosen in ways.
As mentioned, a single signed circuit may correspond to two cases of sign epistasis. Indeed, a two-locus system with reciprocal sign epistasis is an example. It is thus of interest to identify all order perturbations, rather than identifying signed circuits only. Theorem 1 and its proof indicate how one can find all order perturbations. In particular, the complete list for identifying rectangular perturbations for consists of the following 24 expressions, where the first 18 expressions concern sign epistasis, and the remaining six size 2 perturbations.
Rank orders compatible with additive fitness
Request a detailed protocolDifferent rank orders may differ only 'by labels’. For instance, the rank orders
differ by the map and , applied to both loci for each genotype. By definition, a cube isomorphism preserves the adjacency structure of the cube (a pair of mutational neighbors is mapped to a pair of neighbors).
Two rank orders are considered equivalent if a cube isomorphism induces a map between them. Differently expressed, for any given rank order (for a two-locus system), one can obtain an equivalent order by assigning the label 00 to a genotype of choice (among four alternatives) and then 10 to one of its neighbors (among two alternatives). After that, the adjacency condition determines the labels of the remaining genotypes. The new rank order is identical to the original one, except that the genotypes have new labels as described. It follows that each equivalence class consists of orders. The 24 rank orders for a two-locus systems can be partitioned into equivalence classes.
For general , each equivalence class consists of rank orders by a similar argument. In particular, for , each equivalence class consists of 48 rank orders, and the rank orders can be partitioned into 840 equivalence classes. Questions on rank orders for are manageable as it suffices to check 840 orders.
To determine whether a specific rank order is compatible with additive fitness, one has to solve a system of inequalities. For simplicity, we illustrate the argument for a two-locus system. Consider the order We can assume that
Additive fitness would imply , and then the rank order implies , which is a contradiction. It follows that the rank order is incompatible with additive fitness. In general, a rank order combined with an additive assumption determines a system of linear inequalities. The rank order is compatible with additive fitness exactly if the system has a solution. It is not difficult to find software for solving such a system of inequalities. In particular any software for solving linear programming problems can be used.
The case was discussed in the main text. For , one can verify computationally that there exist 14 rank orders (up to equivalence) with no order perturbations. As outlined, one can verify that all 14 rank orders are compatible with additive fitness. For , the analogous statement is not true, which was first shown by Kraft et al. (1959). An explicit counterexample is given in Maclagan (1998). This author studies Boolean term orders, in our terminology perturbation free rank orders, and refers to an order as being coherent if it is compatible with additive fitness. (The counterexample is described in slightly different notation, and the translation to our notation is
and analogously.)
As explained, in principle, one can check whether a given rank order is compatible with additive fitness. However, the theoretical understanding of rank orders and additive fitness is still limited.
Rank orders and statistical significance
Request a detailed protocolAs noted in the main text, rank-order methods are sensitive for measurement errors. A sufficient number of tests is necessary for reliable results, and elementary probability theory provides some guidance. If, for instance, genotype beats genotype in 9 out of 10 comparisons, then it is reasonable to reject a null hypothesis of equal fitness.
Research on inferring rank orders from pairwise comparisons has a long history because of applications to sports and games (Wauthier et al., 2013; Boyd and Silk, 1983; Albers and de Vries, 2001; Bradley and Terry, 1952; Thurstone, 1927). However, statistical significance for rank orders has received considerably less attention, and it would be interesting to develop more theory on the topic.
Simulations. The upper graph in Figure 3 was obtained by assuming additive fitness, a fitness decrease by 0.01 for each mutation, and errors sampled from a Gaussian distribution, where the magnitude of the errors is a value on the list 0.0010, 0.0011, 0.0012, …, 0.01. The assumptions were the same for the lower graph, except that a Student’s t-distribution with df = 3 was used.
Data availability
All data generated or analyzed during this study are included in the manuscript and supporting files.
References
-
Elo-rating as a tool in the sequential estimation of dominance strengthsAnimal Behaviour 61:489–495.https://doi.org/10.1006/anbe.2000.1571
-
A method for assigning cardinal dominance ranksAnimal Behaviour 31:45–58.https://doi.org/10.1016/S0003-3472(83)80172-9
-
The peaks and geometry of fitness landscapesJournal of Theoretical Biology 317:1–10.https://doi.org/10.1016/j.jtbi.2012.09.028
-
Exploring the effect of sex on empirical fitness landscapesThe American Naturalist 174:S15–S30.https://doi.org/10.1086/599081
-
Empirical fitness landscapes and the predictability of evolutionNature Reviews Genetics 15:480–490.https://doi.org/10.1038/nrg3744
-
Molecular evolution over the mutational landscapeEvolution 38:1116–1129.https://doi.org/10.2307/2408444
-
Estimating the predictability of Cancer evolutionBioinformatics 35:i389–i397.https://doi.org/10.1093/bioinformatics/btz332
-
Intuitive probability on finite setsThe Annals of Mathematical Statistics 30:408–419.https://doi.org/10.1214/aoms/1177706260
-
Resolving the paradox of sex and recombinationNature Reviews Genetics 3:252–261.https://doi.org/10.1038/nrg761
-
Reciprocal sign epistasis is a necessary condition for multi-peaked fitness landscapesJournal of Theoretical Biology 272:141–144.https://doi.org/10.1016/j.jtbi.2010.12.015
-
Noncoherent initial ideals in exterior algebrasBeiträge Zur Algebra Und Geometrie / Contributions to Algebra and Geometry 56:759–762.https://doi.org/10.1007/s13366-015-0239-5
-
The method of paired comparisons for social valuesThe Journal of Abnormal and Social Psychology 21:384–400.https://doi.org/10.1037/h0065439
-
ConferenceEfficient ranking from pairwise comparisonsInternational Conference on Machine Learning. pp. 109–117.
-
ConferenceThe roles of mutation, inbreeding, crossbreeding, and selection in evolutionProceedings of the Sixth International Congress of Genetics. pp. 356–366.
Article and author information
Author details
Funding
No external funding was received for this work
Acknowledgements
We are grateful to Casey Aguilar-Gervase, Tonia Bell, Payal Dudheida and David Dunleavy for their studies on rank orders of genotypes for four-locus systems, and to Ethan Christensen for his work on antimalarial drug resistance.
Version history
- Received: August 14, 2019
- Accepted: January 5, 2020
- Accepted Manuscript published: January 14, 2020 (version 1)
- Version of Record published: February 4, 2020 (version 2)
Copyright
© 2020, Crona
This article is distributed under the terms of the Creative Commons Attribution License, which permits unrestricted use and redistribution provided that the original author and source are credited.
Metrics
-
- 1,404
- views
-
- 147
- downloads
-
- 16
- citations
Views, downloads and citations are aggregated across all versions of this paper published by eLife.
Download links
Downloads (link to download the article as PDF)
Open citations (links to open the citations from this article in various online reference manager services)
Cite this article (links to download the citations from this article in formats compatible with various reference manager tools)
Further reading
-
- Evolutionary Biology
The RNA world hypothesis proposes that during the early evolution of life, primordial genomes of the first self-propagating evolutionary units existed in the form of RNA-like polymers. Autonomous, non-enzymatic, and sustained replication of such information carriers presents a problem, because product formation and hybridization between template and copy strands reduces replication speed. Kinetics of growth is then parabolic with the benefit of entailing competitive coexistence, thereby maintaining diversity. Here, we test the information-maintaining ability of parabolic growth in stochastic multispecies population models under the constraints of constant total population size and chemostat conditions. We find that large population sizes and small differences in the replication rates favor the stable coexistence of the vast majority of replicator species (‘genes’), while the error threshold problem is alleviated relative to exponential amplification. In addition, sequence properties (GC content) and the strength of resource competition mediated by the rate of resource inflow determine the number of coexisting variants, suggesting that fluctuations in building block availability favored repeated cycles of exploration and exploitation. Stochastic parabolic growth could thus have played a pivotal role in preserving viable sequences generated by random abiotic synthesis and providing diverse genetic raw material to the early evolution of functional ribozymes.
-
- Ecology
- Evolutionary Biology
Seasonal animal dormancy is widely interpreted as a physiological response for surviving energetic challenges during the harshest times of the year (the physiological constraint hypothesis). However, there are other mutually non-exclusive hypotheses to explain the timing of animal dormancy, that is, entry into and emergence from hibernation (i.e. dormancy phenology). Survival advantages of dormancy that have been proposed are reduced risks of predation and competition (the ‘life-history’ hypothesis), but comparative tests across animal species are few. Using the phylogenetic comparative method applied to more than 20 hibernating mammalian species, we found support for both hypotheses as explanations for the phenology of dormancy. In accordance with the life-history hypotheses, sex differences in hibernation emergence and immergence were favored by the sex difference in reproductive effort. In addition, physiological constraint may influence the trade-off between survival and reproduction such that low temperatures and precipitation, as well as smaller body mass, influence sex differences in phenology. We also compiled initial evidence that ectotherm dormancy may be (1) less temperature dependent than previously thought and (2) associated with trade-offs consistent with the life-history hypothesis. Thus, dormancy during non-life-threatening periods that are unfavorable for reproduction may be more widespread than previously thought.






