Skip to main content
SpringerLink
Log in

Approximation of Generating Function Barcode for Hamiltonian Diffeomorphisms

  • Published:
Foundations of Computational Mathematics Aims and scope Submit manuscript

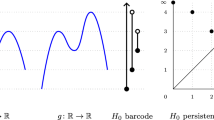
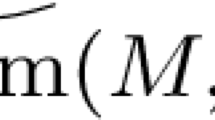Abstract

Persistence modules and barcodes are used in symplectic topology to define various invariants of Hamiltonian diffeomorphisms, however numerical methods for computing these barcodes are not yet well developed. In this paper we define one such invariant called the generating function barcode of compactly supported Hamiltonian diffeomorphisms of \( \mathbb {R}^{2n}\) by applying Morse theory to generating functions quadratic at infinity associated to such Hamiltonian diffeomorphisms and provide an algorithm (i.e a finite sequence of explicit calculation steps) that approximates it.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4

Similar content being viewed by others

References

  1. D. Alvarez-Gavela et al. “Embeddings of free groups into asymptotic cones of Hamiltonian diffeomorphisms”. In: Journal of Topology and Analysis 11.02, pp. 467-498, 2019.

  2. V. Arnold. Mathematical methods of classical mechanics. Springer, New York, 1989.

    Book  Google Scholar 

  3. U. Bauer and M. Lesnick. “Induced matchings and the algebraic stability of persistence barcodes”. In: Journal of Computational Geometry 6.2, pp. 162-191, 2015.

  4. U. Bauer et al. “Phat-persistent homology algorithms toolbox”. In: J. Symbolic Comput. 78 (2017), pp. 76-90.

    Article  MathSciNet  MATH  Google Scholar 

  5. M. Bialy and L. Polterovich. “Geodesics of Hofer’s metric on the group of Hamiltonian diffeomorphisms”. In: Duke Math. J. 76.1, pp. 273-292, 1994.

  6. M. Chaperon. “Une idée du type ”géodesiques brisées” pour les syst‘emes hamiltoniens. (A ”broken geodesic” type suggestion for Hamiltonian systems)”. French. In: C. R. Acad. Sci., Paris, Sér. I 298, pp. 293-296, 1984.

  7. M. Chaperon. “Phases génératrices en géométrie symplectique”. French. In: Les rencontres physiciensmathématiciens de Strasbourg -RCP25 41, 1990.

  8. H. Edelsbrunner and J. L. Harer. Computational topology: an introduction. American Mathematical Society, Providence, 2010.

    MATH  Google Scholar 

  9. A. Floer and H. Hofer. “Symplectic homology. I. Open sets in Cn”. In: Math. Z. 215.1, pp. 37-88, 1994.

  10. M. Fraser. “Contact spectral invariants and persistence”. In: Preprint arXiv:1502.05979, 2015.

  11. A. Hatcher. Algebraic topology. Cambridge University Press, Cambridge, 2002.

    MATH  Google Scholar 

  12. L. Hörmander. “Fourier integral operators. I”. In: Acta Math. 127.1-2, pp. 79-183, 1971.

  13. A. Kislev and E. Shelukhin. “Bounds on spectral norms and barcodes”. In: Geom. Topol. 25.7, pp. 3257-3350, 2021.

  14. F. Laudenbach and J.-C. Sikorav. “Persistance d’intersection avec la section nulle au cours d’une isotopie hamiltonienne dans un fibré cotangent”. In: Invent. Math. 82.2, pp. 349-357, 1985.

  15. D. McDuff and D. Salamon. Introduction to symplectic topology. Third. Oxford University Press, Oxford, 2017.

    Book  MATH  Google Scholar 

  16. J. Milnor. Morse theory. Princeton University Press, Princeton, 1963.

    Book  MATH  Google Scholar 

  17. L. Polterovich, E. Shelukhin, and V. Stojisavljević. “Persistence modules with operators in Morse and Floer theory”. In: Mosc. Math. J. 17.4, pp. 757-786, 2017.

  18. L. Polterovich and E. Shelukhin. “Autonomous Hamiltonian flows, Hofer’s geometry and persistence modules”. In: Selecta Mathematica 22.1, pp. 227-296, 2016.

  19. L. Polterovich et al. Topological persistence in geometry and analysis. American Mathematical Society, Providence, 2020.

    Book  MATH  Google Scholar 

  20. E. Shelukhin. “On the Hofer-Zehnder conjecture”. In: Preprint arXiv:1905.04769, 2019.

  21. J.-C. Sikorav. “Probl‘emes d’intersections et de points fixes en géométrie hamiltonienne”. In: Comment. Math. Helv. 62.1, pp. 62-73, 1987.

  22. B. Stevenson. “A quasi-isometric embedding into the group of Hamiltonian diffeomorphisms with Hofer’s metric”. In: Israel J. Math. 223.1, pp. 141-195, 2018.

  23. D. Théret. “Utilisation des fonctions generatrices en geometrie symplectique globale”. PhD thesis. Université Paris 7, France, 1996.

  24. D. Théret. “A complete proof of Viterbo’s uniqueness theorem on generating functions”. In: Topology Appl. 96.3, pp. 249-266, 1999.

  25. L. Traynor. “Symplectic homology via generating functions”. In: Geom. Funct. Anal. 4.6 , pp. 718-748, 1994.

  26. M. Usher and J. Zhang. “Persistent homology and Floer-Novikov theory”. In: Geom. Topol. 20.6, pp. 3333-3430, 2016.

  27. C. Viterbo. “Symplectic topology as the geometry of generating functions”. In: Math. Ann. 292.4, pp. 685-710, 1992.

  28. C. Viterbo. “An introduction to symplectic topology through sheaf theory”. In: Preprint 2011.

  29. J. Zhang. “p-cyclic persistent homology and Hofer distance”. In: J. Symplectic Geom. 17.3, pp. 857-927, 2019.

  30. A. Zomorodian and G. Carlsson. “Computing persistent homology”. In: Discrete Comput. Geom. 33.2, pp. 249-274, 2005.

Download references

Acknowledgements

This paper is a part of the second author’s thesis, carried out under the supervision of Prof. Leonid Polterovich and Prof. Lev Buhovsky at Tel-Aviv university. We thank them both for many meaningful discussions and for their original ideas motivating this project. The authors also wish to thank Prof. Yoel Shkolnisky from the applied math department of Tel-Aviv university for his kind assistance with computational issues. Finally, we thank the anonymous referee for their thorough review and many usefull comments and suggestions. The first author is partially supported by the European Research Council Grant No. 637386. The second author is supported by ISF Grant Numbers 1102/20 and 2026/17.

Author information

Authors and Affiliations

  1. School of Mathematical Sciences, Tel Aviv University, P.O. Box 39040, 6997801, Tel Aviv, Israel

    Pazit Haim-Kislev & Ofir Karin

Authors
  1. Pazit Haim-Kislev
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Ofir Karin
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Ofir Karin.

Additional information

Communicated by Shmuel Weinberger.

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Haim-Kislev, P., Karin, O. Approximation of Generating Function Barcode for Hamiltonian Diffeomorphisms. Found Comput Math (2023). https://doi.org/10.1007/s10208-023-09631-w

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s10208-023-09631-w

Keywords

Mathematics Subject Classification

Search

Navigation